Chapter 4.1, Problem 46PPS

(a)

To determine

To Calculate:

The cost of each CD for a member.

Answer to Problem 46PPS

Cost of each CD for a member = $5.25

Explanation of Solution

Given Information:

Let

y = Total cost in a year = $111.25

x = Number of CD purchased by a member in a year = 17

b = The membership cost per year = $22

Calculation:

First , we form the equation :

Let

y = Total cost in an year

x = Number of CD purchased by a member in an year

b = The membership cost per year

m = Cost of each CD

Now, we form the equation :

Cost of 1 CD = m

So, cost of x CD in an year = mx

The membership cost per year = b

So, the linear equation ( slope-intercept form) of the total cost y , when x CD purchased by a member in an year

  $y=mx+b\mathrm{..............................}(1)$

Now , we find m by substituting the values in (1)

  $\begin{array}{l}y=111.25\\ x=17\\ b=22\\ y=mx+b\mathrm{..........................................}[y=111.25,x=17,b=22]\\ \Rightarrow 111.25=m(17)+\mathrm{22......................}[substituting]\\ \Rightarrow 111.25-22=17m+22-\mathrm{22..........}[subtract"22"each\_side]\\ \Rightarrow 89.25=17m\mathrm{..................................}[simplify]\\ \Rightarrow \frac{89.25}{17}=\frac{17m}{17}\mathrm{.................................}[divide"17"each\_side]\\ \Rightarrow 5.25=m\\ OR\\ \Rightarrow m=5.25\end{array}$

Hence, the cost of each CD per member = $5.25.

(b)

To determine

To Calculate:

A linear equation to represent the total cost y of a one year membership, if x CDs are purchased .

Answer to Problem 46PPS

The equation that represents the total cost of a 1-year membership is :

  $y=5.25x+22$

Where,

y = Total cost in an year

x = Number of CD purchased by a member in an year

Explanation of Solution

Given Information:

Let

y = Total cost in a year = $111.25

x = Number of CD purchased by a member in a year = 17

b = The membership cost per year = $22

Calculation:

Let

y = Total cost in an year

x = Number of CD purchased by a member in an year

b = The membership cost per year

m = Cost of each CD

Now, we form the equation :

Cost of 1 CD = m

So, cost of x CD in an year = mx

The membership cost per year = b

So, the linear equation ( slope-intercept form) of the total cost y , when x CD purchased by a member in an year

  $y=mx+b\mathrm{..............................}(1)$

Substituting the values of m = 5.25 and b =22 in (1) , we get required equation:

  $y=5.25x+22$

Hence,

The equation that represents the total cost of a 1-year membership is :

  $y=5.25x+22$

Where,

y = Total cost in an year

x = Number of CD purchased by a member in an year

(c)

To determine

To Graph : The linear equation $y=5.25x+22$

Explanation of Solution

Given Information:

From part (a) and (b)

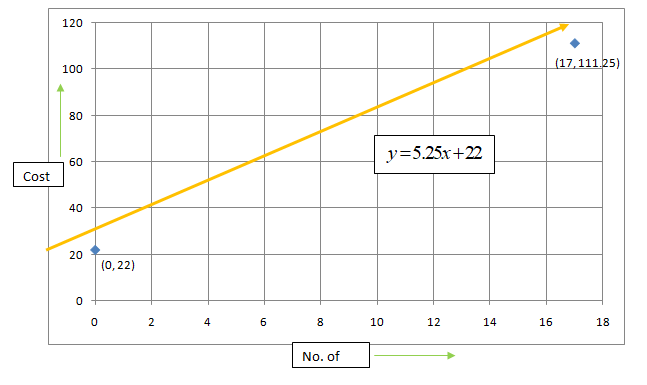
Equation of line : $y=5.25x+22$

And points on the line are (0,22) , (17,111.25)

Graph:

To graph the equation, plot the points (0,22) , (17,111.25) .

  

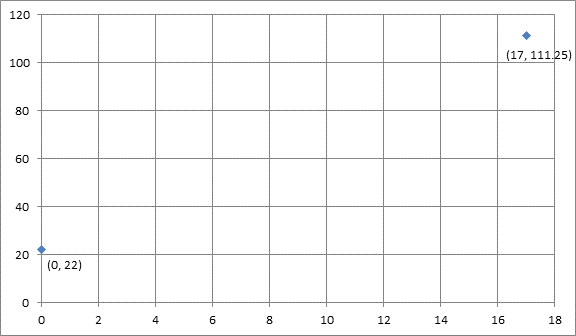
Now , draw a line to connect the points .