Chapter 4, Problem 87CE

Note: In each of the following tables, the upper bin limit is excluded from that bin but is included as the lower limit of the next bin.

A random sample of individuals who filed their own income taxes were asked how much time (hours) they spent preparing last year’s federal income tax forms. (a) Estimate the mean. (b) Estimate the standard deviation. (c) Do you think the observations would be distributed uniformly within each interval? Why would that matter? (d) Why do you imagine that unequal bin sizes (interval widths) were used?

To determine

Estimate the mean from the grouped data and frequencies.

Answer to Problem 87CE

The mean from the grouped data and frequencies is 9.458.

Explanation of Solution

Calculation:

The given information is about the random sample of individuals who filed their own taxes and time spent by them for the federal income tax forms during last year.

The mean for the grouped data is:

$\overline{x}=\frac{{\displaystyle \sum _{j=1}^k{f}_j{m}_j}}{n}$

Where,

$f_j$ is the frequency for j^th interval,

$m_j$ is the mid-point for j^th interval,

n is the total sample size $\left({\displaystyle \sum _{j=1}^k{f}_j}\right)$, when j lies between 1 and k.

If $x_l$ and $x_u$ are the lower and upper limits of the j^th interval, then $m_j=\frac{x_l+x_u}{2}$

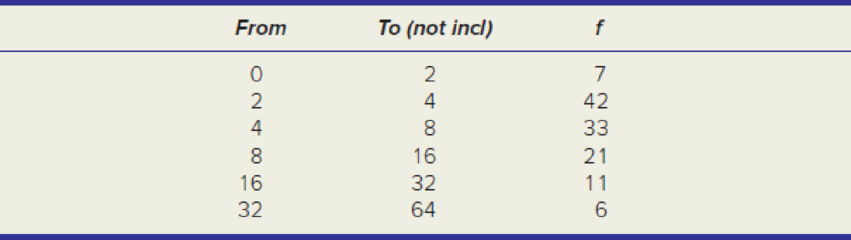
The table below gives the mean for the grouped data:

From $x_l$	To $x_u$	Frequency $f_j$	Mid-point $m_j=\frac{x_l+x_u}{2}$	$f_j\times m_j$
0	2	7	$\frac{0+2}{2}=1$	7
2	4	42	$\frac{2+4}{2}=3$	126
4	8	33	$\frac{4+8}{2}=6$	198
8	16	21	$\frac{8+16}{2}=12$	252
16	32	11	$\frac{16+32}{2}=24$	264
32	64	6	$\frac{32+64}{2}=48$	288
		${\displaystyle \sum f_j}=120$		${\displaystyle \sum _{j=1}^6{f}_j{m}_j}=1,135$

The mean for the grouped data is:

Substitute the values ${\displaystyle \sum f_j}=120$ and ${\displaystyle \sum _{j=1}^6{f}_j{m}_j}=1,135$ to get the mean,

$\begin{array}{c}\overline{x}=\frac{1,135}{120}\\ =9.458\end{array}$

Thus, the mean from the grouped data and frequencies is 9.458.

To determine

Estimate the standard deviation from the grouped data and frequencies.

Answer to Problem 87CE

The standard deviation from the grouped data and frequencies is 10.855.

Explanation of Solution

Calculation:

The standard deviation for the grouped data is:

$s=\sqrt{{\displaystyle \sum _{j=1}^k\frac{f_j\left(m_j-\overline{x}\right)}{n-1}}}$

Where,

$f_j$ is the frequency for j^th interval,

$m_j$ is the mid-point for j^th interval,

n is the total sample size $\left({\displaystyle \sum _{j=1}^k{f}_j}\right)$, when j lies between 1 and k.

If $x_l$ and $x_u$ are the lower and upper limits of the j^th interval, then $m_j=\frac{x_l+x_u}{2}$

The table below gives the mean for the grouped data:

From $x_l$	To $x_u$	Frequency $f_j$	Mid-point $m_j=\frac{x_l+x_u}{2}$	${\left(m_j-\overline{x}\right)}^2$	$f_j{\left(m_j-\overline{x}\right)}^2$
0	2	7	$\frac{0+2}{2}=1$	${\left(1-9.458\right)}^2=71.54$	500.78
2	4	42	$\frac{2+4}{2}=3$	${\left(3-9.458\right)}^2=41.71$	1,751.642
4	8	33	$\frac{4+8}{2}=6$	${\left(6-9.458\right)}^2=11.96$	394.606
8	16	21	$\frac{8+16}{2}=12$	${\left(12-9.458\right)}^2=6.462$	135.697
16	32	11	$\frac{16+32}{2}=24$	${\left(24-9.458\right)}^2=211.47$	2,326.17
32	64	6	$\frac{32+64}{2}=48$	${\left(48-9.458\right)}^2=1,485.49$	8,912.915
		${\displaystyle \sum f_j}=120$			$\begin{array}{l}{\displaystyle \sum _{j=1}^6{f}_j{\left(m_j-\overline{x}\right)}^2}\\ =14,021.81\end{array}$

The standard deviation for the grouped data is:

Substitute the values ${\displaystyle \sum f_j}=120$ and ${\displaystyle \sum _{j=1}^6{f}_j{\left(m_j-\overline{x}\right)}^2}=14,021.81$ to get the standard deviation,

$\begin{array}{c}s=\sqrt{\frac{14,021.81}{120-1}}\\ =\sqrt{\frac{14,021.81}{119}}\\ =\sqrt{117.83}\\ =10.855\end{array}$

Thus, the standard deviation from the grouped data and frequencies is 10.855.

To determine

Decide whether the observations are distributed uniformly within each interval.

Answer to Problem 87CE

No, the observations are distributed uniformly within each interval.

Explanation of Solution

A careful inspection on the observation states that the lower frequency ranges are much greater than that of the higher ranges. Thus, it is clear that the normal curve will be skewed right. Most of the data values seemed to appear closer to the lower frequency range. The difference made in the estimate of the mean was caused due to the changes made in the midpoint. If the midpoint has equal weight than the estimate of mean would appear much low.

To determine

Explain the reason for thinking that the bins used are of unequal sizes (interval width).

Explanation of Solution

The equal sizes of the bins in a histogram may not always allow the shape of the data to be represented accurately. Moreover, ensuring equal bins may produce one or more empty classes. This makes the histogram more difficult to interpret and is not desirable.

In this case, equal bins would have given rise to empty classes.

Hence, the bins used are of unequal sizes.