Chapter 4, Problem 197RE

a.

To determine

To graph using graphing utility the arctangent function and its polynomial approximation.

Explanation of Solution

Given information:

Use graphing device to graph the function

  $\begin{array}{l}\arctan x\approx x-\frac{x^3}{3}+\frac{x^5}{5}-\frac{x^7}{7}\\ \text{where }x\text{ is in radians}\text{.}\end{array}$

Graph:

Sketch the graph using graphing utility.

Step 1: Press WINDOW button to access the Window editor.

Step 2: Press $\boxed{\text{Y=}}$ button.

Step 3: Enter the expression $x^4-4x^3+2x^2+4x-3$ which is required to graph.

Step 4: Press GRAPH button to graph the function and adjust the windows according to the graph.

The graph is obtained as:

  

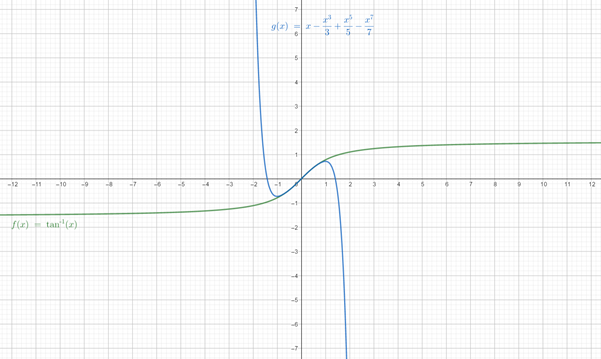
Interpretation:

From the above graph, it can be observed that the

Domain of $\arctan x$ : $\text{R}$ (all real numbers)

Range of $\arctan x$ : $\left(-\frac{\pi }{2},\frac{\pi }{2}\right)$

Domain of $x-\frac{x^3}{3}+\frac{x^5}{5}-\frac{x^7}{7}$ : $\text{R}$ (all real numbers)

Range of $x-\frac{x^3}{3}+\frac{x^5}{5}-\frac{x^7}{7}$ : $\text{R}$ (all real numbers)

As $x$ approaches to $0$ , value of both $\arctan x$ and $x-\frac{x^3}{3}+\frac{x^5}{5}-\frac{x^7}{7}$ approaches to $0$ .

b.

To determine

To predict the next term of polynomial approximation of the $\arctan x$ function .

Answer to Problem 197RE

The exact value of ${\tan }^{-1}\left(-\sqrt{3}\right)$ is $-\frac{\pi }{3}$

Explanation of Solution

Given:

The function and its polynomial approximation is:

  $\begin{array}{l}\arctan x\approx x-\frac{x^3}{3}+\frac{x^5}{5}-\frac{x^7}{7}\\ \text{where }x\text{ is in radians}\text{.}\end{array}$

Calculation:

The polynomial approximation is $x-\frac{x^3}{3}+\frac{x^5}{5}-\frac{x^7}{7}$

On observing the pattern, it can be observed that the polynomial contains alternate positive and negative signs along with odd power of x with odd numbers in denominator with common difference $2$ .

Therefore, the next term is $\frac{x^9}{9}$

The polynomial is : $x-\frac{x^3}{3}+\frac{x^5}{5}-\frac{x^7}{7}+\frac{x^9}{9}$

Graph:

  

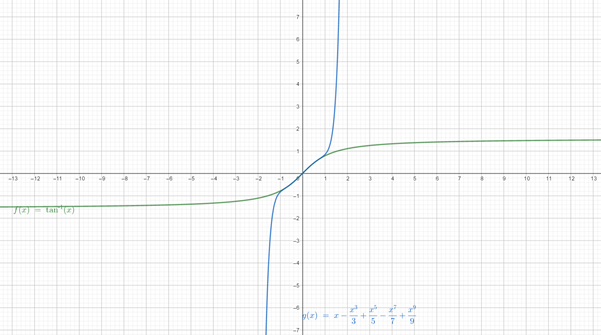
Interpretation:

From the above graph, it can be observed that the as the next term is added the graph become more accurate.