
Logistic growth Scientists often use the logistic growth function
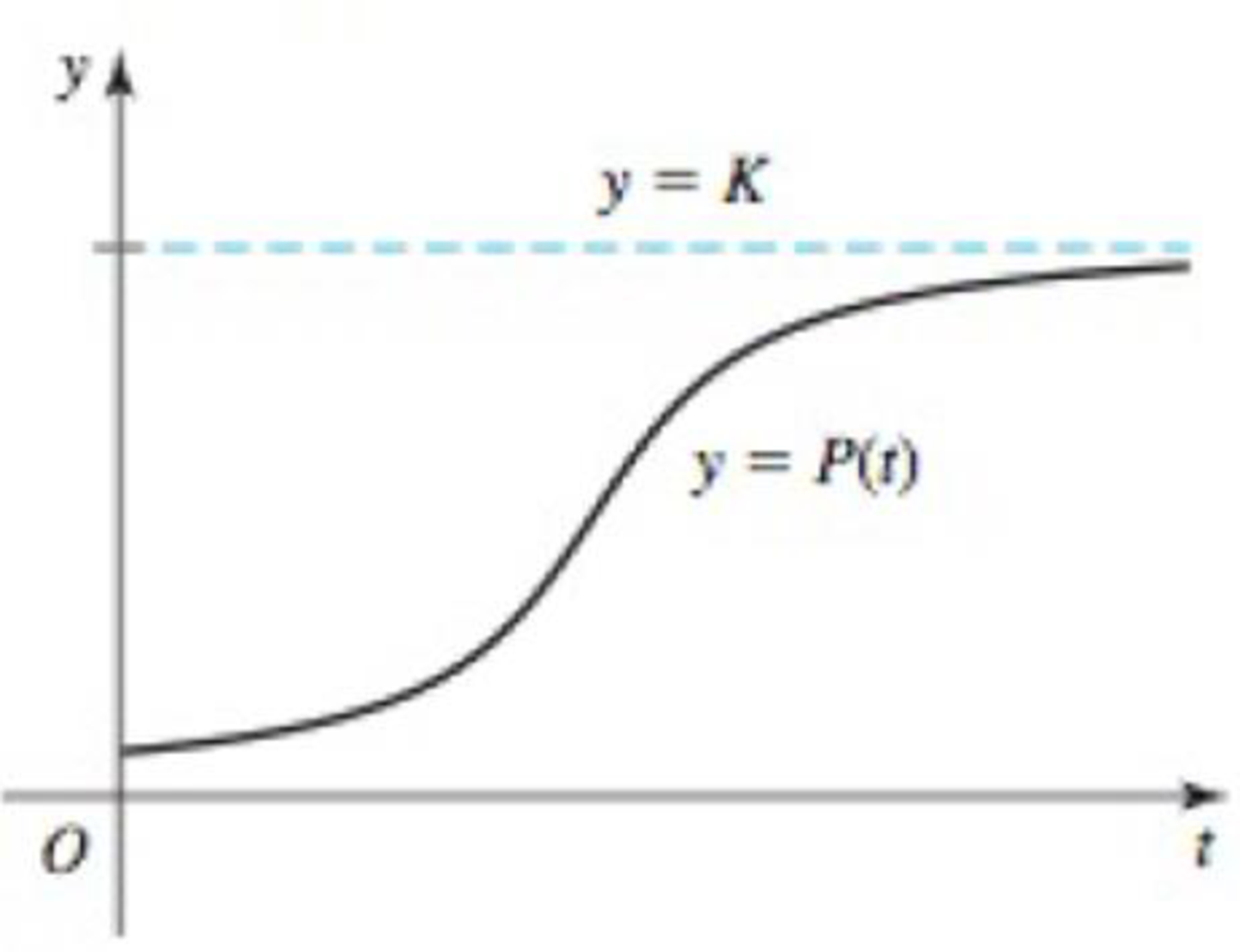
96. Population crash The logistic model can be used for situations in which the initial population P0 is above the carrying capacity K. For example, consider a deer population of 1500 on an island where a fire has reduced the carrying capacity to 1000 deer.
- a. Assuming a base growth rate of r0 = 0.1 and an initial population of P(0) = 1500, write a logistic growth function for the deer population and graph it. Based on the graph, what happens to the deer population in the long run?
- b. How fast (in deer per year) is the population declining immediately after the fire at t = 0?
- c. How long does it take the deer population to decline to 1200 deer?
Want to see the full answer?
Check out a sample textbook solution
Chapter 3 Solutions
Single Variable Calculus: Early Transcendentals (2nd Edition) - Standalone book
Additional Math Textbook Solutions
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
Elementary Statistics: Picturing the World (7th Edition)
A First Course in Probability (10th Edition)
Intro Stats, Books a la Carte Edition (5th Edition)
Precalculus
Basic Business Statistics, Student Value Edition
- 2. f(x)=√7-x 4. A manufacturer has a monthly fixed cost of $40,000 and a production cost of $8 for each unit produced. The product sells for $12 per unit. 1. What is the cost function? 2. What is the revenue function? 3. Compute the profit corresponding to 12,000 units. 5. A rectangular box is to have a square base and a volume of 20 ft3. The material for the base costs $0.30 per ft2, the material for the sides cost $0.10 per ft2, and the material for the top costs $0.20 per ft2. Letting x denote the length of one side of the base,arrow_forwardSolve using superposition principlearrow_forwardreview problems please help!arrow_forward
- 3. f(7) 3. Find the domain of each of the following functions. 1 1. f(x)=2-6x+8 2. f(x)=√√7-x 4. A manufacturer has a monthly fixed cost of $40,000 and a production cost of $8 for each unit produced. The product sells for $12 per unit.arrow_forward7. Evaluate the following limits and justify each step. (a) lim (3x²+2x+1) 1 x²+4x-12 (b) lim 1 2 x² - 2x t-√√3t+4 (c) lim t-0 4-t x²-6x+5 (d) lim (e) lim x 5 x-5 x→2 x²+2x+3 4u+1-3 (f) lim u➡2 u-2 1 (g) lim x-3 2 x 55 x - 7x4 +4 (h) lim xx 5x+2x-1 x+1 (i) lim x²-2x+5 - 7x8+4x7 +5xarrow_forward6. Given the following graph f(x). (-2,2) 2- -5 -3 -2 (-2,-1) -1 (0,1) -2- 1 (3,0) 2 3 4 5 (3,-1) א X Compute each of the following. (a) f(-2) (b) lim f(x) #129 (c) lim f(x) *→12+ (d) lim f(x) 811H (e) f(0) (f) lim f(x) 8011 (m) Is the function continuous at x = -2,0,3? Why or why not? (g) lim f(x) +0x (h) lim f(x) x 0 (i) f(3) (j) lim f(x) x-3- (k) lim f(x) x+3+ (1) lim f(x) #13arrow_forward
- 3. Compute the profit corresponding to 12,000 units. 5. A rectangular box is to have a square base and a volume of 20 ft3. The material for the base costs $0.30 per ft2, the material for the sides cost $0.10 per ft2, and the material for the top costs $0.20 per ft2. Letting a denote the length of one side of the base, find a function in the variable x giving the cost of constructing the box. 6. Given the following graph f(x).arrow_forward8. On what intervals, each function continuous? (a) f(x) = 3x11 + 4x²+1 3x²+5x-1 (b) g(x) = x²-4 X, x < 1, QTs the function f(x) continuous at = 1? Use the definition of continuity to justifyarrow_forwardreview problem please help!arrow_forward
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage


 Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning





