
Concept explainers
In Figure P35.30, a thin converging lens of focal length 14.0 cm forms an image of the square abed, which is he = hb = 10.0 cm high and lies between distances of pd = 20.0 cm and pa = 30.0 cm from the lens. Let a′, b′, c′. and d′ represent the respective corners of the image. Let qa represent the image distance for points a′ and b′, qd represent the image distance for points c′ and d′,
where h′ and q are in centimeters. (d) Explain why the geometric area of the image is given by
(e) Carry out the integration to find the area of the image.
Figure P35.30
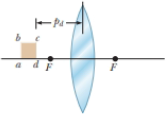
(a)
Answer to Problem 36.50P
Explanation of Solution
Given info: The focal length of the lens is
Formula to calculate the image of any object in a thin lens is,
Here,
Substitute
Here,
Substitute
Substitute
Here,
Substitute,
Formula to calculate the height of the corresponding image point

Here,
Substitute
For calculating the image height corresponding to
Substitute
Conclusion:
Therefore, the values of
(b)
To draw: The sketch of the ray diagram.
Answer to Problem 36.50P
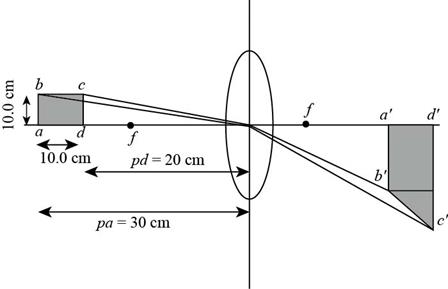
Explanation of Solution
The image of the square
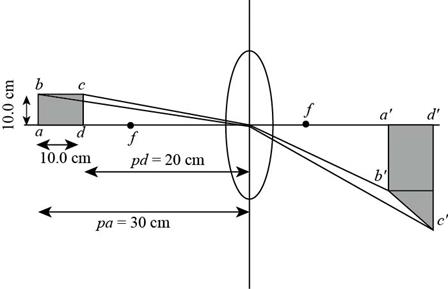
Figure (1)
(c)
To show: The relation,
Answer to Problem 36.50P
Explanation of Solution
Here,
From the lens,
Here,
Substitute
Substitute
Conclusion:
Therefore the relation between height of the image and the image distance is
(d)
To write: The explanation that the geometric area of image is
Answer to Problem 36.50P
Explanation of Solution
Given info: The geometric area of a image is,
Here,
From equation (10) the integral sums up the small areas of region covered by the image itself. The height of the small regions is
Therefore area of that small region is
Therefore the integration from
Conclusion:
Therefore, the geometric area of the image is given by integral
(e)
Answer to Problem 36.50P
Explanation of Solution
Given info: The geometric area of the image is given by the integral,
From equation (9) substitute
Integrate the above equation with respect to
Conclusion:
Therefore, the geometric area of the image is
Want to see more full solutions like this?
Chapter 36 Solutions
Physics for Scientists and Engineers, Volume 1, Chapters 1-22
- In Figure P26.38, a thin converging lens of focal length 14.0 cm forms an image of the square abcd, which is hc = hb = 10.0 cm high and lies between distances of pd = 20.0 cm and pa = 30.0 cm from the lens. Let a, b, c, and d represent the respective corners of the image. Let qa represent the image distance for points a and b, qd represent the image distance for points c and d, hb represent the distance from point b to the axis, and hc represent the height of c. (a) Find qa, qd, hb, and hc. (b) Make a sketch of the image. (c) The area of the object is 100 cm2. By carrying out the following steps, you will evaluate the area of the image. Let q represent the image distance of any point between a and d, for which the object distance is p. Let h represent the distance from the axis to the point at the edge of the image between b and c at image distance q. Demonstrate that h=10.0q(114.01q) where h and q are in centimeters. (d) Explain why the geometric area of the image is given by qaqdhdq (e) Carry out the integration to find the area of the image. Figure P26.38arrow_forwardTwo converging lenses having focal lengths of f1 = 10.0 cm and f2 = 20.0 cm are placed a distance d = 50.0 cm apart as shown in Figure P35.48. The image due to light passing through both lenses is to be located between the lenses at the position x = 31.0 cm indicated. (a) At what value of p should the object be positioned to the left of the first lens? (b) What is the magnification of the final image? (c) Is the final image upright or inverted? (d) Is the final image real or virtual?arrow_forwardA converging lens made of crown glass has a focal length of 15.0 cm when used in air. If the lens is immersed in water, what is its focal length? (a) negative (b) less than 15.0 cm (c) equal to 15.0 cm (d) greater than 15.0 cm (e) none of those answersarrow_forward
- Why is the following situation impossible? Consider the lensmirror combination shown in Figure P35.55. The lens has a focal length of fL = 0.200 m, and the mirror has a focal length of fM = 0.500 m. The lens and mirror are placed a distance d = 1.30 m apart, and an object is placed at p = 0.300 m from the lens. By moving a screen to various positions to the left of the lens, a student finds two different positions of the screen that produce a sharp image of the object. One of these positions corresponds to light leaving the object and traveling to the left through the lens. The other position corresponds to light traveling to the right from the object, reflecting from the mirror and then passing through the lens. Figure P35.55 Problem 55 and 57.arrow_forwardA lamp of height S cm is placed 40 cm in front of a converging lens of focal length 20 cm. There is a plane mirror 15 cm behind the lens. Where would you find the image when you look in the mirror?arrow_forwardThe left face of a biconvex lens has a radius of curvature of magnitude 12.0 cm, and the right face has a radius of curvature of magnitude 18.0 cm. The index of refraction of the glass is 1.44. (a) Calculate the focal length of the lens for light incident from the left. (b) What If? After the lens is turned around to interchange the radii of curvature of the two faces, calculate the focal length of the lens for light incident from the left.arrow_forward
- Figure P38.43 shows a concave meniscus lens. If |r1| = 8.50 cm and |r2| = 6.50 cm, find the focal length and determine whether the lens is converging or diverging. The lens is made of glass with index of refraction n = 1.55. CHECK and THINK: How do your answers change if the object is placed on the right side of the lens? FIGURE P38.43arrow_forwardWhat is the magnification of a magnifying lens with a focal length of 10 cm if it is held 3.0 cm from the eye and the object is 12 cm from the eye?arrow_forwardA convex mirror with a radius of curvature of 25.0 cm is used to form an image of an arrow that is 10.0 cm away from the mirror. If the arrow is 2.00 cm tall and inverted (pointing below the optical axis), what is the height of the arrows image?arrow_forward
- An amoeba is 0.305 cm away from the 0.300 cm- focal length objective lens of a microscope. (a) Where is the image formed by the objective lens? (b) What is this image’s magnification? (C) An eyepiece with a 2.00-cm focal length is placed 20.0 cm from the objective. Where is the final image? (d) What angular magnification is produced by the eyepiece? (e) What is the overall magnification? (See Figure 2.39.)arrow_forwardYou view an object by holding a 2.5 cm-focal length magnifying glass 10 cm away from it. How far from your eye should you hold the magnifying glass to obtain a magnification of 10 ?arrow_forwardA doctor examines a mole with a 15.0-cm focal length magnifying glass held 13.5 cm from the mole. (a) Where is the image? (b) What is its magnification? (c) How big is the image of a 5.00 mm diameter mole?arrow_forward
 Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning





