
Concept explainers
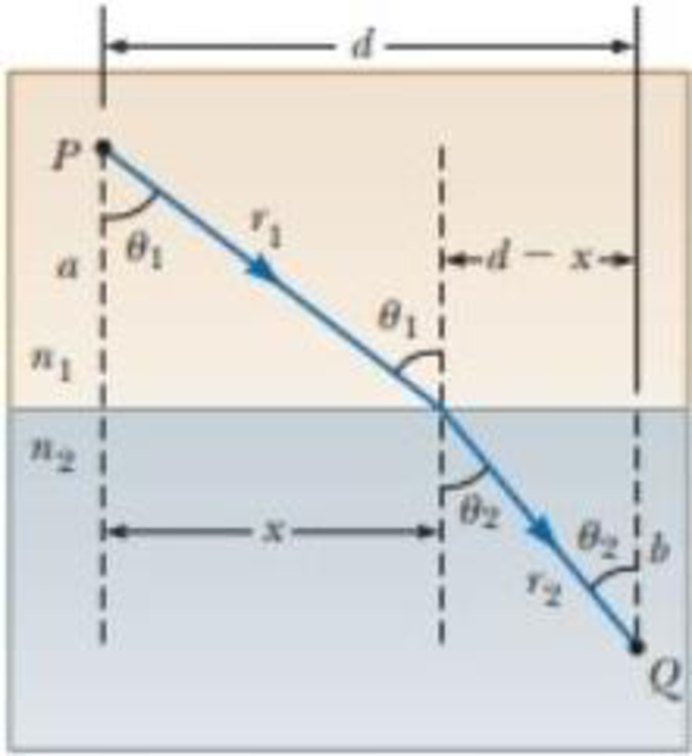
Pierre de Fermat (1601–1665) showed that whenever light travels from one point to another, its actual path is the path that requires the smallest time interval. This statement is known as Fermat’s principle. The simplest example is for light propagating in a homogeneous medium. It moves in a straight line because a straight line is the shortest distance between two points. Derive Snell’s law of refraction from Fermat’s principle. Proceed as follows. In Figure P34.54, a light ray travels from point P in medium 1 to point Q in medium 2. The two points are, respectively, at perpendicular distances a and b from the interface. The displacement from P to Q has the component d parallel to the interface, and we let x represent the coordinate of the point where the ray enters the second medium. Let t = 0 be the instant the light starts from P. (a) Show that the time at which the light arrives at Q is
(b) To obtain the value of x for which t has its minimum value, differentiate t with respect to x and set the derivative equal to zero. Show that the result implies
(c) Show that this expression in turn gives Snell’s law.
Figure P34.54 Problems 54 and 55.
Want to see the full answer?
Check out a sample textbook solution
Chapter 34 Solutions
Bundle: Physics For Scientists And Engineers With Modern Physics, Loose-leaf Version, 10th + Webassign Printed Access Card For Serway/jewett's Physics For Scientists And Engineers, 10th, Single-term
- 1. A long silver rod of radius 3.5 cm has a charge of -3.9 ис on its surface. Here ŕ is a unit vector ст directed perpendicularly away from the axis of the rod as shown in the figure. (a) Find the electric field at a point 5 cm from the center of the rod (an outside point). E = N C (b) Find the electric field at a point 1.8 cm from the center of the rod (an inside point) E=0 Think & Prepare N C 1. Is there a symmetry in the charge distribution? What kind of symmetry? 2. The problem gives the charge per unit length 1. How do you figure out the surface charge density σ from a?arrow_forward1. Determine the electric flux through each surface whose cross-section is shown below. 55 S₂ -29 S5 SA S3 + 9 Enter your answer in terms of q and ε Φ (a) s₁ (b) s₂ = -29 (C) Φ զ Ερ (d) SA = (e) $5 (f) Sa $6 = II ✓ -29 S6 +39arrow_forwardNo chatgpt pls will upvotearrow_forward
- the cable may break and cause severe injury. cable is more likely to break as compared to the [1] ds, inclined at angles of 30° and 50° to the vertical rings by way of a scaled diagram. [4] I 30° T₁ 3cm 3.8T2 cm 200 N 50° at it is headed due North and its airspeed indicat 240 km/h. If there is a wind of 100 km/h from We e relative to the Earth? [3]arrow_forwardCan you explain this using nodal analysis With the nodes I have present And then show me how many KCL equations I need to write, I’m thinking 2 since we have 2 dependent sourcesarrow_forwardstate the difference between vector and scalar quarrow_forward
- Please don't use Chatgpt will upvote and give handwritten solutionarrow_forwardNo chatgpt pls will upvotearrow_forwardThe shear leg derrick is used to haul the 200-kg net of fish onto the dock as shown in. Assume the force in each leg acts along its axis. 5.6 m. 4 m- B Part A Determine the compressive force along leg AB. Express your answer to three significant figures and include the appropriate units. FAB = Value Submit Request Answer Part B Units ? Determine the compressive force along leg CB. Express your answer to three significant figures and include the appropriate units. FCB= Value Submit Request Answer Part C ? Units Determine the tension in the winch cable DB. Express your answer with the appropriate units. 2marrow_forward
- Part A (Figure 1) shows a bucket suspended from a cable by means of a small pulley at C. If the bucket and its contents have a mass of 10 kg, determine the location of the pulley for equilibrium. The cable is 6 m long. Express your answer to three significant figures and include the appropriate units. Figure 4 m B НА x = Value Submit Request Answer Provide Feedback < 1 of 1 T 1 m Units ?arrow_forwardThe particle in is in equilibrium and F4 = 165 lb. Part A Determine the magnitude of F1. Express your answer in pounds to three significant figures. ΑΣΦ tvec F₁ = Submit Request Answer Part B Determine the magnitude of F2. Express your answer in pounds to three significant figures. ΑΣΦ It vec F2 = Submit Request Answer Part C Determine the magnitude of F3. Express your answer in pounds to three significant figures. ? ? lb lb F₂ 225 lb 135° 45° 30° -60°-arrow_forwardThe 10-lb weight is supported by the cord AC and roller and by the spring that has a stiffness of k = 10 lb/in. and an unstretched length of 12 in. as shown in. Part A Determine the distance d to maintain equilibrium. Express your answer in inches to three significant figures. 節 ΕΠΙ ΑΣΦ d = *k J vec 5 t 0 ? d C A in. 12 in. Barrow_forward
 Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning





