
Concept explainers
A large, flat sheet carries a uniformly distributed
the sheet radiates an
(a) Find the wave function for the electric field of the wave to the right of the sheet. (b) Find the Poynting vector as a function of x and t. (c) Find the intensity of the wave. (d) What If? If the sheet is to emit
Figure P33.28
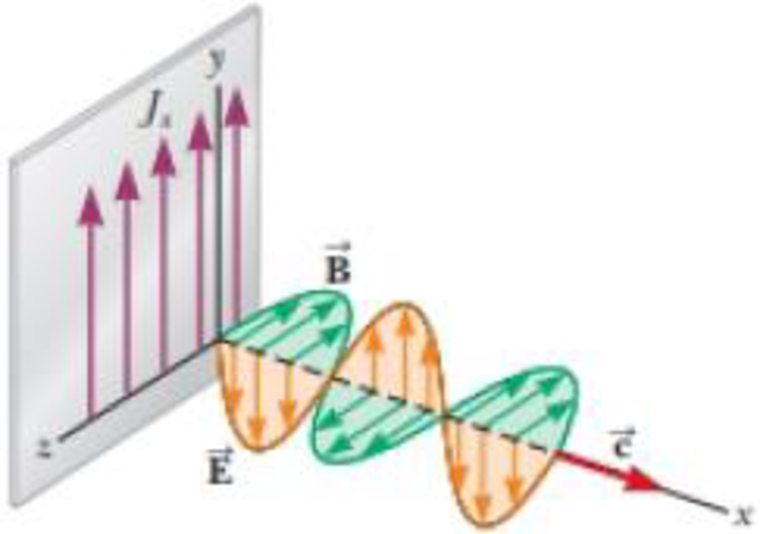
(a)
The wave function for the electric field of the wave to the right of the sheet.
Answer to Problem 46P
The wave function for the electric field of the wave to the right of the sheet is
Explanation of Solution
Given info: The wave function for the magnetic field of the wave to the right of the sheet is
Write the Maxwell’s third equation,
Here,
Substitute
Integrating the above equation with respect to
Substitute
The direction of electric field must be perpendicular to the direction of propagation
Conclusion:
Therefore, the wave function for the electric field of the wave to the right of the sheet is
(b)
The Poynting vector as a function of
Answer to Problem 46P
The Poynting vector as a function of
Explanation of Solution
Given info: The wave function for the magnetic field of the wave to the right of the sheet is
Write the formula to calculate the Poynting vector.
Here,
Substitute
Conclusion:
Therefore, the Poynting vector as a function of
(c)
The intensity of the wave.
Answer to Problem 46P
The intensity of the wave is
Explanation of Solution
Given info: The wave function for the magnetic field of the wave to the right of the sheet is
The wave function for the magnetic field of the wave is.
The maximum value of
The wave function for the electric field of the wave is.
The maximum value of
Write the formula to calculate the intensity of the wave is,
Here,
Substitute
Conclusion:
Therefore, the intensity of the wave is
(d)
The maximum value of sinusoidal current density.
Answer to Problem 46P
The maximum value of sinusoidal current density is
Explanation of Solution
Given info: The intensity of the wave is
The intensity of the wave from part (c) is,
Here,
Rearrange the above expression for
Substitute
Conclusion:
Therefore, the maximum value of sinusoidal current density is
Want to see more full solutions like this?
Chapter 34 Solutions
Physics for Scientists and Engineers With Modern Physics
- Find the ratio of the diameter of silver to iron wire, if they have the same resistance per unit length (as they might in household wiring). d. Ag dFe = 2.47 ×arrow_forwardFind the ratio of the diameter of silver to iron wire, if they have the same resistance per unit length (as they might in household wiring). d Ag = 2.51 dFe ×arrow_forwardShow that the units 1 v2/Q = 1 W, as implied by the equation P = V²/R. Starting with the equation P = V²/R, we can get an expression for a watt in terms of voltage and resistance. The units for voltage, V, are equivalent to [? v2 v2 A, are equivalent to J/C ✓ X . Therefore, 1 = 1 = 1 A V1 J/s Ω V-A X = 1 W. . The units for resistance, Q, are equivalent to ? The units for current,arrow_forward
 Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
 Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill
Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill





