Chapter 3.3, Problem 14P

Determine the stretch in each spring for equilibrium of the 2-kg block. The springs are shown in the equilibrium position.

To determine

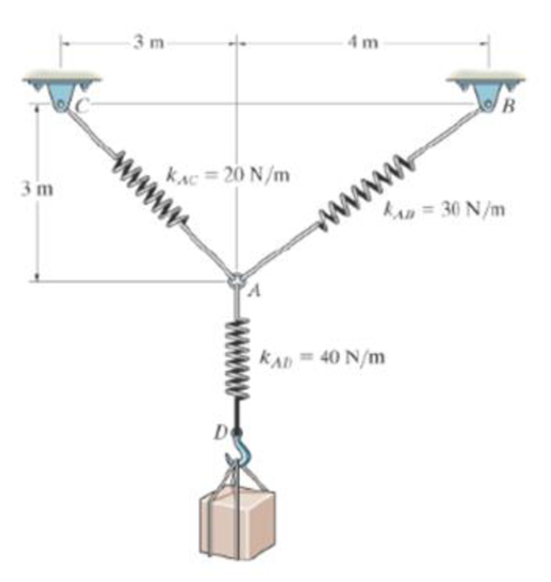
The stretch in each spring for the equilibrium of the block.

Answer to Problem 14P

The stretch in spring AD, $\underset{\_}{x_{AD}=0.4905\text{m}}$.

The stretch in spring AC, $\underset{\_}{x_{AC}=0.793\text{m}}$.

The stretch in spring AB, $\underset{\_}{x_{AB}=0.467\text{m}}$.

Explanation of Solution

Given information:

• The mass of the block (m) is 2 kg.

Assumption:

Consider point E in between the points B and C.

Show the free body diagram of the springs in the equilibrium position as in Figure 1.

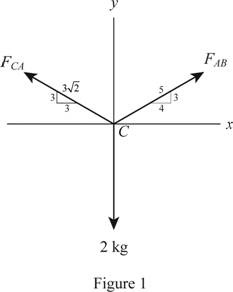
Determine the length of the spring AC using the formula.

$l_{AC}=\sqrt{l_{AE}^2+l_{CE}^2}$ (I)

Here, the length of the point AE is $l_{AE}$ and length of the point AB is $l_{AB}$.

Determine the length of the spring AB using the formula.

$l_{AB}=\sqrt{l_{AE}^2+l_{BE}^2}$ (II)

Here, the length of the point BE is $l_{BE}$.

Determine the force in spring AD using the formula.

$F_{AD}=mg$ (III)

Here, the mass of the block is m and the acceleration due to gravity is g.

Determine the stretch in spring AD.

$F_{AD}=k_{AD}{x}_{AD}$ (IV)

Here, the stiffness of the spring AD is $k_{AD}$.

Determine the stretch in the springs AC and AB for equilibrium of the block by applying the equation of equilibrium.

Along the horizontal direction:

$\begin{array}{l}{\displaystyle \sum F_x=0}\\ F_{AB}\cos {\theta }_{AB}-F_{AC}\cos {\theta }_{AC}=0\end{array}$ (V)

Here, the force acting on spring AB is $F_{AB}$, the angle for the force AB is ${\theta }_{AB}$, the force acting on cable AC is $F_{AC}$, and the angle for the force AC is ${\theta }_{AC}$.

Along the vertical direction:

$\begin{array}{l}{\displaystyle \sum F_y=0}\\ F_{AB}\sin {\theta }_{AB}+F_{AC}\sin {\theta }_{AC}-F_{AD}=0\end{array}$ (VI)

Determine the stretch in spring AC.

$F_{AC}=k_{AC}{x}_{AC}$ (VII)

Here, the stiffness of the spring AC is $k_{AC}$.

Determine the stretch in spring AB.

$F_{AB}=k_{AB}{x}_{AB}$ (VIII)

Here, the stiffness of the spring AB is $k_{AB}$.

Conclusion:

Substitute 3 m for $l_{AE}$ and 3 m for $l_{CE}$ in Equation (I).

$\begin{array}{c}{l}_{AC}=\sqrt{3^2+3^2}\\ =3\sqrt{2}\text{m}\end{array}$

Substitute 3 m for $l_{AE}$ and 4 m for $l_{BE}$ in Equation (II).

$\begin{array}{c}{l}_{AB}=\sqrt{3^2+4^2}\\ =5\text{m}\end{array}$

Substitute 2 kg for m and $9.81\text{m}/{\text{s}}^{\text{2}}$ for g in Equation (III).

$\begin{array}{c}{F}_{AD}=2\times 9.81\\ =19.62\frac{\text{kg}\cdot \text{m}}{{\text{s}}^{\text{2}}}\times \frac{1\text{N}}{\frac{\text{kg}\cdot \text{m}}{{\text{s}}^{\text{2}}}}\\ =19.62\text{N}\end{array}$

Substitute 19.62 N for $F_{AD}$ and $40\text{N}/\text{m}$ for $k_{AD}$ in Equation (IV).

$\begin{array}{l}19.62=40x_{AD}\\ x_{AD}=\frac{19.62}{40}\\ x_{AD}=0.4905\text{m}\end{array}$

Thus, the stretch in spring AD is $\underset{\_}{0.4905\text{m}}$.

Determine the $\cos {\theta }_{AB}$ value.

$\cos {\theta }_{AB}=\frac{l_{BE}}{l_{AB}}$

Substitute 4 m for $l_{BE}$ and 5 m for $l_{AB}$.

$\cos {\theta }_{AB}=\frac{4}{5}$

Determine the $\cos {\theta }_{AC}$ value.

$\cos {\theta }_{AC}=\frac{l_{CE}}{l_{AC}}$

Substitute 3m for $l_{CE}$ and $3\sqrt{2}$ m for $l_{AC}$.

$\begin{array}{c}\cos {\theta }_{AC}=\frac{3}{3\sqrt{2}}\\ =\frac{1}{\sqrt{2}}\end{array}$

Substitute $\frac{4}{5}$ for $\cos {\theta }_{AB}$ and $\frac{1}{\sqrt{2}}$ for $\cos {\theta }_{AC}$ in Equation (V).

$\begin{array}{l}{F}_{AB}\left(\frac{4}{5}\right)-F_{AC}\left(\frac{1}{\sqrt{2}}\right)=0\\ F_{AB}\left(\frac{4}{5}\right)=F_{AC}\left(\frac{1}{\sqrt{2}}\right)\\ F_{AB}=F_{AC}\left(\frac{5}{4\sqrt{2}}\right)\end{array}$ (IX)

Determine the $\sin {\theta }_{AB}$ value.

$\sin {\theta }_{AB}=\frac{l_{AE}}{l_{AB}}$

Substitute 3 m for $l_{AE}$ and 5 m for $l_{AB}$.

$\sin {\theta }_{AB}=\frac{3}{5}$

Determine the $\sin {\theta }_{AC}$ value.

$\sin {\theta }_{AC}=\frac{l_{AE}}{l_{AC}}$

Substitute 3m for $l_{AE}$ and $3\sqrt{2}$ m for $l_{AC}$.

$\begin{array}{c}\sin {\theta }_{AC}=\frac{3}{3\sqrt{2}}\\ =\frac{1}{\sqrt{2}}\end{array}$

Substitute $F_{AC}\left(\frac{5}{4\sqrt{2}}\right)$ for $F_{AB}$, $\frac{3}{5}$ for $\sin {\theta }_{AB}$, $\frac{1}{\sqrt{2}}$ for $\sin {\theta }_{AC}$, 19.62 N for $F_{AD}$ in Equation (VI).

$\begin{array}{l}{F}_{AC}\left(\frac{5}{4\sqrt{2}}\right)\left(\frac{3}{5}\right)+F_{AC}\left(\frac{1}{\sqrt{2}}\right)-19.62=0\\ \frac{F_{AC}}{\sqrt{2}}\left(\frac{3}{4}+1\right)=19.62\\ F_{AC}\left(\frac{7}{4}\right)=19.62\times \sqrt{2}\\ F_{AC}=\frac{19.62\times \sqrt{2}\times 4}{7}\end{array}$

$F_{AC}=15.86\text{N}$

Substitute 15.86 N for $F_{AC}$ and $20\text{N}/\text{m}$ for $k_{AC}$ in Equation (VII).

$\begin{array}{l}15.86=20\times x_{AC}\\ x_{AC}=\frac{15.86}{20}\\ x_{AC}=0.793\text{m}\end{array}$

Thus, the stretch in spring AC is $\underset{\_}{0.793\text{m}}$.

Substitute 15.86 N for $F_{AC}$ in Equation (IX).

$\begin{array}{c}{F}_{AB}=15.86\left(\frac{5}{4\sqrt{2}}\right)\\ =14.01\text{N}\end{array}$

Substitute 14.01 N for $F_{AB}$ and $30\text{N}/\text{m}$ for $k_{AB}$ in Equation (VIII).

$\begin{array}{l}14.01=30\times x_{AB}\\ x_{AB}=\frac{14.01}{30}\\ x_{AB}=0.467\text{m}\end{array}$

Thus, the stretch in spring AB is $\underset{\_}{0.467\text{m}}$.