Numerical Analysis
10th Edition
ISBN: 9781305253667
Author: Richard L. Burden, J. Douglas Faires, Annette M. Burden
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 3.3, Problem 11ES
The following data are given for a polynomial P(x) of unknown degree.
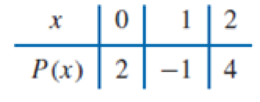
Determine the coefficient of x2 in P(x) if all third-order forward differences are 1.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Business Discuss
Does the series converge or diverge
Does the series converge or diverge
Chapter 3 Solutions
Numerical Analysis
Ch. 3.1 - For the given functions f(x), let x0 = 0, x1 =...Ch. 3.1 - Use Theorem 3.3 to find an error bound for the...Ch. 3.1 - Prob. 4ESCh. 3.1 - The data for Exercise 6 were generated using the...Ch. 3.1 - Prob. 9ESCh. 3.1 - Prob. 10ESCh. 3.1 - Prob. 11ESCh. 3.1 - Prob. 12ESCh. 3.1 - Prob. 15ESCh. 3.1 - Prob. 17ES
Ch. 3.1 - It is suspected that the high amounts of tannin in...Ch. 3.1 - Prob. 21ESCh. 3.1 - Prove Taylors Theorem 1.14 by following the...Ch. 3.1 - Prob. 1DQCh. 3.1 - If we decide to increase the degree of the...Ch. 3.2 - Let P3(x) be the interpolating polynomial for the...Ch. 3.2 - Nevilles method is used to approximate f(0.4),...Ch. 3.2 - Nevilles method is used to approximate f(0.5),...Ch. 3.2 - Suppose xj = j, for j = 0, 1, 2, 3, and it is...Ch. 3.2 - Nevilles Algorithm is used to approximate f(0)...Ch. 3.2 - Prob. 11ESCh. 3.2 - Prob. 13ESCh. 3.2 - Can Nevilles method be used to obtain the...Ch. 3.3 - Use Eq. (3.10) or Algorithm 3.2 to construct...Ch. 3.3 - Use Eq. (3.10) or Algorithm 3.2 to construct...Ch. 3.3 - Use the Newton forward-difference formula to...Ch. 3.3 - Use the Newton forward-difference formula to...Ch. 3.3 - Use the Newton backward-difference formula to...Ch. 3.3 - Use the Newton backward-difference formula to...Ch. 3.3 - a. Use Algorithm 3.2 to construct the...Ch. 3.3 - a. Use Algorithm 3.2 to construct the...Ch. 3.3 - a. Approximate f(0.05) using the following data...Ch. 3.3 - The following data are given for a polynomial P(x)...Ch. 3.3 - The following data are given for a polynomial P(x)...Ch. 3.3 - The Newton forward-difference formula is used to...Ch. 3.3 - Prob. 14ESCh. 3.3 - Prob. 16ESCh. 3.3 - Prob. 17ESCh. 3.3 - Show that the polynomial interpolating the...Ch. 3.3 - Prob. 20ESCh. 3.3 - Prob. 21ESCh. 3.3 - Prob. 22ESCh. 3.3 - Prob. 23ESCh. 3.3 - Compare and contrast the various...Ch. 3.3 - Is it easier to add a new data pair using...Ch. 3.3 - Prob. 3DQCh. 3.4 - Use Theorem 3.9 or Algorithm 3.3 to construct an...Ch. 3.4 - Use Theorem 3.9 or Algorithm 3.3 to construct an...Ch. 3.4 - The data in Exercise 1 were generated using the...Ch. 3.4 - The data in Exercise 2 were generated using the...Ch. 3.4 - Let f (x) = 3xex e2x. a. Approximate f (1.03) by...Ch. 3.4 - The following table lists data for the function...Ch. 3.4 - a. Show that H2n + 1 (x) is the unique polynomial...Ch. 3.4 - Prob. 1DQCh. 3.4 - Prob. 2DQCh. 3.4 - Prob. 3DQCh. 3.5 - Determine the natural cubic spline S that...Ch. 3.5 - Determine the clamped cubic spline s that...Ch. 3.5 - Construct the natural cubic spline for the...Ch. 3.5 - Construct the natural cubic spline for the...Ch. 3.5 - The data in Exercise 3 were generated using the...Ch. 3.5 - Prob. 6ESCh. 3.5 - Prob. 8ESCh. 3.5 - Prob. 11ESCh. 3.5 - Prob. 12ESCh. 3.5 - Prob. 13ESCh. 3.5 - Prob. 14ESCh. 3.5 - Given the partition x0 = 0, x1 = 0.05, and x2 =...Ch. 3.5 - Prob. 16ESCh. 3.5 - Prob. 21ESCh. 3.5 - Prob. 22ESCh. 3.5 - Prob. 23ESCh. 3.5 - It is suspected that the high amounts of tannin in...Ch. 3.5 - Prob. 29ESCh. 3.5 - Prob. 30ESCh. 3.5 - Prob. 31ESCh. 3.5 - Prob. 32ESCh. 3.5 - Let f C2[a, b] and let the nodes a = x0 x1 xn...Ch. 3.5 - Prob. 34ESCh. 3.5 - Prob. 35ESCh. 3.6 - Let (x0, y0) = (0,0) and (x1, y1) = (5, 2) be the...Ch. 3.6 - Prob. 2ESCh. 3.6 - Prob. 5ESCh. 3.6 - Prob. 1DQ
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- Suppose that a particle moves along a straight line with velocity v (t) = 62t, where 0 < t <3 (v(t) in meters per second, t in seconds). Find the displacement d (t) at time t and the displacement up to t = 3. d(t) ds = ["v (s) da = { The displacement up to t = 3 is d(3)- meters.arrow_forwardLet f (x) = x², a 3, and b = = 4. Answer exactly. a. Find the average value fave of f between a and b. fave b. Find a point c where f (c) = fave. Enter only one of the possible values for c. c=arrow_forwardThe following data represent total ventilation measured in liters of air per minute per square meter of body area for two independent (and randomly chosen) samples. Analyze these data using the appropriate non-parametric hypothesis testarrow_forward
- each column represents before & after measurements on the same individual. Analyze with the appropriate non-parametric hypothesis test for a paired design.arrow_forwardShould you be confident in applying your regression equation to estimate the heart rate of a python at 35°C? Why or why not?arrow_forwardGiven your fitted regression line, what would be the residual for snake #5 (10 C)?arrow_forward
- Calculate the 95% confidence interval around your estimate of r using Fisher’s z-transformation. In your final answer, make sure to back-transform to the original units.arrow_forwardCalculate Pearson’s correlation coefficient (r) between temperature and heart rate.arrow_forwardCalculate the least squares regression line and write the equation.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
College Algebra (MindTap Course List)
Algebra
ISBN:9781305652231
Author:R. David Gustafson, Jeff Hughes
Publisher:Cengage Learning
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning
College Algebra
Algebra
ISBN:9781305115545
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Finding Local Maxima and Minima by Differentiation; Author: Professor Dave Explains;https://www.youtube.com/watch?v=pvLj1s7SOtk;License: Standard YouTube License, CC-BY