
Certain solid substances, known as hydrated compounds, have well-defined molecular ratios of water to some other species. For example, calcium sulfate dihydrate (commonly known as gypsum, CaSO42H2O), has 2 moles of water per mole of calcium sulfate; alternatively, it may be said that 1 mole of gypsum consists of 1 mole of calcium sulfate and 2 moles of water. The water in such substances is called water of hydration. (More information about hydrated salts is given in Chapter 6.)
In order to eliminate the discharge of sulfuric acid into the environment, a process has been developed in which the acid is reacted with aragonite (CaCO3) to produce calcium sulfate. The calcium sulfate then comes out of solution in a crystallizer to form a slurry (a suspension of solid particles in a liquid) of solid gypsum particles suspended in an aqueous CaSO4 solution. The slurry flows front tlte crystallizer to a filter in which the particles arc collected as a filter cake. The filter cake, which is 95.0 wt% solid gypsum and the remainder CaSO4 solution, is fed to a dryer in which all water (including the water of hydration in the crystals) is driven off to yield anhydrous (water-free) CaSO4 as product A flowchart and relevant process data arc given below.
Solids content of slurry leaving crystallizer 0.35 kg CaSO4-2H2O/L slurry CaSO4 content of slurry liquid: 0.209g CaSO4/100g H2O Specific gravities: CaSO4-2H2O(s), 2.32; liquid solutions, 1.05
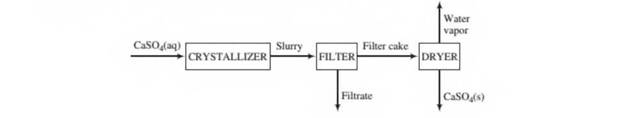
- Briefly explain in your own words the functions of the three units (crystallizer, filter, and dryer).
- Take a basis of one liter of solution leaving the crystallizer and calculate tlte mass (kg) and volume (L) of solid gypsum, the mass of CaSO4 in the gypsum, and the mass of CaSO4 in the liquid solution.
- Calculate the percentage recovery of CaSO4—that is, the percentage of the total CaSO4(precipitated plus dissolved) leaving the crystallizer recovered as solid anhydrous CaSO4.
- List five potential negative consequences of discharging H2SO4 into the river passing the plant.
Want to see the full answer?
Check out a sample textbook solution
Chapter 3 Solutions
ELEM PRIN CHEM PROCESS ETXT + WILEYPLUS
Additional Engineering Textbook Solutions
Database Concepts (8th Edition)
Starting Out with Java: From Control Structures through Data Structures (4th Edition) (What's New in Computer Science)
Starting Out with C++ from Control Structures to Objects (9th Edition)
Starting Out with Java: From Control Structures through Objects (7th Edition) (What's New in Computer Science)
Thinking Like an Engineer: An Active Learning Approach (4th Edition)
Starting Out with Programming Logic and Design (5th Edition) (What's New in Computer Science)
- Thermophysical Properties of Petroleum Fractions and Crude Ofls 67 3.4. A gas oil has the following TBP distillation data Volume % TBP (°C) 0 216 10 243 30 268 50 284 70 304 90 318 95 327 100 334 It also has an average boiling point of 280 °C and an average density of 0.850 g/cm³. (a) Split this gas oil fraction into five pseudo-components. Calculate T., Pc and w for each pseudo-component. (b) Calculate T, Pc and w for the whole gas oil fraction. (c) Calculate the enthalpy of this gas oil fraction at 400 °C using the Lee- Kessler correlation with a reference state of ideal gas at 273.15 K.arrow_forward3.3. Use the following crude assay data with crude API of 36 to estimate cut vol%, critical properties and molecular weight for Light Naphtha (90- 190 °F) and Kerosene (380-520 °F). In addition, calculate the fractions of paraffins, naphthenes and aromatics in the two cuts. ASTM D86 (°F) Volume % Cum vol% SG 86 0.0 0.0 122 0.5 0.5 0.6700 167 1.2 1.7 0.6750 212 1.6 3.3 0.7220 257 2.7 6.0 0.7480 302 3.1 9.1 0.7650 347 3.9 13.0 0.7780 392 4.7 17.7 0.7890 437 5.7 23.4 0.8010 482 8.0 31.4 0.8140 527 10.7 42.1 0.8250 584 5.0 47.1 0.8450 636 10.0 57.1 0.8540 689 7.8 64.9 0.8630 742 7.0 71.9 0.8640 794 6.5 78.4 0.8890 20.8 99.2 0.9310arrow_forward۱۱۳ ۱۱۱۰ ۱۱۰ A + C Chegg Learn on the go = Chegg © chegg.com/homewo Open in app EN-US QUESTIONS AND PROBLEMS 4.1. With 100,000 BPD of the following crude (API = 36), estimate the products of the atmospheric distillation column. If the atmospheric residue of the crude is taken at 650+ F. It enters in a vacuum distilla- tion tower to give three products: light vacuum gas oil (650-850 °F), heavy vacuum gas oil (850-1050 °F) and vacuum residue (1050+ °F). Calculate the mass flow rate of these products. Then calculate the sulphur content (lb/hr) for each product. ASTM D86 (°F) vol% Cum vol% SG 86 0.0 0.0 122 0.5 0.5 0.6700 167 1.2 1.7 0.6750 212 1.6 3.3 0.7220 257 2.7 6.0 0.7480 3021 3.1 9.1 0.7650 347 3.9 13.0 0.7780 392 4.7 17.7 0.7890 437 5.7 23.4 0.8010 4821 8.0 31.41 0.8140 527 10,7 42.1 0.8250 584 5.0 47.1 0.8450 6361 10,0 57.1 0.8540 689 7,8 64.9 0.8630 7421 7.0 71.9 0.8640 794 6.5 78.4 0.8890 20.8 99.2 0.9310 Show transcribed image text Here's the best way to solve it. This problem…arrow_forward
- Q1/obtain the transfer function for the block diagram shown in the figure below: G4 Garrow_forward(Population density parameters from sieve analysis data)2 One hundred fifty grams of crystals separated from one litre of suspension from an MSMPR crystallizer is subjected to screen analysis to get the following data: Tyler mesh Mass(g) 12/14 28.5 14/20 29.2 20/28 28/35 35/48 below 48 mesh 37.5 27 24.7 3.1 Mesh no./ screen opening(um) data: 12/1410 μm; 14/1190; 20/841; 28/595; 35/420; 48/297. The working volume of the crystallizer is 200 litres, and the rate of withdrawal of the slurry is 250 litre per hour. Given pc = 1400 kg/m³ and volume shape factor o, = 0.42, determine the crystal growth rate and the zero-size population density of the crystals. What is the rate of nucleation, Bº?arrow_forwardneed help with this phase transformations practise questionarrow_forward
- help with this practise question on phase transformations.arrow_forwardDifferentiate between an ideal and a regular solution consisting of a mixture of A and B atoms. Which of these solutions, is likely to contain a random mixture of atoms at all temperatures? For the binary A-B ideal-solution, differentiate the equation for the configurational entropy of mixing with respect to concentration. Hence show that the slope of the free energy of mixing versus concentration curve is towards tinfinity when the mole fraction is 0 or 1. Does this make it easy or hard to purify materials? [50%] (ii) How can a phase that has a limited solubility for a particular solute be forced to accept larger concentrations which far exceed its equilibrium solubility? [20%] (iii) Atoms of A and B are arranged in a straight line at random, with the mole fraction of B equal to x. What is the probability of finding two A atoms next to each other? How would your calculation be modified if this were to be a two-dimensional array of A and B atoms? [20%] (iv) An alloy is to be made,…arrow_forwardCan the method steps be given for these questions please 10 answer given is 0.01m/s 11 answer given is 0.067e Cnm where e is charge of electron divided by volume of unit cell, giving 0.165 C/m^2 12 answer is 0.08%arrow_forward
- 3. Differentiate f(x) = x² sin(x). 4. Evaluate the limit: lim x 0 sin(2x) Xarrow_forwardDifferentiate between an ideal and a regular solution consisting of a mixture of A andB atoms. Which of these solutions, is likely to contain a random mixture of atomsat all temperatures? For the binary A-B ideal-solution, differentiate the equationfor the configurational entropy of mixing with respect to concentration. Hence showthat the slope of the free energy of mixing versus concentration curve is towards±infinity when the mole fraction is 0 or 1. Does this make it easy or hard to purifymaterials?arrow_forwardQuestion During the solidification of a binary alloy, with a positive temperature gradient in the melt, a planar solid-liquid interface is moving at the steady state, Fig. Q1(i). The variation of the solute concentration, C, in the melt ahead of the interface is given by, b) If m is the liquidus gradient, or the slope of liquidus, Fig.Q1 (iv), how does the equilibrium temperature, T, vary with the melt composition C? T₁ = C=C1+ exp R.x D (equation 1.1) T L Solid Melt (iv) T₁ S S+L where Co is the nominal solute concentration in the alloy, Ko is the equilibrium distribution coefficient, R is the solid-liquid interface moving rate, D is the solute diffusivity in the melt and x is distance into the liquid phase, Fig. Q1(ii). Answer the questions in the steps below, to show that the level of constitutional supercooling is governed by both the actual temperature, T, and the composition, C, in the solidification front. a) Consider a point in the melt at a distance x away from the solid/melt…arrow_forward
 Introduction to Chemical Engineering Thermodynami...Chemical EngineeringISBN:9781259696527Author:J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark SwihartPublisher:McGraw-Hill Education
Introduction to Chemical Engineering Thermodynami...Chemical EngineeringISBN:9781259696527Author:J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark SwihartPublisher:McGraw-Hill Education Elementary Principles of Chemical Processes, Bind...Chemical EngineeringISBN:9781118431221Author:Richard M. Felder, Ronald W. Rousseau, Lisa G. BullardPublisher:WILEY
Elementary Principles of Chemical Processes, Bind...Chemical EngineeringISBN:9781118431221Author:Richard M. Felder, Ronald W. Rousseau, Lisa G. BullardPublisher:WILEY Elements of Chemical Reaction Engineering (5th Ed...Chemical EngineeringISBN:9780133887518Author:H. Scott FoglerPublisher:Prentice Hall
Elements of Chemical Reaction Engineering (5th Ed...Chemical EngineeringISBN:9780133887518Author:H. Scott FoglerPublisher:Prentice Hall
 Industrial Plastics: Theory and ApplicationsChemical EngineeringISBN:9781285061238Author:Lokensgard, ErikPublisher:Delmar Cengage Learning
Industrial Plastics: Theory and ApplicationsChemical EngineeringISBN:9781285061238Author:Lokensgard, ErikPublisher:Delmar Cengage Learning Unit Operations of Chemical EngineeringChemical EngineeringISBN:9780072848236Author:Warren McCabe, Julian C. Smith, Peter HarriottPublisher:McGraw-Hill Companies, The
Unit Operations of Chemical EngineeringChemical EngineeringISBN:9780072848236Author:Warren McCabe, Julian C. Smith, Peter HarriottPublisher:McGraw-Hill Companies, The





