
Bundle: Elementary Linear Algebra, Loose-leaf Version, 8th + WebAssign Printed Access Card for Larson's Elementary Linear Algebra, 8th Edition, Single-Term
8th Edition
ISBN: 9781337604925
Author: Ron Larson
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Concept explainers
Question
Chapter 2.6, Problem 16E
To determine
a.
To sketch:
The line that appears to be the best fit for the given points in the graph
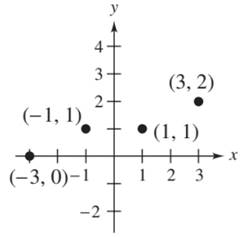
To determine
b.
The least square regression line for the graph
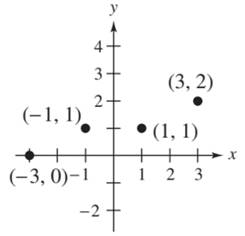
To determine
c.
The sum of the squared error for the graph
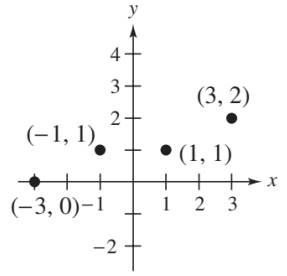
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
2) If Mand N be two water hyper Plane ofx
Show that MUN and MN is hy Per Plane
ofx with prove and Examplame.
or
3) IS AUB is convex set and affine set
or blensed set or symmetre setorsubsie....
Show that A and B is convex or affine
or Hensedsed or symmetivce or subspace.
4) 18 MUN is independence show that
Prove or ExPlane Mand Nave independend.
or not.
5) Jet X be Vector Pace over I show that is xty
tnx st Xty 3 fix→ F s-t
f(x)
(9)
Jet Mand N be two blanced set of Xbe
Vector space show tha MUNIS ansed set
Find a polynomial with integer coefficients that satisfies the given conditions. T(x) has degree 4, zeros i and 1 + i, and constant term 12.
How to solve 2542000/64132 without a calculator?
Chapter 2 Solutions
Bundle: Elementary Linear Algebra, Loose-leaf Version, 8th + WebAssign Printed Access Card for Larson's Elementary Linear Algebra, 8th Edition, Single-Term
Ch. 2.1 - Equality of Matrices In Exercises 1-4, find x and...Ch. 2.1 - Equality of Matrices In Exercises 1-4, find x and...Ch. 2.1 - Equality of Matrices In Exercises 1-4, find x and...Ch. 2.1 - Equality of Matrices In Exercises 1-4, find x and...Ch. 2.1 - Operations with Matrices In Exercises 5-10, find,...Ch. 2.1 - Operations with Matrices In Exercises 5-10, find,...Ch. 2.1 - Operations with Matrices In Exercises 5-10, find,...Ch. 2.1 - Operations with Matrices In Exercises 5-10, find,...Ch. 2.1 - Operations with Matrices In Exercises 5-10, find,...Ch. 2.1 - Operations with Matrices In Exercises 5-10, find,...
Ch. 2.1 - Find a c21 and b c13, where C=2A-3B, A=544-312,...Ch. 2.1 - Find a c23 and b c32, where C=5A+2B,...Ch. 2.1 - Solve for x,y and z in the matrix equation...Ch. 2.1 - Solve for x,y,z and w in the matrix equation...Ch. 2.1 - Prob. 15ECh. 2.1 - Prob. 16ECh. 2.1 - Finding Products of Two Matrices In Exercises...Ch. 2.1 - Finding Products of Two Matrices In Exercises...Ch. 2.1 - Finding Products of Two Matrices In Exercises...Ch. 2.1 - Finding Products of Two Matrices In Exercises...Ch. 2.1 - Prob. 21ECh. 2.1 - Prob. 22ECh. 2.1 - Prob. 23ECh. 2.1 - Prob. 24ECh. 2.1 - Prob. 25ECh. 2.1 - Prob. 26ECh. 2.1 - Prob. 27ECh. 2.1 - Prob. 28ECh. 2.1 - Matrix Size In Exercises 29-36, let A,B,C,D,andE...Ch. 2.1 - Matrix Size In Exercises 29-36, let A,B,C,D,andE...Ch. 2.1 - Prob. 31ECh. 2.1 - Prob. 32ECh. 2.1 - Matrix Size In Exercises 29-36, let A,B,C,D,andE...Ch. 2.1 - Matrix Size In Exercises 29-36, let A,B,C,D,andE...Ch. 2.1 - Matrix Size In Exercises 29-36, let A,B,C,D,andE...Ch. 2.1 - Matrix Size In Exercises 29-36, let A,B,C,D,andE...Ch. 2.1 - Solving a Matrix Equation In Exercises 37 and 38,...Ch. 2.1 - Solving a Matrix Equation In Exercises 37 and 38,...Ch. 2.1 - Prob. 39ECh. 2.1 - Solving a System of Linear Equations In Exercises...Ch. 2.1 - Solving a System of Linear Equations In Exercises...Ch. 2.1 - Prob. 42ECh. 2.1 - Solving a System of Linear Equations In Exercises...Ch. 2.1 - Solving a System of Linear Equations In Exercises...Ch. 2.1 - Prob. 45ECh. 2.1 - Solving a System of Linear Equations In Exercises...Ch. 2.1 - Prob. 47ECh. 2.1 - Prob. 48ECh. 2.1 - Writing a Linear Combination In Exercises 49-52,...Ch. 2.1 - Writing a Linear Combination In Exercises 49-52,...Ch. 2.1 - Writing a Linear Combination In Exercises 49-52,...Ch. 2.1 - Prob. 52ECh. 2.1 - Solving a Matrix Equation In Exercises 53 and 54,...Ch. 2.1 - Solving a Matrix Equation In Exercises 53 and 54,...Ch. 2.1 - Solving a Matrix Equation In Exercises 55 and 56,...Ch. 2.1 - Solving a Matrix Equation In Exercises 55 and 56,...Ch. 2.1 - Prob. 57ECh. 2.1 - Prob. 58ECh. 2.1 - Finding Product of Diagonal Matrices In Exercises...Ch. 2.1 - Finding Product of Diagonal Matrices In Exercises...Ch. 2.1 - Guide Proof Prove that if A and B are diagonal...Ch. 2.1 - Prob. 62ECh. 2.1 - Trace of a matrix In Exercises 63-66, find the...Ch. 2.1 - Trace of a matrix In Exercises 63-66, find the...Ch. 2.1 - Prob. 65ECh. 2.1 - Prob. 66ECh. 2.1 - Proof Prove that each statement is true when A and...Ch. 2.1 - Proof Prove that if A and B are square matrices of...Ch. 2.1 - Find conditions on w,x,y,andz such that AB=BA for...Ch. 2.1 - Prob. 70ECh. 2.1 - Prob. 71ECh. 2.1 - Show that no 22 matrices A and B exist that...Ch. 2.1 - Prob. 73ECh. 2.1 - Prob. 74ECh. 2.1 - Prob. 75ECh. 2.1 - Prob. 76ECh. 2.1 - Prob. 77ECh. 2.1 - Prob. 78ECh. 2.1 - Agriculture A fruit grower raises two crops,...Ch. 2.1 - Prob. 80ECh. 2.1 - Prob. 81ECh. 2.1 - Prob. 82ECh. 2.1 - Prob. 83ECh. 2.1 - Prob. 84ECh. 2.1 - True or False? In Exercises 85 and 86, determine...Ch. 2.1 - True or False In Exercises 85 and 86, determine...Ch. 2.1 - Prob. 87ECh. 2.2 - Evaluating an Expression In Exercise 1-6, evaluate...Ch. 2.2 - Evaluating an Expression In Exercise 1-6, evaluate...Ch. 2.2 - Prob. 3ECh. 2.2 - Prob. 4ECh. 2.2 - Prob. 5ECh. 2.2 - Evaluating an Expression In Exercise 1-6, evaluate...Ch. 2.2 - Prob. 7ECh. 2.2 - Prob. 8ECh. 2.2 - Operations with Matrices In Exercises 7-12,...Ch. 2.2 - Prob. 10ECh. 2.2 - Operations with Matrices In Exercises 7-12,...Ch. 2.2 - Prob. 12ECh. 2.2 - Solve for X in the Equation, given A=-401-532 and...Ch. 2.2 - Solve for X in the Equation, given A=-2-1103-4 and...Ch. 2.2 - Operations with Matrices In Exercises 15-22,...Ch. 2.2 - Prob. 16ECh. 2.2 - Operations with Matrices In Exercises 15-22,...Ch. 2.2 - Prob. 18ECh. 2.2 - Operations with Matrices In Exercises 15-22,...Ch. 2.2 - Prob. 20ECh. 2.2 - Prob. 21ECh. 2.2 - Operations with Matrices In Exercises 15-22,...Ch. 2.2 - Associativity of Matrix Multiplication In...Ch. 2.2 - Prob. 24ECh. 2.2 - Noncommutativity of Matrix Multiplication In...Ch. 2.2 - Noncommutativity of Matrix Multiplication In...Ch. 2.2 - Prob. 27ECh. 2.2 - Equal Matrix Products In Exercises 27 and 28, show...Ch. 2.2 - Zero Matrix Product In Exercises 29 and 30, show...Ch. 2.2 - Zero Matrix Product In Exercises 29 and 30, show...Ch. 2.2 - Prob. 31ECh. 2.2 - Prob. 32ECh. 2.2 - Prob. 33ECh. 2.2 - Operations with Matrices In Exercises 31-36,...Ch. 2.2 - Operations with Matrices In Exercises 31-36,...Ch. 2.2 - Prob. 36ECh. 2.2 - Writing In Exercises 37 and 38, explain why the...Ch. 2.2 - Prob. 38ECh. 2.2 - Finding the Transpose of a Matrix In Exercises 39...Ch. 2.2 - Finding the Transpose of a Matrix In Exercises 39...Ch. 2.2 - Finding the Transpose of a product of Two Matrices...Ch. 2.2 - Finding the Transpose of a product of Two Matrices...Ch. 2.2 - Finding the Transpose of a product of Two Matrices...Ch. 2.2 - Finding the Transpose of a product of Two Matrices...Ch. 2.2 - Multiplication with the Transpose of a Matrix In...Ch. 2.2 - Multiplication with the Transpose of a Matrix In...Ch. 2.2 - Prob. 47ECh. 2.2 - Prob. 48ECh. 2.2 - Prob. 49ECh. 2.2 - Prob. 50ECh. 2.2 - Prob. 51ECh. 2.2 - Prob. 52ECh. 2.2 - Finding an nth Root of a Matrix In Exercises 53...Ch. 2.2 - Finding an nth Root of a Matrix In Exercises 53...Ch. 2.2 - Prob. 55ECh. 2.2 - Prob. 56ECh. 2.2 - Prob. 57ECh. 2.2 - CAPSTONE In the matrix equation aX+AbB=bAB+IB...Ch. 2.2 - Polynomial Function In Exercises 59 and 60, find...Ch. 2.2 - Polynomial Function In Exercises 59 and 60, find...Ch. 2.2 - Guided proof Prove the associative property of...Ch. 2.2 - Proof Prove the associative property of...Ch. 2.2 - Proof Prove that the scalar 1 is the identity for...Ch. 2.2 - Proof Prove the distributive property: c+dA=cA+dA.Ch. 2.2 - Prob. 65ECh. 2.2 - Prob. 66ECh. 2.2 - Prob. 67ECh. 2.2 - Proof Prove properties 2, 3, and 4 of Theorem 2.6.Ch. 2.2 - GuidedProof Prove that if A is an mn matrix, then...Ch. 2.2 - Prob. 70ECh. 2.2 - Prob. 71ECh. 2.2 - Symmetric and Skew-Symmetric Matrices In Exercises...Ch. 2.2 - Prob. 73ECh. 2.2 - Prob. 74ECh. 2.2 - Proof Prove that the main diagonal of a...Ch. 2.2 - Proof Prove that if A and B are nn skew-symmetric...Ch. 2.2 - Proof Let A be a square matrix of order n. a Show...Ch. 2.2 - Proof Prove that if A is an nn matrix, then A-AT...Ch. 2.2 - Prob. 79ECh. 2.3 - The Inverse of a Matrix In Exercises 1-6, show...Ch. 2.3 - Prob. 2ECh. 2.3 - Prob. 3ECh. 2.3 - The Inverse of a Matrix In Exercises 1-6, show...Ch. 2.3 - The Inverse of a Matrix In Exercises 1-6, show...Ch. 2.3 - Prob. 6ECh. 2.3 - Finding the Inverse of a Matrix In Exercises 7-30,...Ch. 2.3 - Prob. 8ECh. 2.3 - Finding the Inverse of a Matrix In Exercises 7-30,...Ch. 2.3 - Prob. 10ECh. 2.3 - Prob. 11ECh. 2.3 - Prob. 12ECh. 2.3 - Finding the Inverse of a Matrix In Exercises 7-30,...Ch. 2.3 - Finding the Inverse of a Matrix In Exercises 7-30,...Ch. 2.3 - Prob. 15ECh. 2.3 - Prob. 16ECh. 2.3 - Prob. 17ECh. 2.3 - Prob. 18ECh. 2.3 - Finding the Inverse of a Matrix In Exercises 7-30,...Ch. 2.3 - Finding the Inverse of a Matrix In Exercises 7-30,...Ch. 2.3 - Finding the Inverse of a Matrix In Exercises 7-30,...Ch. 2.3 - Finding the Inverse of a Matrix In Exercises 7-30,...Ch. 2.3 - Finding the Inverse of a Matrix In Exercises 7-30,...Ch. 2.3 - Prob. 24ECh. 2.3 - Prob. 25ECh. 2.3 - Prob. 26ECh. 2.3 - Prob. 27ECh. 2.3 - Finding the Inverse of a Matrix In Exercises 7-30,...Ch. 2.3 - Prob. 29ECh. 2.3 - Prob. 30ECh. 2.3 - Finding the Inverse of a 22 Matrix In Exercises...Ch. 2.3 - Finding the Inverse of a 22 Matrix In Exercises...Ch. 2.3 - Prob. 33ECh. 2.3 - Finding the Inverse of a 22 Matrix In Exercises...Ch. 2.3 - Finding the Inverse of a 22 Matrix In Exercises...Ch. 2.3 - Finding the Inverse of a 22 Matrix In Exercises...Ch. 2.3 - Prob. 37ECh. 2.3 - Prob. 38ECh. 2.3 - Finding the Inverse of the Square of a Matrix In...Ch. 2.3 - Finding the Inverse of the Square of a Matrix In...Ch. 2.3 - Finding the Inverses of Products and Transposes In...Ch. 2.3 - Finding the Inverses of Products and Transposes In...Ch. 2.3 - Finding the Inverses of Products and Transposes In...Ch. 2.3 - Prob. 44ECh. 2.3 - Solving a System of Equations Using an Inverse In...Ch. 2.3 - Prob. 46ECh. 2.3 - Solving a System of Equations Using an Inverse In...Ch. 2.3 - Solving a System of Equations Using an Inverse In...Ch. 2.3 - Prob. 49ECh. 2.3 - Prob. 50ECh. 2.3 - Prob. 51ECh. 2.3 - Prob. 52ECh. 2.3 - Matrix Equal to Its Own Inverse In Exercises 53...Ch. 2.3 - Matrix Equal to Its Own Inverse In Exercises 53...Ch. 2.3 - Singular Matrix In Exercises 55 and 56, find x...Ch. 2.3 - Singular Matrix In Exercises 55 and 56, find x...Ch. 2.3 - Prob. 57ECh. 2.3 - Prob. 58ECh. 2.3 - Prob. 59ECh. 2.3 - Prob. 60ECh. 2.3 - Prob. 61ECh. 2.3 - Prob. 62ECh. 2.3 - Prob. 63ECh. 2.3 - Prob. 64ECh. 2.3 - Prob. 65ECh. 2.3 - Proof Prove that if A2=A, then I-2A=I-2A-1.Ch. 2.3 - Guided Proof Prove that the inverse of a symmetric...Ch. 2.3 - Prob. 68ECh. 2.3 - Prob. 69ECh. 2.3 - Prob. 70ECh. 2.3 - Prob. 71ECh. 2.3 - True or False ? In Exercises 71 and 72, determine...Ch. 2.3 - Prob. 73ECh. 2.3 - Prob. 74ECh. 2.3 - Prob. 75ECh. 2.3 - Prob. 76ECh. 2.3 - Proof Let u be an n1 column matrix satisfying...Ch. 2.3 - Prob. 78ECh. 2.3 - Let A,D, and P be nn matrices satisfying AP=PD....Ch. 2.3 - Prob. 80ECh. 2.3 - Prob. 81ECh. 2.3 - Prob. 82ECh. 2.3 - Prob. 83ECh. 2.4 - Elementary Matrices In Exercises 1-8, determine...Ch. 2.4 - Elementary Matrices In Exercises 1-8, determine...Ch. 2.4 - Prob. 3ECh. 2.4 - Prob. 4ECh. 2.4 - Elementary Matrices In Exercises 1-8, determine...Ch. 2.4 - Elementary Matrices In Exercises 1-8, determine...Ch. 2.4 - Elementary Matrices In Exercises 1-8, determine...Ch. 2.4 - Elementary Matrices In Exercises 1-8, determine...Ch. 2.4 - Finding an Elementary Matrix In Exercises 9-12,...Ch. 2.4 - Finding an Elementary Matrix In Exercises 9-12,...Ch. 2.4 - Finding an Elementary Matrix In Exercises 9-12,...Ch. 2.4 - Finding an Elementary Matrix In Exercises 9-12,...Ch. 2.4 - Finding a Sequence of Elementary Matrices In...Ch. 2.4 - Finding a Sequence of Elementary Matrices In...Ch. 2.4 - Finding a Sequence of Elementary Matrices In...Ch. 2.4 - Finding a Sequence of Elementary Matrices In...Ch. 2.4 - Finding a Sequence of Elementary Matrices In...Ch. 2.4 - Finding a Sequence of Elementary Matrices In...Ch. 2.4 - Finding the Inverse of an Elementary Matrix In...Ch. 2.4 - Finding the Inverse of an Elementary Matrix In...Ch. 2.4 - Finding the Inverse of an Elementary Matrix In...Ch. 2.4 - Finding the Inverse of an Elementary Matrix In...Ch. 2.4 - Finding the Inverse of an Elementary Matrix In...Ch. 2.4 - Finding the Inverse of an Elementary Matrix In...Ch. 2.4 - Finding the Inverse of a Matrix In Exercises...Ch. 2.4 - Finding the Inverse of a Matrix In Exercises...Ch. 2.4 - Finding the Inverse of a Matrix In Exercises...Ch. 2.4 - Finding the Inverse of a Matrix In Exercises...Ch. 2.4 - Finding a Sequence of Elementary Matrices In...Ch. 2.4 - Finding a Sequence of Elementary Matrices In...Ch. 2.4 - Finding a Sequence of Elementary Matrices In...Ch. 2.4 - Finding a Sequence of Elementary Matrices In...Ch. 2.4 - Finding a Sequence of Elementary Matrices In...Ch. 2.4 - Finding a Sequence of Elementary Matrices In...Ch. 2.4 - Finding a Sequence of Elementary Matrices In...Ch. 2.4 - Prob. 36ECh. 2.4 - Writing Is the product of two elementary matrices...Ch. 2.4 - Prob. 38ECh. 2.4 - Use elementary matrices to find the inverse of...Ch. 2.4 - Use elementary matrices to find the inverse of...Ch. 2.4 - True or False? In Exercises 41 and 42, determine...Ch. 2.4 - True or False? In Exercises 41 and 42, determine...Ch. 2.4 - Finding an LU-Factorization of a Matrix In...Ch. 2.4 - Prob. 44ECh. 2.4 - Finding an LU-Factorization of a Matrix In...Ch. 2.4 - Finding an LU-Factorization of a Matrix In...Ch. 2.4 - Solving a Linear System Using LU-Factorization In...Ch. 2.4 - Prob. 48ECh. 2.4 - Idempotent Matrices In Exercises 49-52, determine...Ch. 2.4 - Idempotent Matrices In Exercises 49-52, determine...Ch. 2.4 - Idempotent Matrices In Exercises 49-52, determine...Ch. 2.4 - Idempotent Matrices In Exercises 49-52, determine...Ch. 2.4 - Prob. 53ECh. 2.4 - Guided Proof Prove that A is idempotent if and...Ch. 2.4 - Proof Prove that if A is an nn matrix that is...Ch. 2.4 - Proof Prove that if A and B are idempotent and...Ch. 2.4 - Guided Proof Prove that if A is row-equivalent to...Ch. 2.4 - Proof Prove that if A is row-equivalent to B, then...Ch. 2.4 - Proof Let A be a nonsingular matrix. Prove that if...Ch. 2.4 - CAPSTONE a Explain how to find an elementary...Ch. 2.4 - Show that the matrix below does not have an LU...Ch. 2.5 - Stochastic Matrices In Exercise 1-6, determine...Ch. 2.5 - Prob. 2ECh. 2.5 - Prob. 3ECh. 2.5 - Stochastic Matrices In Exercise 1-6, determine...Ch. 2.5 - Prob. 5ECh. 2.5 - Prob. 6ECh. 2.5 - Airplane Allocation An airline has 30 airplane in...Ch. 2.5 - Chemistry In a chemistry experiment, a test tube...Ch. 2.5 - Finding State Matrices In exercises 9 and 10, use...Ch. 2.5 - Finding State Matrices In exercises 9 and 10, use...Ch. 2.5 - Purchase of a product The market research...Ch. 2.5 - Spread of a Virus A medical researcher is studying...Ch. 2.5 - Prob. 13ECh. 2.5 - Prob. 14ECh. 2.5 - Smokers and Non smokers In a population of 10,000,...Ch. 2.5 - Consumer Preference In a population of 100,000...Ch. 2.5 - Regular and Steady State Matrices In Exercises...Ch. 2.5 - Prob. 18ECh. 2.5 - Regular and Steady State Matrices In Exercises...Ch. 2.5 - Prob. 20ECh. 2.5 - Regular and Steady State Matrices In Exercises...Ch. 2.5 - Regular and Steady State Matrices In Exercises...Ch. 2.5 - Prob. 23ECh. 2.5 - Prob. 24ECh. 2.5 - Regular and Steady State Matrices In Exercises...Ch. 2.5 - Prob. 26ECh. 2.5 - Prob. 27ECh. 2.5 - Prob. 28ECh. 2.5 - Regular and Steady State Matrices In Exercises...Ch. 2.5 - Prob. 30ECh. 2.5 - Prob. 31ECh. 2.5 - Find the steady state matrix for each stochastic...Ch. 2.5 - Prob. 33ECh. 2.5 - Prob. 34ECh. 2.5 - Stock Sales and Purchases Eight hundred fifty...Ch. 2.5 - Customer Preference Two movie theatres that show...Ch. 2.5 - Absorbing Markov Chains In Exercises 3740,...Ch. 2.5 - Absorbing Markov Chains In Exercises 3740,...Ch. 2.5 - Absorbing Markov Chains In Exercises 3740,...Ch. 2.5 - Prob. 40ECh. 2.5 - Prob. 41ECh. 2.5 - Prob. 42ECh. 2.5 - Prob. 43ECh. 2.5 - Prob. 44ECh. 2.5 - Epidemic Model In a population of 200,000 people,...Ch. 2.5 - Chess Tournament Two people are engaged in a chess...Ch. 2.5 - Explain how you can determine the steady state...Ch. 2.5 - CAPSTONE Explain how to find the nth state matrix...Ch. 2.5 - Consider the Markov chain whose matrix of...Ch. 2.5 - Markov Chain with Reflecting Boundaries The figure...Ch. 2.5 - Prob. 51ECh. 2.5 - Prob. 52ECh. 2.5 - Prob. 53ECh. 2.5 - Prob. 54ECh. 2.5 - Prob. 55ECh. 2.5 - Proof Prove that when P is a regular stochastic...Ch. 2.6 - Encoding a Message In Exercises 1 and 2, write the...Ch. 2.6 - Prob. 2ECh. 2.6 - Prob. 3ECh. 2.6 - Decoding a Message In Exercises 3-6, use A-1to...Ch. 2.6 - Prob. 5ECh. 2.6 - Prob. 6ECh. 2.6 - Prob. 7ECh. 2.6 - Prob. 8ECh. 2.6 - Decoding a Message Use a software or a graphing...Ch. 2.6 - Decoding a Message A code breaker intercepted the...Ch. 2.6 - Prob. 11ECh. 2.6 - Prob. 12ECh. 2.6 - Solving for the Output Matrix A small community...Ch. 2.6 - Prob. 14ECh. 2.6 - Prob. 15ECh. 2.6 - Prob. 16ECh. 2.6 - Prob. 17ECh. 2.6 - Prob. 18ECh. 2.6 - Prob. 19ECh. 2.6 - Prob. 20ECh. 2.6 - Prob. 21ECh. 2.6 - Prob. 22ECh. 2.6 - Prob. 23ECh. 2.6 - Prob. 24ECh. 2.6 - Prob. 25ECh. 2.6 - Prob. 26ECh. 2.6 - Demand A hardware retailer wants to know the...Ch. 2.6 - Prob. 28ECh. 2.6 - Prob. 29ECh. 2.6 - Prob. 30ECh. 2.6 - Prob. 31ECh. 2.CR - Prob. 1CRCh. 2.CR - Operation with Matrices In Exercise 1-6, perform...Ch. 2.CR - Prob. 3CRCh. 2.CR - Prob. 4CRCh. 2.CR - Prob. 5CRCh. 2.CR - Prob. 6CRCh. 2.CR - Prob. 7CRCh. 2.CR - Prob. 8CRCh. 2.CR - Prob. 9CRCh. 2.CR - Prob. 10CRCh. 2.CR - Prob. 11CRCh. 2.CR - Prob. 12CRCh. 2.CR - Prob. 13CRCh. 2.CR - Prob. 14CRCh. 2.CR - Prob. 15CRCh. 2.CR - Prob. 16CRCh. 2.CR - Prob. 17CRCh. 2.CR - Prob. 18CRCh. 2.CR - Prob. 19CRCh. 2.CR - Prob. 20CRCh. 2.CR - Prob. 21CRCh. 2.CR - Prob. 22CRCh. 2.CR - Prob. 23CRCh. 2.CR - Prob. 24CRCh. 2.CR - Prob. 25CRCh. 2.CR - Prob. 26CRCh. 2.CR - Prob. 27CRCh. 2.CR - Prob. 28CRCh. 2.CR - Nonsingular Matrix In Exercises 29 and 30, find x...Ch. 2.CR - Nonsingular Matrix In Exercises 29 and 30, find x...Ch. 2.CR - Prob. 31CRCh. 2.CR - Prob. 32CRCh. 2.CR - Finding a Sequence of Elementary Matrix In...Ch. 2.CR - Prob. 34CRCh. 2.CR - Prob. 35CRCh. 2.CR - Finding a Sequence of Elementary Matrix In...Ch. 2.CR - Prob. 37CRCh. 2.CR - Prob. 38CRCh. 2.CR - Prob. 39CRCh. 2.CR - Prob. 40CRCh. 2.CR - Consider the matrices below....Ch. 2.CR - Prob. 42CRCh. 2.CR - Prob. 43CRCh. 2.CR - Prob. 44CRCh. 2.CR - Prob. 45CRCh. 2.CR - Prob. 46CRCh. 2.CR - Solving a Linear System Using LU-Factorization In...Ch. 2.CR - Prob. 48CRCh. 2.CR - Manufacturing A company manufactures tables and...Ch. 2.CR - Prob. 50CRCh. 2.CR - Gasoline Sales Matrix A shows the numbers of...Ch. 2.CR - Prob. 52CRCh. 2.CR - Prob. 53CRCh. 2.CR - Prob. 54CRCh. 2.CR - Prob. 55CRCh. 2.CR - Prob. 56CRCh. 2.CR - Prob. 57CRCh. 2.CR - Prob. 58CRCh. 2.CR - Finding State Matrices In Exercises 5962, use the...Ch. 2.CR - Prob. 60CRCh. 2.CR - Prob. 61CRCh. 2.CR - Prob. 62CRCh. 2.CR - Prob. 63CRCh. 2.CR - Prob. 64CRCh. 2.CR - Prob. 65CRCh. 2.CR - Regular and Steady State Matrix In Exercises 6568,...Ch. 2.CR - Regular and Steady State Matrix In Exercises 6568,...Ch. 2.CR - Prob. 68CRCh. 2.CR - Prob. 69CRCh. 2.CR - Classified Documents A courtroom has 2000...Ch. 2.CR - Prob. 71CRCh. 2.CR - Prob. 72CRCh. 2.CR - True or False? In Exercises 7376, determine...Ch. 2.CR - Prob. 74CRCh. 2.CR - Prob. 75CRCh. 2.CR - Prob. 76CRCh. 2.CR - Encoding a Message In Exercises 77and 78, write...Ch. 2.CR - Encoding a Message In Exercises 77and 78, write...Ch. 2.CR - Decoding a Message In Exercises 79-82, use A1 to...Ch. 2.CR - Decoding a Message In Exercises 79-82, use A1 to...Ch. 2.CR - Decoding a Message In Exercises 79-82, use A1 to...Ch. 2.CR - Prob. 82CRCh. 2.CR - Industrial System An industrial system has two...Ch. 2.CR - Prob. 84CRCh. 2.CR - Prob. 85CRCh. 2.CR - Prob. 86CRCh. 2.CR - Prob. 87CRCh. 2.CR - Prob. 88CRCh. 2.CR - Cellular Phone Subscribers The table shows the...Ch. 2.CR - Prob. 90CR
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.Similar questions
- How much is the circumference of a circle whose diameter is 7 feet?C =π darrow_forwardHow to solve 2542/64.132arrow_forwardAssume that you fancy polynomial splines, while you actually need ƒ(t) = e²/3 – 1 for t€ [−1, 1]. See the figure for a plot of f(t). Your goal is to approximate f(t) with an inter- polating polynomial spline of degree d that is given as sa(t) = • Σk=0 Pd,k bd,k(t) so that sd(tk) = = Pd,k for tk = −1 + 2 (given d > 0) with basis functions bd,k(t) = Σi±0 Cd,k,i = • The special case of d 0 is trivial: the only basis function b0,0 (t) is constant 1 and so(t) is thus constant po,0 for all t = [−1, 1]. ...9 The d+1 basis functions bd,k (t) form a ba- sis Bd {ba,o(t), ba,1(t), bd,d(t)} of the function space of all possible sα (t) functions. Clearly, you wish to find out, which of them given a particular maximal degree d is the best-possible approximation of f(t) in the least- squares sense. _ 1 0.9 0.8 0.7 0.6 0.5 0.4 0.3 0.2 0.1 0 -0.1 -0.2 -0.3 -0.4 -0.5 -0.6 -0.7 -0.8 -0.9 -1 function f(t) = exp((2t)/3) - 1 to project -1 -0.9 -0.8 -0.7 -0.6 -0.5 -0.4 -0.3 -0.2 -0.1 0 0.1 0.2 0.3 0.4 0.5…arrow_forward
- An image processor considered a 750×750 pixels large subset of an image and converted it into gray-scale, resulting in matrix gIn - a false-color visualization of gIn is shown in the top-left below. He prepared a two-dim. box filter f1 as a 25×25 matrix with only the 5×5 values in the middle being non-zero – this filter is shown in the top-middle position below. He then convolved £1 with itself to get £2, before convolving £2 with itself to get f3. In both of the steps, he maintained the 25×25 size. Next, he convolved gIn with £3 to get gl. Which of the six panels below shows g1? Argue by explaining all the steps, so far: What did the image processor do when preparing ₤3? What image processing operation (from gin to g1) did he prepare and what's the effect that can be seen? Next, he convolved the rows of f3 with filter 1/2 (-1, 8, 0, -8, 1) to get f4 - you find a visualization of filter f 4 below. He then convolved gIn with f4 to get g2 and you can find the result shown below. What…arrow_forward3ur Colors are enchanting and elusive. A multitude of color systems has been proposed over a three-digits number of years - maybe more than the number of purposes that they serve... - Everyone knows the additive RGB color system – we usually serve light-emitting IT components like monitors with colors in that system. Here, we use c = (r, g, b) RGB with r, g, bЄ [0,1] to describe a color c. = T For printing, however, we usually use the subtractive CMY color system. The same color c becomes c = (c, m, y) CMY (1-c, 1-m, 1-y) RGB Note how we use subscripts to indicate with coordinate system the coordinates correspond to. Explain, why it is not possible to find a linear transformation between RGB and CMY coordinates. Farbenlehr c von Goethe Erster Band. Roſt einen Defte mit fergen up Tübingen, is et 3. Cotta'fden Babarblung. ISIO Homogeneous coordinates give us a work-around: If we specify colors in 4D, instead, with the 4th coordinate being the homogeneous coordinate h so that every actual…arrow_forwardCan someone provide an answer & detailed explanation please? Thank you kindly!arrow_forward
- Given the cubic function f(x) = x^3-6x^2 + 11x- 6, do the following: Plot the graph of the function. Find the critical points and determine whether each is a local minimum, local maximum, or a saddle point. Find the inflection point(s) (if any).Identify the intervals where the function is increasing and decreasing. Determine the end behavior of the graph.arrow_forwardGiven the quadratic function f(x) = x^2-4x+3, plot the graph of the function and find the following: The vertex of the parabola .The x-intercepts (if any). The y-intercept. Create graph also before solve.arrow_forwardwhat model best fits this dataarrow_forward
- Round as specified A) 257 down to the nearest 10’s place B) 650 to the nearest even hundreds, place C) 593 to the nearest 10’s place D) 4157 to the nearest hundreds, place E) 7126 to the nearest thousand place arrow_forwardEstimate the following products in two different ways and explain each method  A) 52x39 B) 17x74 C) 88x11 D) 26x42arrow_forwardFind a range estimate for these problems A) 57x1924 B) 1349x45 C) 547x73951arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
 Elementary Linear Algebra (MindTap Course List)AlgebraISBN:9781305658004Author:Ron LarsonPublisher:Cengage Learning
Elementary Linear Algebra (MindTap Course List)AlgebraISBN:9781305658004Author:Ron LarsonPublisher:Cengage Learning

 Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt

Elementary Linear Algebra (MindTap Course List)
Algebra
ISBN:9781305658004
Author:Ron Larson
Publisher:Cengage Learning



Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning

Big Ideas Math A Bridge To Success Algebra 1: Stu...
Algebra
ISBN:9781680331141
Author:HOUGHTON MIFFLIN HARCOURT
Publisher:Houghton Mifflin Harcourt
Correlation Vs Regression: Difference Between them with definition & Comparison Chart; Author: Key Differences;https://www.youtube.com/watch?v=Ou2QGSJVd0U;License: Standard YouTube License, CC-BY
Correlation and Regression: Concepts with Illustrative examples; Author: LEARN & APPLY : Lean and Six Sigma;https://www.youtube.com/watch?v=xTpHD5WLuoA;License: Standard YouTube License, CC-BY