
Concept explainers
a.
To find:The relative frequency histogram for the data.
a.
Answer to Problem 2.34E
The relative frequency histogram for the datais shown in Figure-1.
Explanation of Solution
Given information: The data is,
| Washington | 0 | Van Buren | 4 | Buchanan | 0 |
| Adams | 5 | W.H. Harrison | 10 | Lincoln | 4 |
| Jefferson | 6 | Tyler* | 15 | A. Johnson | 5 |
| Madison | 0 | Polk | 0 | Grant | 4 |
| Monroe | 2 | Taylor | 6 | Hayes | 8 |
| JO. Adams | 4 | Fillmore* | 2 | Garfield | 7 |
| Jackson | 0 | Pierce | 3 | Arthur | 3 |
| Cleveland | 5 | Coolidge | 2 | Nixon | 2 |
| B. Harrison* | 3 | Hoover | 2 | Ford | 4 |
| McKinley | 2 | F.D.Roosevelt | 6 | Carter | 4 |
| T. Roosevelt* | 6 | Truman | 1 | Reagan* | 4 |
| Taft | 3 | Eisenhower | 2 | G.H.W. Bush | 6 |
| Wilson* | 3 | Kennedy | 3 | Clinton | 1 |
| Harding | 0 | L.B.Johmson | 2 | G.W. Bush | 2 |
| Obama | 2 |
Calculation:
The histogram is shown below.
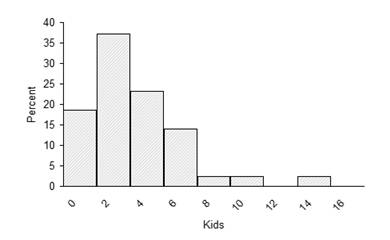
Figure-1
Thus, the relative frequency histogram for the data is shown in Figure-1.
b.
To find: The mean and standard deviation.
b.
Answer to Problem 2.34E
The mean and standard deviation is
Explanation of Solution
Given information: The data is,
| Washington | 0 | Van Buren | 4 | Buchanan | 0 |
| Adams | 5 | W.H. Harrison | 10 | Lincoln | 4 |
| Jefferson | 6 | Tyler* | 15 | A. Johnson | 5 |
| Madison | 0 | Polk | 0 | Grant | 4 |
| Monroe | 2 | Taylor | 6 | Hayes | 8 |
| JO. Adams | 4 | Fillmore* | 2 | Garfield | 7 |
| Jackson | 0 | Pierce | 3 | Arthur | 3 |
| Cleveland | 5 | Coolidge | 2 | Nixon | 2 |
| B. Harrison* | 3 | Hoover | 2 | Ford | 4 |
| McKinley | 2 | F.D.Roosevelt | 6 | Carter | 4 |
| T. Roosevelt* | 6 | Truman | 1 | Reagan* | 4 |
| Taft | 3 | Eisenhower | 2 | G.H.W. Bush | 6 |
| Wilson* | 3 | Kennedy | 3 | Clinton | 1 |
| Harding | 0 | L.B.Johmson | 2 | G.W. Bush | 2 |
| Obama | 2 |
Calculation:
The mean is,
The variance is,
The standard deviation is,
Thus, the mean and standard deviation is
c.
To find: The percentage falling into the given intervals.
c.
Answer to Problem 2.34E
The percentage falling into the given intervalsis
Explanation of Solution
Given information: The data is,
| Washington | 0 | Van Buren | 4 | Buchanan | 0 |
| Adams | 5 | W.H. Harrison | 10 | Lincoln | 4 |
| Jefferson | 6 | Tyler* | 15 | A. Johnson | 5 |
| Madison | 0 | Polk | 0 | Grant | 4 |
| Monroe | 2 | Taylor | 6 | Hayes | 8 |
| JO. Adams | 4 | Fillmore* | 2 | Garfield | 7 |
| Jackson | 0 | Pierce | 3 | Arthur | 3 |
| Cleveland | 5 | Coolidge | 2 | Nixon | 2 |
| B. Harrison* | 3 | Hoover | 2 | Ford | 4 |
| McKinley | 2 | F.D.Roosevelt | 6 | Carter | 4 |
| T. Roosevelt* | 6 | Truman | 1 | Reagan* | 4 |
| Taft | 3 | Eisenhower | 2 | G.H.W. Bush | 6 |
| Wilson* | 3 | Kennedy | 3 | Clinton | 1 |
| Harding | 0 | L.B.Johmson | 2 | G.W. Bush | 2 |
| Obama | 2 |
Calculation:
The interval is,
The measurement falling into the intervals are 33.
The interval is,
The measurement falling into the intervals are 41.
The interval is,
The measurement falling into the intervals are 42.
According to the Empirical formula the percentage of values falling into the intervals are
Thus, the percentage falling into the given intervals is
Want to see more full solutions like this?
Chapter 2 Solutions
Introduction to Probability and Statistics