Bundle: Physics For Scientists And Engineers With Modern Physics, Loose-leaf Version, 10th + Webassign Printed Access Card For Serway/jewett's Physics For Scientists And Engineers, 10th, Single-term
10th Edition
ISBN: 9781337888585
Author: Raymond A. Serway, John W. Jewett
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 24, Problem 28P
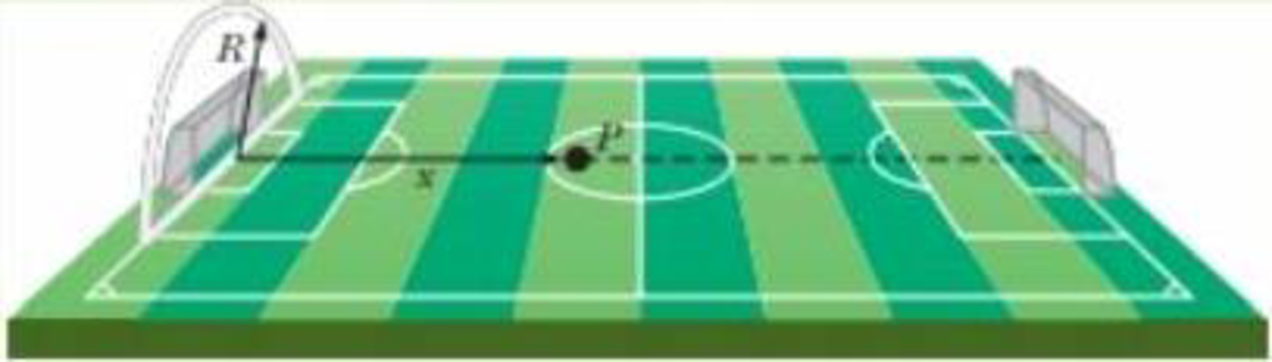
You are a coach for the Physics Olympics team participating in a competition overseas. You are given the following sample problem to present to your team of students, which you need to solve very quickly: A person is standing on the midline of a soccer field. At one end of the field, as shown in Figure P24.28, is a letter D, consisting of a semicircular metallic ring of radius R and a long straight metal rod of length 2R, the diameter of the ring. The plane of the ring is perpendicular to the ground and perpendicular to the midline of the field shown by the broken line in Figure P24.28. Because of an approaching lightning storm, the semicircular ring and the rod become charged. The ring and the rod each attain a charge Q. What is the electric potential at point P, which is at a position x along the midline of the field, measured from the center of the rod, due to the letter D? Think quickly and use all resources available to you, which include your physics textbook: yon are in competition!
Figure P24.28
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
Select the best answer, explaining the reason to justify your choice.
A simple and common technique for accelerating electrons is shown in the figure, which depicts a uniform electric field between two plates. Electrons are released, usually from a hot filament, near the negative plate, and there is a small hole in the positive plate that allows the electrons to pass through.
Randomized Variables E = 2.7 × 104 N/C
Calculate the horizontal component of the electron's acceleration if the field strength is 2.7 × 104 N/C. Express your answer in meters per second squared, and assume the electric field is pointing in the negative x-direction as shown in the figure.
In the Rutherford model of the hydrogen atom, a proton (mass M, charge Q) is the nucleus and an electron (mass m, charge q) moves
around the proton in a circle of radius r. Let k denote the Coulomb force constant (1/4TTE0) and G the universal gravitational constant.
The ratio of the electrostatic force to the gravitational force between electron and proton is:
O kMm/GQq
O kQq/GMm
O GQq/kMm
O GMm/kQq
O kQq/GMmr²
Chapter 24 Solutions
Bundle: Physics For Scientists And Engineers With Modern Physics, Loose-leaf Version, 10th + Webassign Printed Access Card For Serway/jewett's Physics For Scientists And Engineers, 10th, Single-term
Ch. 24.1 - two points and are located within a region in...Ch. 24.2 - QUICK QUIZ 24.2 The labeled points in Figure 24.4...Ch. 24.3 - In Figure 24.8b, take q2, to be a negative source...Ch. 24.4 - In a certain region of space, the electric...Ch. 24 - How much work is done (by a battery, generator, or...Ch. 24 - (a) Find the electric potential difference Ve...Ch. 24 - Oppositely charged parallel plates are separated...Ch. 24 - Starting with the definition of work, prove that...Ch. 24 - An insulating rod having linear charge density =...Ch. 24 - Review. A block having mass m and charge + Q is...
Ch. 24 - Three positive charges are located at the corners...Ch. 24 - Two point charges Q1 = +5.00 nC and Q2 = 3.00 nC...Ch. 24 - You are working on a laboratory device that...Ch. 24 - Your roommate is having trouble understanding why...Ch. 24 - Four point charges each having charge Q are...Ch. 24 - The two charges in Figure P24.12 are separated by...Ch. 24 - Show that the amount of work required to assemble...Ch. 24 - Two charged particles of equal magnitude are...Ch. 24 - Three particles with equal positive charges q are...Ch. 24 - Prob. 16PCh. 24 - Prob. 17PCh. 24 - Prob. 18PCh. 24 - How much work is required to assemble eight...Ch. 24 - Four identical particles, each having charge q and...Ch. 24 - It is shown in Example 24.7 that the potential at...Ch. 24 - Figure P24.22 represents a graph of the electric...Ch. 24 - Figure P24.23 shows several equipotential lines,...Ch. 24 - An electric field in a region of space is parallel...Ch. 24 - A rod of length L (Fig. P24.25) lies along the x...Ch. 24 - For the arrangement described in Problem 25,...Ch. 24 - A wire having a uniform linear charge density is...Ch. 24 - You are a coach for the Physics Olympics team...Ch. 24 - The electric field magnitude on the surface of an...Ch. 24 - Why is the following situation impossible? A solid...Ch. 24 - A solid metallic sphere of radius a carries total...Ch. 24 - Prob. 32PCh. 24 - A very large, thin, flat plate of aluminum of area...Ch. 24 - Prob. 34PCh. 24 - Prob. 35PCh. 24 - A long, straight wire is surrounded by a hollow...Ch. 24 - Prob. 37APCh. 24 - Prob. 38APCh. 24 - Prob. 39APCh. 24 - Why is the following situation impossible? You set...Ch. 24 - The thin, uniformly charged rod shown in Figure...Ch. 24 - A GeigerMueller tube is a radiation detector that...Ch. 24 - Review. Two parallel plates having charges of...Ch. 24 - When an uncharged conducting sphere of radius a is...Ch. 24 - A solid, insulating sphere of radius a has a...Ch. 24 - Prob. 46APCh. 24 - For the configuration shown in Figure P24.45,...Ch. 24 - An electric dipole is located along the y axis as...Ch. 24 - A disk of radius R (Fig. P24.49) has a nonuniform...Ch. 24 - Prob. 50CPCh. 24 - (a) A uniformly charged cylindrical shell with no...
Additional Science Textbook Solutions
Find more solutions based on key concepts
8. The formula ΔU = nCvΔT for the change in the internal energy of a fixed amount of an ideal gas is valid
only...
College Physics (10th Edition)
Is Earths inner core solid and the outer core liquid because the inner core is cooler than the outer core? Expl...
Conceptual Integrated Science
Choose the best answer to each of the following. Explain your reasoning. If Earth were twice as far as it actua...
The Cosmic Perspective Fundamentals (2nd Edition)
7. (II) (a) What is the current in the element of an electric clothes dryer with a resistance of 8.6 ?when it i...
Physics: Principles with Applications
The Rankine temperature scale (abbreviatedR) uses the same size degrees as Fahrenheit, but measured up from abs...
An Introduction to Thermal Physics
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- In preparation for an experiment that you will do in your introductory nuclear physics lab, you are shown the inside of a Geiger tube. You measure the radius and the length of the central wire of the Geiger tube to be 2.0 x 10-4 m and 1.2 x 10-1 m, respectively. The outer surface of the tube is a conducting cylindrical shell that has an inner radius of 1.5 x 10-2 m. The shell is coaxial with the wire and has the same length (0.12 m). (a) Calculate the capacitance of your tube, assuming that the gas in the tube has a dielectric constant of 1.00. F (b) Calculate the value of the linear charge density on the wire when the potential difference between the wire and shell is of 1.20 kV. C/marrow_forwardA long cylinder of charge q has a radius a. The charge density within its volume, p, is uniform (Figure). Describe the form of the electric field generated by the cylinder. Find the electric field strength at a distance r from the axis of the cylinder in the regions (i) r > a and (ii) 0 <r<a.If a non-relativistic electron moves in a circle at a constant distance R from the axis of the cylinder, where R > a, find an expression for its speed.arrow_forwardAn electron has an initial velocity of 2.4x106 m/s in the +x direction. It enters a uniform electric field E = 397 N/C which is in the +y direction. What is the ratio of the y-component of the velocity of the electron to the x-component of the velocity after traveling 5 cm in the +x direction in the field? (Your result must include 2 digit after the decimal point and maximum of 5% of error is accepted in your answer. Take elementary charge 1.6x10-19 C and take mass of electron 9.1x10-31 kg.)arrow_forward
- Suppose a capacitor consists of two coaxial thin cylindrical conductors. The inner cylinder of radius ra has a charge of +Q, while the outer cylinder of radius rp has charge -Q. The electric field E at a radial distance r from the central axis is given by the function: E = ae-T/ao + B/r + bo where alpha (a), beta (8), ao and bo are constants. Find an expression for its capacitance. First, let us derive the potential difference Vah between the two conductors. The potential difference is related to the electric field by: Vab = Edr = - Edr Calculating the antiderivative or indefinite integral, Vab = (-aage-r/a0 + B + bo By definition, the capacitance Cis related to the charge and potential difference by: C = Evaluating with the upper and lower limits of integration for Vab, then simplifying: C= Q/( (e-rb/ao - eTalao) + B In( ) + bo ( ))arrow_forwardA simple and common technique for accelerating electrons is shown in the figure, which depicts a uniform electric field between two plates. Electrons are released, usually from a hot filament, near the negative plate, and there is a small hole in the positive plate that allows the electrons to pass through.Randomized VariablesE = 2.7 × 104 N/C Calculate the horizontal component of the electron's acceleration if the field strength is 2.7 × 104 N/C. Express your answer in meters per second squared, and assume the electric field is pointing in the negative x-direction as shown in the figure.arrow_forwardInside a vacuum tube, an electron is projected between two parallel Problem 1 plates. The plates are separated by 3 cm and are 10 cm long. Equal but opposite electric charges are placed on the plates, so that there is a uniform electric field between the plates and no field outside of the plates. The electron is projected in the center of the positive plate and its initial velocity is 4.5 x 10 m/s straight towards the center of the negative plate. Find the magnitude of the electric field such that the electron just barely misses touching the negative plate before it turns around goes directly back out of the hole. 10cm. Зстarrow_forward
- electrons Two protons (p) and two (e) are arranged on a circle of 5 [cm], with angles 0₁ = 20°, 0₂ = 60°, 03 = 20° and 04 = 60°, as radius r shown in the figure. (qp = +1.6 x 10-1⁹ [C] and qe = -1.6 × 10-19 [C]). The figure is not to scale. 04 y a. Find Enet, the net electric field vector produced at the center of the circle. Enet =([ ])i + ( ]) Ĵ [N/C] b. Where on the circle should a fifth point charge qo be placed (give its angle relative the +x-axis) and what is its value (calculate qo) in order to have Ēnet (the net electric field at the center of the circle) equals zero (Type the detailed solution to this question in the below box, Show all your calculation steps by typing in the box). Р Xarrow_forwardTwo conductors with concentric radii r1=5 m and r2=23 m are placed in such a way that their center is the origin. While there is a charge Q1=7,1 µC on the circle with radius r1, there is a charge Q2=7,1 µC Accordingly, the electric field at point A(18, 162°, 52°) is given below. Find the amplitude of the electric field. TT=3,1416 E= 36п 10-9 Q2 r2 Q1 A Xarrow_forwardA uranium ion and an iron ion are separated by a distance of ?=60.30 nm.R=60.30 nm. The uranium atom is singly ionized; the iron atom is doubly ionized. Calculate the distance ? from the uranium atom at which an electron will be in equilibrium. Ignore the gravitational attraction between the particles. What is the magnitude of the force on the electron from the uranium ion?arrow_forward
- Suppose a capacitor consists of two coaxial thin cylindrical conductors. The inner cylinder of radius rg has a charge of +Q, while the outer cylinder of radius rh has charge -Q. The electric field E at a radial distance r from the central axis is given by the function: E = ae-r/a0 + B/r + bo where alpha (a), beta (B), ao and bo are constants. Find an expression for its capacitance. First, let us derive the potential difference Vab between the two conductors. The potential difference is related to the electric field by: Vab = [ * Edr = - Edr Calculating the antiderivative or indefinite integral, Vab = (-aage-r/ao + B + bo By definition, the capacitance C is related to the charge and potential difference by: C = Q Evaluating with the upper and lower limits of integration for Vab, then simplifying: C = Q / ( (erb/a0 - eTa/a0) + B In( )+ bo ( ))arrow_forwardTwo conductors with concentric radii r1=4 m and r2=24 m are placed in such a way that their center is the origin. While there is a charge Q1=1 µC on the circle with radius r1, there is a charge Q2=1,3 µC Accordingly, the electric field at point A(15, 74°, 22°) is given below. Find the amplitude of the electric field. TI=3,1416 1 10-9 E= 36n Q2 r2 Q1 r1 •A Yanıt: Seçiniz. +arrow_forwardA proton is fired from far away towards the nucleus of a mercury atom. Mercury is element number 80, and the diameter of the nucleus is 14.0 fm. If the proton is fired at a speed of 13600000 m/s, what is its closest approach to the surface of the nucleus (in fm)? Assume that the nucleus remains at rest.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...
Physics
ISBN:9781133939146
Author:Katz, Debora M.
Publisher:Cengage Learning
Principles of Physics: A Calculus-Based Text
Physics
ISBN:9781133104261
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...
Physics
ISBN:9781337553292
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Electric Fields: Crash Course Physics #26; Author: CrashCourse;https://www.youtube.com/watch?v=mdulzEfQXDE;License: Standard YouTube License, CC-BY