Physics for Scientists and Engineers
10th Edition
ISBN: 9781337553278
Author: Raymond A. Serway, John W. Jewett
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 24, Problem 10P
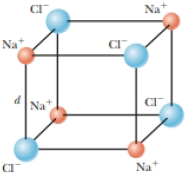
Your roommate is having trouble understanding why solids form. He asks, “Why would atoms bond into solids rather than just floating freely with respect to each other?” To help him understand at least one type of bonding in solids, you decide to embark on an energy explanation. You show him a drawing of a primitive cell of a sodium chloride crystal, NaCl, or simple table salt. The drawing is shown in Figure P24.10, where the orange spheres are Na+ ions and the blue spheres are Cl− ions. Each ion has a charge of magnitude equal to the elementary charge e. The ions lie on the comers of a cube of side d. You explain to your roommate that the electrical potential energy is defined as zero when all eight charges are infinitely far apart from each other. Then you bring them together to form the crystal structure shown. (a) Evaluate the electric potential energy of the crystal as shown and (b) show that it is energetically favorable for such crystals to form.
Figure P24.10
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
A conductor with an inner cavity, like that shown in (Figure 1), carries a total charge of
+4.60 nC. The charge within the cavity, insulated from the conductor, is q = -6.50 nC.
You may want to review (Page)
Part A
For related problem-solving tips and strategies, you may want to view a Video Tutor
Solution of A conductor with a cavity.
How much charge is on the inner surface of the conductor?
να ΑΣφ
?
qinner =
Submit
Request Answer
Part B
How much charge is on the outer surface of the conductor?
Eνα ΑΣφ
?
Jouter =
nC
Figure
< 1 of 1
Submit
Request Answer
Provide Feedback
Suppose a capacitor consists of two coaxial thin cylindrical conductors. The inner cylinder of radius ra has a charge of +Q, while the outer cylinder of radius rb has charge -Q. The electric field E at a radial distance r from the central axis is given by the function:
E = αe-r/a0 + β/r + b0
where alpha (α), beta (β), a0 and b0 are constants. Find an expression for its capacitance.
First, let us derive the potential difference Vab between the two conductors. The potential difference is related to the electric field by:
Suppose a capacitor consists of two coaxial thin cylindrical conductors. The inner cylinder of radius ra has a charge of +Q, while the outer cylinder of radius rb has charge -Q. The electric field E at a radial distance r from the central axis is given by the function:
E = αe-r/a0 + β/r + b0
where alpha (α), beta (β), a0 and b0 are constants. Find an expression for its capacitance.
First, let us derive the potential difference Vab between the two conductors. The potential difference is related to the electric field by:
Calculating the antiderivative or indefinite integral ,
Vab = (-αa0e-r/a0 + β + b0 )
By definition, the capacitance C is related to the charge and potential difference by:
C = /
Evaluating with the upper and lower limits of integration for Vab, then simplifying:
C = Q / ( (e-rb/a0 - e-ra/a0) + β ln() + b0 () )
Chapter 24 Solutions
Physics for Scientists and Engineers
Ch. 24.1 - In Figure 24.1, two points and are located...Ch. 24.2 - The labeled points in Figure 24.4 are on a series...Ch. 24.3 - In Figure 24.8b, take q2, to be a negative source...Ch. 24.4 - In a certain region of space, the electric...Ch. 24 - How much work is done (by a battery, generator, or...Ch. 24 - (a) Find the electric potential difference Ve...Ch. 24 - Oppositely charged parallel plates are separated...Ch. 24 - Starting with the definition of work, prove that...Ch. 24 - An insulating rod having linear charge density =...Ch. 24 - Review. A block having mass m and charge + Q is...
Ch. 24 - Three positive charges are located at the corners...Ch. 24 - Two point charges Q1 = +5.00 nC and Q2 = 3.00 nC...Ch. 24 - You are working on a laboratory device that...Ch. 24 - Your roommate is having trouble understanding why...Ch. 24 - Four point charges each having charge Q are...Ch. 24 - The two charges in Figure P24.12 are separated by...Ch. 24 - Show that the amount of work required to assemble...Ch. 24 - Two charged particles of equal magnitude are...Ch. 24 - Three particles with equal positive charges q are...Ch. 24 - Review. A light, unstressed spring has length d....Ch. 24 - Review. Two insulating spheres have radii 0.300 cm...Ch. 24 - Review. Two insulating spheres have radii r1 and...Ch. 24 - How much work is required to assemble eight...Ch. 24 - Four identical particles, each having charge q and...Ch. 24 - It is shown in Example 24.7 that the potential at...Ch. 24 - Figure P24.22 represents a graph of the electric...Ch. 24 - Figure P24.23 shows several equipotential lines,...Ch. 24 - An electric field in a region of space is parallel...Ch. 24 - A rod of length L (Fig. P24.25) lies along the x...Ch. 24 - For the arrangement described in Problem 25,...Ch. 24 - A wire having a uniform linear charge density is...Ch. 24 - You are a coach for the Physics Olympics team...Ch. 24 - The electric field magnitude on the surface of an...Ch. 24 - Why is the following situation impossible? A solid...Ch. 24 - A solid metallic sphere of radius a carries total...Ch. 24 - A positively charged panicle is at a distance R/2...Ch. 24 - A very large, thin, flat plate of aluminum of area...Ch. 24 - A solid conducting sphere of radius 2.00 cm has a...Ch. 24 - A spherical conductor has a radius of 14.0 cm and...Ch. 24 - A long, straight wire is surrounded by a hollow...Ch. 24 - Why is the following situation impossible? In the...Ch. 24 - On a dry winter day, you scuff your leather-soled...Ch. 24 - (a) Use the exact result from Example 24.4 to find...Ch. 24 - Why is the following situation impossible? You set...Ch. 24 - The thin, uniformly charged rod shown in Figure...Ch. 24 - A GeigerMueller tube is a radiation detector that...Ch. 24 - Review. Two parallel plates having charges of...Ch. 24 - When an uncharged conducting sphere of radius a is...Ch. 24 - A solid, insulating sphere of radius a has a...Ch. 24 - A hollow, metallic, spherical shell has exterior...Ch. 24 - For the configuration shown in Figure P24.45,...Ch. 24 - An electric dipole is located along the y axis as...Ch. 24 - A disk of radius R (Fig. P24.49) has a nonuniform...Ch. 24 - A particle with charge q is located at x = R, and...Ch. 24 - (a) A uniformly charged cylindrical shell with no...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- Which word or name has the same symmetry as the letters in the name ZAK? (Explain your answer.) a. NUT b. SUE c. CAL d. BIGarrow_forwardA solid sphere of radius R has a spherically symmetrical, nonuniform volume charge density given by (r) = A/r, where r is the radial distance from the center of the sphere in meters, and A is a constant such that the density has dimensions of M/L3. Sketch a graph of the magnitude of the electric field as a function of distance for 0 r 3R.arrow_forwardOften we have distributions of charge for which integrating to find the electric field may not be possible in practice. In such cases, we may be able to get a good approximate solution by dividing the distribution into small but finite particles and taking the vector sum of the contributions of each. To see how this might work, consider a very thin rod of length L = 16 cm with uniform linear charge density = 50.0 nC/m. Estimate the magnitude of the electric field at a point P a distance d = 8.0 cm from the end of the rod by dividing it into n segments of equal length as illustrated in Figure P24.21 for n = 4. Treat each segment as a particle whose distance from point P is measured from its center. Find estimates of EP for n = 1, 2, 4, and 8 segments. FIGURE P24.21arrow_forward
- A potassium chloride molecule (KCl) has a dipole moment of 8.9 1030 Cm. Assume the KCl molecule is in a uniform electric field of 325 N/C. What is the change in the systems potential energy when the molecule rotates a. from = 170 to 180, b. from = 90 to 100, and c. from = 10 to 0?arrow_forwardHow many electrons are in a 1.00-g electrically neutral steel paper clip? The molar mass of steel is approximately that of iron, or 55.845 g/mol, and a neutral iron atom has 26 electrons.arrow_forwardSuppose a capacitor consists of two coaxial thin cylindrical conductors. The inner cylinder of radius ra has a charge of +Q, while the outer cylinder of radius rb has charge -Q. The electric field E at a radial distance r from the central axis is given by the function: E = αe-r/a0 + β/r + b0 where alpha (α), beta (β), a0 and b0 are constants. Find an expression for its capacitance.arrow_forward
- Suppose a capacitor consists of two coaxial thin cylindrical conductors. The inner cylinder of radius ra has a charge of +Q, while the outer cylinder of radius rb has charge -Q. The electric field E at a radial distance r from the central axis is given by the function: E = αe-r/a0 + β/r + b0 where alpha (α), beta (β), a0 and b0 are constants. Find an expression for its capacitance. First, let us derive the potential difference Vab between the two conductors. The potential difference is related to the electric field by: First, let us derive the potential difference Vab between the two conductors. The potential difference is related to the electric field by: Calculating the antiderivative or indefinite integral , Vab = (-αa0e-r/a0 + β + b0 ) By definition, the capacitance C is related to the charge and potential difference by: C = / Evaluating with the upper and lower limits of integration for Vab, then simplifying: C = Q / ( (e-rb/a0 - e-ra/a0) + β ln() + b0 () )arrow_forwardSuppose a capacitor consists of two coaxial thin cylindrical conductors. The inner cylinder of radius ra has a charge of +Q, while the outer cylinder of radius rb has charge -Q. The electric field E at a radial distance r from the central axis is given by the function: E = αe-r/a0 + β/r + b0 where alpha (α), beta (β), a0 and b0 are constants. Find an expression for its capacitance.arrow_forward23.57 - An Ionic Crystal. Figure P23.57 Figure P23.57 shows eight point charges arranged at the comers of a cube with sides of length d. The values of the charges are +q and -q, as shown. This is +9 a model of one cell of a cubic ionic crystal. In sodium chloride (NaCI), for instance, the posi- tive ions are Na* and the nega- tive ions are CI". (a) Calculate the potential energy U of this arrangement. (Take as zero the potential energy of the eight charges when they are infinitely far apart.) (b) In part (a), you should have found that U < 0. Explain the relationship between this result and the observation that such ionic crystals exist in nature.arrow_forward
- The figure shows the interface between two linear dielectrics, silicon and silicon dioxide with relative permittivities 12 and 4 respectively. The interfaces of the two materials are perpendicular to the 2 as shown in the figure, and can contain an interface charge density, p. An electric field in silicon dioxide (region 1) is given as : 4î + 6k kV/m. D₁ and D₂ are the corresponding electric displacements in regions 1 and 2 respectively. The questions on this page are all based on this E₁ = system. Р E2, D2, E₁, D₁ Region 2: Si Er2 = 12 Er1 = 4 Region 1: SiO₂ N 12, Xarrow_forwardSuppose a capacitor consists of two coaxial thin cylindrical conductors. The inner cylinder of radius ra has a charge of +Q, while the outer cylinder of radius rp has charge -Q. The electric field E at a radial distance r from the central axis is given by the function: E = ae-r/ao + B/r + bo where alpha (a), beta (B), ao and bo are constants. Find an expression for its capacitance. First, let us derive the potential difference Vab between the two conductors. The potential difference is related to the electric field by: ['´e Vob = Edr= - Edr Calculating the antiderivative or indefinite integral, Vab = (-aaoe¯r7ao + B + bo By definition, the capacitance C is related to the charge and potential difference by: C = Evaluating with the upper and lower limits of integration for Vab, then simplifying: C = Q / ( (erb/ao - eralao) + B In( ) + bo ( ))arrow_forwardE8P9 Part d, e and f please.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers
Physics
ISBN:9781337553278
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...
Physics
ISBN:9781337553292
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...
Physics
ISBN:9781133939146
Author:Katz, Debora M.
Publisher:Cengage Learning
8.02x - Lect 1 - Electric Charges and Forces - Coulomb's Law - Polarization; Author: Lectures by Walter Lewin. They will make you ♥ Physics.;https://www.youtube.com/watch?v=x1-SibwIPM4;License: Standard YouTube License, CC-BY