Chapter 2.2, Problem 53E

(a)

To determine

To find: the percentile is a pregnancy that lasts 240 days

Answer to Problem 53E

The proportion of pregnancies is lasting less than 240 days.

Explanation of Solution

Given:

Mean is 266 days

Standard deviation is 16 days.

Calculation:

For the given information, $\mu =266days$ , $\sigma =16days$

State: Let x be a random variable defined as length of pregnancies. We want the proportionof pregnancies that last less than 240 days.

Plan: The proportion of pregnancies lasting less than 240 days.

  

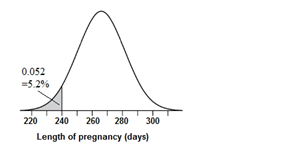
Do: For x =240, we find the corresponding z value as follows:

  $\begin{array}{l}z=\frac{240-266}{16}\\ =-1.63\end{array}$

Therefore, the area below 240 is equal to the area below -1.63 in z-scale.

Using standard normal table, we can see that the proportion of observations below -1.63 is

0.0516 That is about 5.2%

Conclude: About 5.2% of pregnancies last less than 240 days.

Conclusion:

Therefore, About 5.2% of pregnancies last less than 240 days.

(b)

To determine

To find:the percent of pregnancies which last between 240 and 270 days

Answer to Problem 53E

Approximately 55% of pregnancies last between 240 and 270 days.

Explanation of Solution

Calculation:

Let x be a random variable defined as length of pregnancies. We want the proportion of pregnancies lasting between 240 and 270 days.

Plan: The proportion of pregnancies lasting between 240 and 270 days.

  

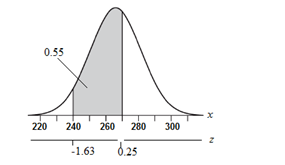
Do: From part (a), we have seen that for x = 240, z = -1.63.

For, x = 270, we can calculate the z value as follows:

  $\begin{array}{l}z=\frac{170-266}{16}\\ =0.25\end{array}$

From the standard normal table, the z value corresponding to 0.25 is 0.5987. Also, from part (a), the z value corresponding to -1.63 is 0.052. Therefore, the proportion of observations between

-1.63 and 0.25 is given by

0.5987 − 0.0516 = 0.5471

Approximately 55% of pregnancies last between 240 and 270 days.

Conclusion:

Therefore, 55% of pregnancies last between 240 and 270 days.

(c)

To determine

To find: the number of days that 20% of pregnancies last

Answer to Problem 53E

The longest 20% of pregnancies last approximately 279 or more days.

Explanation of Solution

Calculation:

Let x be a random variable defined as length of pregnancies. We want the number ofdays such that 80% of people have shorter pregnancies than that number of days.

Plan: The 80^th percentile for the length of human pregnancy is shown in the graph below

  

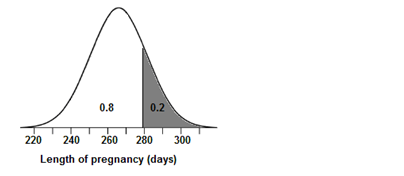
Do: From standard normal table, the value of z corresponding to 0.80 is 0.84. Therefore, the

80^th percentile for the length of human pregnancy can be found by solving the equation:

  $\begin{array}{l}0.84=\frac{x-266}{16}\\ x=279.44\end{array}$

Conclude: The longest 20% of pregnancies last approximately 279 or more days.

Conclusion:

Therefore, the longest 20% of pregnancies last approximately 279 or more days.