Physics, Books a la Carte Edition (5th Edition)

5th Edition

ISBN: 9780134020853

Author: James S. Walker

Publisher: PEARSON

See similar textbooks

Concept explainers

Combination of Capacitors

The connection of capacitors can be established in a circuit in two ways.

Videos

Textbook Question

Chapter 21, Problem 103PP

BIO Footwear Safety

The American National Standards Institute (ANSI) specifies safety standards for a number of potential workplace hazards. For example, ANSI requires that footwear provide protection against the effects of compression from a static weight, impact from a dropped object, puncture from a sharp tool, and cuts from saws. In addition, to protect against the potentially lethal effects of an electrical shock, ANSI provides standards for the electrical resistance that a person and footwear must offer to the flow of electric current.

Specifically, regulation ANSI Z41-1999 states that the resistance of a person and his or her footwear must be tested with the circuit shown in Figure 21-63. In this circuit, the voltage supplied by the battery is ɛ = 50.0 V and the resistance in the circuit is R = 1.00 MΩ. Initially the circuit is open and no current flows. When a person touches the metal sphere attached to the battery, however, the circuit is closed and a small current flows through the person, the shoes, and back to the battery. The amount of current flowing through the person can be determined by using a voltmeter to measure the voltage drop V across the resistor R. To be safe, the current should not exceed 150 µF.

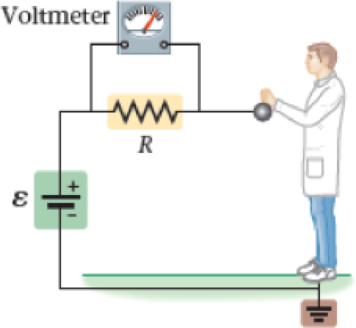
Chapter 21, Problem 103PP, BIO Footwear Safety The American National Standards Institute (ANSI) specifies safety standards for , example 1

Notice that the experimental setup in Figure 21-63 is a dc circuit with two resistors in series—the resistance R and the resistance of the person and footwear, R_pf. It follows that the current in the circuit is I = ɛ/(R + R_pf). We also know that the current is I = V/R, where V is the reading of the voltmeter. These relationships can be combined to relate the voltage V to the resistance R_pf with the result shown in Figure 21-64. According to ANSI regulations, Type II footwear must give a resistance R_pf in the range of 0.1 × 10⁷ Ω to 100 × 10⁷ Ω.

Figure 21-64 Problems 103, 104, 105, and 106

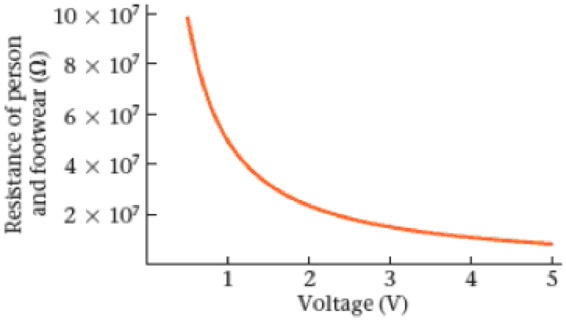
Chapter 21, Problem 103PP, BIO Footwear Safety The American National Standards Institute (ANSI) specifies safety standards for , example 2

103. • Suppose the voltmeter measures a potential difference of 3.70 V across the resistor. What is the current that flows through the person’s body?

A. 3.70 × 10^-6 A
B. 5.00 × 10^-5 A
C. 0.0740 A
D. 3.70 A

Expert Solution & Answer

Want to see the full answer?

Check out a sample textbook solution

See solution

Chapter 21, Problem 102GP

Chapter 21, Problem 104PP

Students have asked these similar questions

No Chatgpt please will upvote h

Helicobacter pylori (H. pylori) is a helically-shaped bacterium that is usually found in the stomach. It burrows through the gastric mucous lining to establish an infection in the stomach's epithelial cells (see photo). Approximately 90% of the people infected with H. pylori will never experience symptoms. Others may develop peptic ulcers and show symptoms of chronic gastritis. The method of motility of H. pylori is a prokaryotic flagellum attached to the back of the bacterium that rigidly rotates like a propeller on a ship. The flagellum is composed of proteins and is approximately 40.0 nm in diameter and can reach rotation speeds as high as 1.50 x 103 rpm. If the speed of the bacterium is 10.0 μm/s, how far has it moved in the time it takes the flagellum to rotate through an angular displacement of 5.00 * 10² rad? Zina Deretsky, National Science Foundation/Flickr H. PYLORI CROSSING MUCUS LAYER OF STOMACH H.pylori Gastric Epithelial mucin cells gel Number i 318 Units um H.pylori…

T1. Calculate what is the received frequency when the car drives away from the radar antenna at a speed v of a) 1 m/s ( = 3.6 km/h), b) 10 m/s ( = 36 km/h), c) 30 m /s ( = 108 km/h) . The radar transmission frequency f is 24.125 GHz = 24.125*10^9 Hz, about 24 GHz. Speed of light 2.998 *10^8 m/s.

Chapter 21 Solutions

Physics, Books a la Carte Edition (5th Edition)

Ch. 21.1 - Enhance Your Understanding 1. The following...Ch. 21.2 - Enhance Your Understanding 2. If the voltage and...Ch. 21.3 - Enhance Your Understanding 3. In the following...Ch. 21.4 - The two circuits shown in Figure 21-17 have...Ch. 21.5 - Prob. 5EYU Ch. 21.6 - Do two capacitors give a larger equivalent...Ch. 21.7 - Give a symbolic expression for the current that...Ch. 21 - Your body is composed of electric charges. Does it...Ch. 21 - Suppose you charge a comb by rubbing it through...Ch. 21 - An electron moving through a wire has an average...

Additional Science Textbook Solutions

Find more solutions based on key concepts

What type of unconformity separates layer G from layer F?

Applications and Investigations in Earth Science (9th Edition)

8. A human maintaining a vegan diet (containing no animal products) would be a: a. producer b. primary consume...

Human Biology: Concepts and Current Issues (8th Edition)

What are four functions of connective tissue?

Anatomy & Physiology (6th Edition)

60. You are 9.0 m from the door of your bus, behind the bus, when it pulls away with an acceleration of 1.0 m/...

Physics for Scientists and Engineers: A Strategic Approach, Vol. 1 (Chs 1-21) (4th Edition)

If isomer A is heated to about 100 C, a mixture of isomers A and B is formed. Explain why there is no trace of ...

Organic Chemistry (8th Edition)

1. ___ Mitosis 2. ___ Meiosis 3. __ Homologous chromosomes 4. __ Crossing over 5. __ Cytokinesis A. Cytoplasmic...

Microbiology with Diseases by Body System (5th Edition)

Knowledge Booster

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.