Chapter 20, Problem 40A

(a)

To determine

The net force on the middle particle.

Answer to Problem 40A

  $F_{net}=18\text{ N}$ , right

Explanation of Solution

Given:

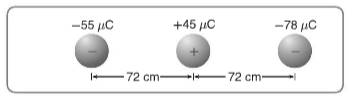
The charge on the left particle is $q_l=-55\mu \text{C}=-\text{55}\times {\text{10}}^{-6}\text{C}$

The charge on the middle particle is $q_m=45\mu \text{C = 45}\times {\text{10}}^{-6}\text{ C}$

The charge on the right particle is $q_r=-78\mu \text{C = }-\text{78}\times {\text{10}}^{-6}\text{ C}$

The middle charge is equally spaced from right and left charges by $d=72\text{ cm = 0}\text{.72 m}$

Formula used:

Coulomb’s law: If $A$ and $B$ are the two objects having charges $q_A$ and $q_B$ respectively which are separated by a distance $d$ , then Coulomb’s law can be written as,

  $F\text{ = K}\frac{q_A{q}_B}{d^2}$

Where $\text{ K}$ is a constant, $\text{ K}=9\times {10}^9\text{ N}{\text{.m}}^2/C^2$

Calculation:

The electrostatic force between the middle and left charge is

  $F_l\text{ = K}\frac{q_l{q}_m}{d^2}$

Electrostatic force between the middle and right charge is,

  $F_r\text{ = K}\frac{q_r{q}_m}{d^2}$

Let left be the negative direction. Hence the net force on the middle charge can be calculated by,

  $\begin{array}{l}{F}_{net}\text{ = }-F_l+F_r\\ F_{net}\text{ = }-\text{K}\frac{q_l{q}_m}{d^2}+\text{K}\frac{q_r{q}_m}{d^2}\end{array}$ $\left(1\right)$

Substituting the numerical values in equation $\left(1\right)$

  $\begin{array}{c}{F}_{net}=\frac{-\left(9\times {10}^9\text{ N}{\text{.m}}^2/C^2\right)\left(55\times {\text{10}}^{-6}\text{ C}\right)\left(45\times {\text{10}}^{-6}\text{ C}\right)}{{\left(\text{0}\text{.72 m}\right)}^2}\\ +\frac{\left(9\times {10}^9\text{ N}{\text{.m}}^2/C^2\right)\left(78\times {\text{10}}^{-6}\text{ C}\right)\left(45\times {\text{10}}^{-6}\text{ C}\right)}{{\left(\text{0}\text{.72 m}\right)}^2}\\ =18\text{N, right}\end{array}$

Conclusion:

Therefore, the net force on the middle particle is $18\text{ N}$ , towards right.

(b)

To determine

The net force on the right particle.

Answer to Problem 40A

  $F_{net}=-42\text{ N}$ , left

Explanation of Solution

Given:

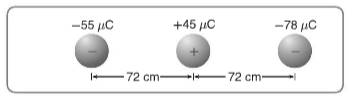
The charge on the left particle is $q_l=-55\mu \text{C}=-\text{55}\times {\text{10}}^{-6}\text{C}$

The charge on the middle particle is $q_m=45\mu \text{C = 45}\times {\text{10}}^{-6}\text{ C}$

The charge on the right particle is $q_r=-78\mu \text{C = }-\text{78}\times {\text{10}}^{-6}\text{ C}$

The middle charge is equally spaced from right and left charges by $d=72\text{ cm = 0}\text{.72 m}$

Formula used:

Coulomb’s law: If $A$ and $B$ are the two objects having charges $q_A$ and $q_B$ respectively which are separated by a distance $d$ , then Coulomb’s law can be written as,

  $F\text{ = K}\frac{q_A{q}_B}{d^2}$

Where $\text{ K}$ is a constant, $\text{ K}=9\times {10}^9\text{ N}{\text{.m}}^2/C^2$

Calculation:

The electrostatic force between the right and left charge is,

  $F_l\text{ = K}\frac{q_l{q}_r}{{\left(2d\right)}^2}$

The electrostatic force between the middle and right charge is,

  $F_m\text{ = K}\frac{q_r{q}_m}{d^2}$

Let left be the negative direction and hence the net force on the right charge can be calculated by,

  $\begin{array}{l}{F}_{net}\text{ = }{F}_l-F_m\\ F_{net}\text{ = K}\frac{q_l{q}_r}{{\left(2d\right)}^2}-\text{K}\frac{q_r{q}_m}{d^2}\end{array}$ $\left(2\right)$

Substituting the numerical values in equation $\left(2\right)$

  $\begin{array}{c}{F}_{net}=\frac{\left(9\times {10}^9\text{ N}{\text{.m}}^2/C^2\right)\left(55\times {\text{10}}^{-6}\text{ C}\right)\left(78\times {\text{10}}^{-6}\text{ C}\right)}{{\left(\text{1}\text{.44 m}\right)}^2}+\frac{\left(9\times {10}^9\text{ N}{\text{.m}}^2/C^2\right)\left(78\times {\text{10}}^{-6}\text{ C}\right)\left(45\times {\text{10}}^{-6}\text{ C}\right)}{{\left(\text{0}\text{.72 m}\right)}^2}\\ =-42\text{N, left}\end{array}$

Conclusion:

The net force on the right particle is $-\text{42 N}$ , towards left.