Concept explainers
The structural components of a flexible pavement.
Explanation of Solution
The structural components of a flexible pavement are as follows:
- The subgrade or prepared roadbed,
- The subbase,
- The base and
- The surface course.
It is necessary to check for the evaluation of every component of the flexible pavement and
Subgrade (Prepared Roadbed)
It is first in the line of materials that are to be laid and serves as the foundation of the flexible pavement structure. It is being spread along the horizontal alignment of the pavement. The materials used in this layer are very well compacted to meet the prescribed specifications of strength. In this layer, borrow materials may also be used according to the pavement being constructed.
Subbase Course
This layer is kept above the subgrade and the materials used in this layer are of better quality as compared to that of the materials used in subgrade. The materials used in this flexible pavement structure must meet the specifications in terms of gradation, strength and plastic characteristics.
And in case, if the materials for the subbase course aren't readily available then the subgrade materials are treated to meet the specifications that of the subbase course material properties.
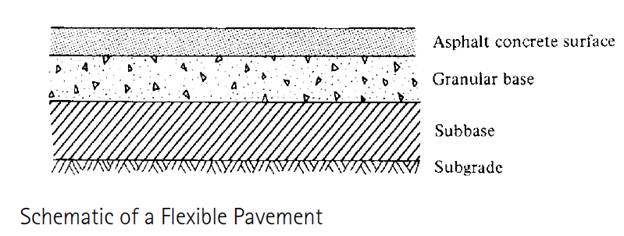
Base Course
This course comes at the third place in the line of pavement structures. It isplaced just above the subbase course and if subbase is not used then it lies immediately above the subgrade of the pavement structure. The materials that are used in this pavement section are of much better quality then those used in the previous layers in terms of gradation, strength and plastic requirements. Granular materials likestone,slag, gravel, and sand are used in this course of flexible pavement structure.Wherein, slag and gravel can be used in both forms i.e., (crushed or uncrushed) stones should be crushed. In case if some of the materials are not meeting the specifications for this line of course then they are used in the base if appropriately stabilized with Portland cement, lime or asphalt.For high quality pavements the material is treated with Portland cement or asphalt to improve the stiffness characteristics of such pavements.
Surface Course
This is the topmost layer of the flexible pavement and is placed above the base course of the road pavement. The materials in this pavement include a mixture of aggregates and asphalt. The properties of this material must be able to hold out against the high tire pressure, withstand abrasive forces due to the traffic flow, prevention against the infiltration of surface water to avoid rupturing of surface course and weakening of the underlying structural bed of the pavement. Considering the expected traffic flow the thickness of this layer varies from 3 inches to 6 inches.
Want to see more full solutions like this?
- What is the budgeted unit work hours, in work hours per unit for the concrete walls?arrow_forwardWhat are the total earned work hours at completion for the column forms?arrow_forward6000 units have been installed to date with 9,000 units to install. Labor costs are $23,300.00 to date. What is the unit cost for labor to date?arrow_forward
- The base rate for labor is $15/hr. The labor burden is 35% and 3% for small tools for the labor. There are 1000 units to install. Records indicate that trade workers can install 10 units per hour, per trade worker. The owners need 15% overhead and profit to pay bills, pay interest on loan and provide some profit to the partners. What is the minimum bid assuming no risk avoidance factor?arrow_forwardCan you show me how to obtain these answers thanks, will rate!arrow_forwardI have the answers for part a just need help with b mostly thanksarrow_forward
- Please explain step by step and show formulasarrow_forward5. (20 Points) Consider a channel width change in the same 7-foot wide rectangular in Problem 4. The horizontal channel narrows as depicted below. The flow rate is 90 cfs, and the energy loss (headloss) through the transition is 0.05 feet. The water depth at the entrance to the transition is initially 4'. 1 b₁ TOTAL ENERGY LINE V² 129 У1 I b₂ TOP VIEW 2 PROFILE VIEW h₁ = 0.05 EGL Y₂ = ? a) b) c) 2 Determine the width, b₂ that will cause a choke at location 2. Determine the water depth at the downstream end of the channel transition (y₂) section if b₂ = 5 feet. Calculate the change in water level after the transition. Plot the specific energy diagram showing all key points. Provide printout in homework. d) What will occur if b₂ = = 1.5 ft.?arrow_forward4. (20 Points) A transition section has been proposed to raise the bed level a height Dz in a 7-foot wide rectangular channel. The design flow rate in the channel is 90 cfs, and the energy loss (headloss) through the transition is 0.05 feet. The water depth at the entrance to the transition section is initially 4 feet. b₁ = b = b2 1 TOTAL ENERGY LINE V² 129 Ут TOP VIEW 2 hloss = 0.05 " EGL Y₂ = ? PROFILE VIEW a) Determine the minimum bed level rise, Dz, which will choke the flow. b) If the step height, Dz = 1 ft, determine the water depth (y2) at the downstream end of the channel transition section. Calculate the amount the water level drops or rises over the step. c) Plot the specific energy diagram showing all key points. Provide printout in Bework. d) What will occur if Dz = 3.0 ft.?. Crest Front Viewarrow_forward
- 1. (20 Points) Determine the critical depth in the trapezoidal drainage ditch shown below. The slope of the ditch is 0.0016, the side slopes are 1V:2.5H, the bottom width is b = 14', and the design discharge is 500 cfs. At this discharge the depth is y = 4.25'. Also, determine the flow regime and calculate the Froude number. Ye= ? Z barrow_forward3. (20 Points) A broad crested weir, 10 feet high, will be constructed in a rectangular channel B feet wide. The weir crest extends a length of B = 120 feet between the banks with 2 - 4 foot wide, round nosed piers in the channel. The width of the weir crest is 8 feet. If H = 6', determine the design discharge for the weir.arrow_forwardParking Needs vs. Alternative Transportation Methods for presentation slides include images and graphsarrow_forward
Traffic and Highway EngineeringCivil EngineeringISBN:9781305156241Author:Garber, Nicholas J.Publisher:Cengage Learning
Construction Materials, Methods and Techniques (M...Civil EngineeringISBN:9781305086272Author:William P. Spence, Eva KultermannPublisher:Cengage Learning
Fundamentals Of Construction EstimatingCivil EngineeringISBN:9781337399395Author:Pratt, David J.Publisher:Cengage,
Principles of Geotechnical Engineering (MindTap C...Civil EngineeringISBN:9781305970939Author:Braja M. Das, Khaled SobhanPublisher:Cengage Learning