VECTOR MECH...,STAT.+DYN.(LL)-W/ACCESS
12th Edition
ISBN: 9781260265453
Author: BEER
Publisher: MCG
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 17.3, Problem 17.110P
A uniform slender bar of length L = 200 mm and mass m = 0.5 kg is supported by a frictionless horizontal table. Initially the bar is spinning about its mass center G with a constant angular speed ω1 = 6 rad/s. Suddenly latch D is moved to the right and is struck by end A of the bar. Knowing that the coefficient of restitution between A and D is e = 0.6, determine the angular velocity of the bar and the velocity of its mass center immediately after the impact.
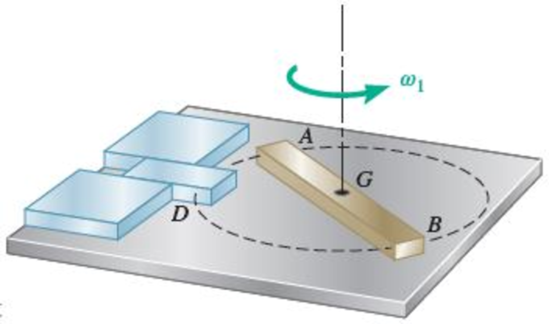
Fig. P17.110
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
The 2.00-kg slender rod shown is hanging in a vertical position and is pin-supported at point A. The slender rod is initially at rest until a 1.000-kg block C, strikes it at its end at point B.The block slides on a frictionless surface with a velocity of 3.50 m/s to the right. After the impact, it slides with a velocity of 1.250 m/s to the right, and the bar rotates with an angular velocity, ω'. Sketch the moment-impulse diagram
1. Which of the following gives the correct kinematic relationship relating the final velocity of the center of the rod, v'G, and its angular velocity, ω'?
2. Which of the following gives the closest value of the coefficient of restitution, e, between the block and the slender rod?
3. Which of the following gives the closest value of the magnitude of the horizontal impulse at the support at point A?
The 2.00-kg slender rod shown is hanging in a vertical position and is pin-supported at point A. The slender rod is initially at rest until a 1.000-kg block C, strikes it at its end at point B.The block slides on a frictionless surface with a velocity of 3.50 m/s to the right. After the impact, it slides with a velocity of 1.250 m/s to the right, and the bar rotates with an angular velocity, ω'.
1. What gives the correct kinematic relationship relating the final velocity of the center of the rod, v' , and its angular velocity, ω'?
2. What is the coefficient of restitution, e, between the block and the slender rod?
3. What is the magnitude of the horizontal impulse at the support at point A?
The 2.00-kg slender rod shown is hanging in a vertical
position and is pin-supported at point A. The slender rod is
initially at rest until a 1.000-kg block C, strikes it at its end
at point B.
The block slides on a frictionless surface with a velocity of
3.50 m/s to the right. After the impact, it slides with a
velocity of 1.250 m/s to the right, and the bar rotates with
an angular velocity, w'.
3.5 m/s
с
B
1.5 m
Answer the following questions given the picture. Please show clear
solutions, I want to learn how to solve the problem.
1a. Which of the following gives the correct kinematic relationship
relating the final velocity of the center of the rod, v'G, and its angular
velocity, w'?
A) 1.333 w'
B) 0.667 w'
C) 1.500 w'
D) 0.75 w'
1b. Which of the following gives the closest value of the magnitude of
the horizontal impulse at the support at point A?
A) 1.125 N-s
B) 1.350 N-s
C) 0.1180 N-s
D) 2.25 N-s
Chapter 17 Solutions
VECTOR MECH...,STAT.+DYN.(LL)-W/ACCESS
Ch. 17.1 - A round object of mass m and radius r is released...Ch. 17.1 - Prob. 17.2CQCh. 17.1 - Prob. 17.3CQCh. 17.1 - Prob. 17.4CQCh. 17.1 - Slender bar A is rigidly connected to a massless...Ch. 17.1 - A 200-kg flywheel is at rest when a constant 300...Ch. 17.1 - The rotor of an electric motor has an angular...Ch. 17.1 - Prob. 17.3PCh. 17.1 - Two disks of the same material are attached to a...Ch. 17.1 - The flywheel of a punching machine has a weight of...
Ch. 17.1 - Prob. 17.6PCh. 17.1 - Prob. 17.7PCh. 17.1 - Prob. 17.8PCh. 17.1 - The 10-in.-radius brake drum is attached to a...Ch. 17.1 - Prob. 17.10PCh. 17.1 - Each of the gears A and B has a mass of 10 kg and...Ch. 17.1 - Solve Prob. 17.11, assuming that the 6 Nm couple...Ch. 17.1 - Prob. 17.13PCh. 17.1 - The double pulley shown has a mass of 15 kg and a...Ch. 17.1 - Gear A has a mass of 1 kg and a radius of gyration...Ch. 17.1 - A slender rod of length l and mass m is pivoted...Ch. 17.1 - The 15-kg rear hatch of a vehicle opens as shown...Ch. 17.1 - A slender 9-lb rod can rotate in a vertical plane...Ch. 17.1 - An adapted golf device attaches to a wheelchair to...Ch. 17.1 - A 10-kg storm window measuring 900 1500 mm is...Ch. 17.1 - A collar with a mass of 1 kg is rigidly attached...Ch. 17.1 - A collar with a mass of 1 kg is rigidly attached...Ch. 17.1 - Two identical slender rods AB and BC are welded...Ch. 17.1 - Prob. 17.24PCh. 17.1 - A 100-kg solid cylindrical disk, 800 mm in...Ch. 17.1 - Prob. 17.26PCh. 17.1 - Greek engineers had the unenviable task of moving...Ch. 17.1 - A small sphere of mass m and radius r is released...Ch. 17.1 - Prob. 17.29PCh. 17.1 - A half-cylinder with mass m and radius r is...Ch. 17.1 - Prob. 17.31PCh. 17.1 - Two uniform cylinders, each of weight W = 14 lb...Ch. 17.1 - Prob. 17.33PCh. 17.1 - A bar of mass m = 5 kg is held as shown between...Ch. 17.1 - The 1.5-kg uniform slender bar AB is connected to...Ch. 17.1 - The motion of the uniform rod AB is guided by...Ch. 17.1 - Prob. 17.37PCh. 17.1 - Prob. 17.38PCh. 17.1 - The ends of a 9-lb rod AB are constrained to move...Ch. 17.1 - The mechanism shown is one of two identical...Ch. 17.1 - The mechanism shown is one of two identical...Ch. 17.1 - Each of the two rods shown is of length L = 1 m...Ch. 17.1 - The 4-kg rod AB is attached to a collar of...Ch. 17.1 - If in Prob. 17.43 the angular velocity of the...Ch. 17.1 - The uniform rods AB and BC are of mass 3 kg and 8...Ch. 17.1 - The uniform rods AB and BC weigh 2.4 kg and 4 kg,...Ch. 17.1 - The 80-mm-radius gear shown has a mass of 5 kg and...Ch. 17.1 - Prob. 17.48PCh. 17.1 - Three shafts and four gears are used to form a...Ch. 17.1 - The experimental setup shown is used to measure...Ch. 17.1 - Prob. 17.51PCh. 17.2 - The 350-kg flywheel of a small hoisting engine has...Ch. 17.2 - Prob. 17.2IMDCh. 17.2 - Prob. 17.3IMDCh. 17.2 - Prob. 17.52PCh. 17.2 - A bolt located 2 in. from the center of an...Ch. 17.2 - A small grinding wheel is attached to the shaft of...Ch. 17.2 - A uniform 144-lb cube is attached to a uniform...Ch. 17.2 - Prob. 17.56PCh. 17.2 - Prob. 17.57PCh. 17.2 - Prob. 17.58PCh. 17.2 - Prob. 17.59PCh. 17.2 - Each of the double pulleys shown has a centroidal...Ch. 17.2 - Each of the gears A and B has a mass of 675 g and...Ch. 17.2 - Two identical uniform cylinders of mass m and...Ch. 17.2 - Two identical 16-lb uniform cylinders of radius r...Ch. 17.2 - Prob. 17.64PCh. 17.2 - Prob. 17.65PCh. 17.2 - Show that, when a rigid body rotates about a fixed...Ch. 17.2 - Prob. 17.68PCh. 17.2 - A flywheel is rigidly attached to a 1.5-in.-radius...Ch. 17.2 - A wheel of radius r and centroidal radius of...Ch. 17.2 - Prob. 17.71PCh. 17.2 - 17.72 and 17.73The 3-lb carriage C is supported as...Ch. 17.2 - Prob. 17.73PCh. 17.2 - Two uniform cylinders, each of mass m = 6 kg and...Ch. 17.2 - Prob. 17.75PCh. 17.2 - Prob. 17.76PCh. 17.2 - A sphere of radius r and mass m is projected along...Ch. 17.2 - A bowler projects an 8.5-in.-diameter ball...Ch. 17.2 - Prob. 17.79PCh. 17.2 - A satellite has a total weight (on Earth) of 250...Ch. 17.2 - Two 10-lb disks and a small motor are mounted on a...Ch. 17.2 - Prob. 17.82PCh. 17.2 - Prob. 17.83PCh. 17.2 - Prob. 17.84PCh. 17.2 - Prob. 17.85PCh. 17.2 - Prob. 17.86PCh. 17.2 - The 30-kg uniform disk A and the bar BC are at...Ch. 17.2 - Prob. 17.88PCh. 17.2 - A 1.8-kg collar A and a 0.7-kg collar B can slide...Ch. 17.2 - Prob. 17.90PCh. 17.2 - A small 4-lb collar C can slide freely on a thin...Ch. 17.2 - Rod AB has a weight of 6 lb and is attached to a...Ch. 17.2 - A 3-kg uniform cylinder A can roll without sliding...Ch. 17.2 - The 4-kg cylinder B and the 3-kg wedge A are at...Ch. 17.2 - The 6-lb steel cylinder A of radius r and the...Ch. 17.3 - A uniform slender rod AB of mass m is at rest on a...Ch. 17.3 - Prob. 17.5IMDCh. 17.3 - Prob. 17.6IMDCh. 17.3 - At what height h above its center G should a...Ch. 17.3 - A bullet weighing 0.08 lb is fired with a...Ch. 17.3 - In Prob. 17.97, determine (a) the required...Ch. 17.3 - A 16-lb wooden panel is suspended from a pin...Ch. 17.3 - Prob. 17.100PCh. 17.3 - A 45-g bullet is fired with a velocity of 400 m/s...Ch. 17.3 - A 45-g bullet is fired with a velocity of 400 m/s...Ch. 17.3 - The tire shown has a radius R = 300 mm and a...Ch. 17.3 - Prob. 17.104PCh. 17.3 - A uniform slender rod AB of mass m is at rest on a...Ch. 17.3 - A uniform slender rod AB is at rest on a...Ch. 17.3 - A bullet of mass m is fired with a horizontal...Ch. 17.3 - Determine the height h at which the bullet of...Ch. 17.3 - A uniform slender bar of length L = 200 mm and...Ch. 17.3 - A uniform slender rod of length L is dropped onto...Ch. 17.3 - A uniform slender rod AB has a mass m, a length L,...Ch. 17.3 - You have been hired to design a baseball catcher...Ch. 17.3 - The trapeze/lanyard air drop (t/LAD) launch is a...Ch. 17.3 - The uniform rectangular block shown is moving...Ch. 17.3 - The 40-kg gymnast drops from her maximum height of...Ch. 17.3 - A uniform slender rod AB of length L = 600 mm is...Ch. 17.3 - Prob. 17.118PCh. 17.3 - A 1-oz bullet is fired with a horizontal velocity...Ch. 17.3 - For the beam of Prob. 17.119, determine the...Ch. 17.3 - Prob. 17.121PCh. 17.3 - Prob. 17.122PCh. 17.3 - A slender rod AB is released from rest in the...Ch. 17.3 - Prob. 17.124PCh. 17.3 - Block A has a mass m and is attached to a cord...Ch. 17.3 - Prob. 17.126PCh. 17.3 - 17.127 and 17.128Member ABC has a mass of 2.4 kg...Ch. 17.3 - 17.127 and 17.128Member ABC has a mass of 2.4 kg...Ch. 17.3 - Prob. 17.129PCh. 17.3 - Prob. 17.130PCh. 17.3 - A small rubber ball of radius r is thrown against...Ch. 17.3 - Sphere A of mass m and radius r rolls without...Ch. 17.3 - In a game of pool, ball A is rolling without...Ch. 17 - A uniform disk, initially at rest and of constant...Ch. 17 - The 8-in.-radius brake drum is attached to a...Ch. 17 - A uniform slender rod is placed at corner B and is...Ch. 17 - The motion of the slender 250-mm rod AB is guided...Ch. 17 - A baseball attachment that helps people with...Ch. 17 - Disks A and B are made of the same material, are...Ch. 17 - Disks A and B are made of the same material, are...
Additional Engineering Textbook Solutions
Find more solutions based on key concepts
Locate the centroid of the area. Prob. 9-17
INTERNATIONAL EDITION---Engineering Mechanics: Statics, 14th edition (SI unit)
Convert the following quantities from English to SI units: a. 98 Btu/(hr-ft-F) b. 0.24 Btu/(lbm-F) C. 0.04 Ibm/...
Heating Ventilating and Air Conditioning: Analysis and Design
23.23 A highly oxidized and uneven round bar is being turned on a lathe. Would you recommend a small or a large...
Manufacturing Engineering & Technology
3.3 It is known that a vertical force of 200 lb is required to remove the nail at C from the board. As the nail...
Vector Mechanics for Engineers: Statics, 11th Edition
17–1C A high-speed aircraft is cruising in still air. How does the temperature of air at the nose of the aircra...
Thermodynamics: An Engineering Approach
What is the importance of modeling in engineering? How are the mathematical models for engineering processes pr...
Heat and Mass Transfer: Fundamentals and Applications
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- A bowler sends his ball down the lane with a forward velocity of 10 ft/s and backspin of 12 rad/s. His ball weighs 16 lbs and has a diameter of 10 in. Knowing that a bowling ball has more weight concentrated towards the center, we will estimate the mass moment of inertia as: I = mr². Starting from t, at the moment the ball hits the alley, and knowing the coefficient of friction is 0.10, determine: (a) The time t, when the ball starts to roll forward without sliding (b) The speed of the ball at this time (c) The distance the ball has traveled at this time 00 Voarrow_forwardDisk A has mass mA = 4.5 kg, radius rA = 278 mm, and initial angular velocity ω0A = 300 rpm clockwise. Disk B has mass mB = 1.0 kg, radius rB = 199 mm, and is at rest when it comes into contact with disk A. Knowing that μk = 0.45 between the disks and neglecting rolling friction ,arrow_forwardTwo uniform cylinders, each of mass m = 6 kg and radius r = 125 mm, are connected by a belt as shown. If the system is released from rest when t = 0, determine (a ) the velocity of the center of cylinder B at t=3s, ( b) the tension in the portion of belt connecting the two cylinders.arrow_forward
- Problem (4) An 8-kg gear with a radius of 80 mm has a centroidal radius of gyration of 50 mm. A 5-kg slender rod AB is attached to the center of the gear while the pin at B is free to slide along the vertical slot (negligible friction). When 0 = 60°, the system is released from rest. Determine S0 mm (a) the velocity of the center of the gear when 6 = 15° (b) the velocity of pin B when 0 = 15°. 320 imm BOarrow_forwardThe motion of the 5.00-kg uniform rod AB is guided by small wheels of negligible mass that roll on the horizontal and vertical surfaces shown. A spring of constant k = 430 N/m is attached to end B of the rod. The rod is released from rest when 0 = 30° (Position 1) and gets pulled down by the initially deformed spring. When 0 = 45° (Position 2), the spring is at its undeformed length. Hint: Irod = 1/12 mL² 1. Which of the following force/s does/do work on rod AB? 1. Normal force on roller A II. Normal force on roller B III. Spring force at B IV. Weight of rod AB B 2. Which of the following best approximates the value of the elastic potential energy of the system at Position 1? 0.6 m 3. Which of the following best approximates the work done by gravity on the rod during its motion from Position 1 to Position 2? 5. Which of the following best approximates the angular speed of the rod at Position 2? A 4. Which of the following expressions gives the kinetic energy of the rod at Position 2 in…arrow_forwardA sphere of radius r and mass m has a linear velocity v0 directed to the left and no angular velocity as it is placed on a belt moving to the right with a constant velocity v1. If after first sliding on the belt the sphere is to have no linear velocity relative to the ground as it starts rolling on the belt without sliding, determine in terms of v1 and the coefficient of kinetic friction µk between the sphere and the belt (a) the required value of v0, (b) the time t1 at which the sphere will start rolling on the belt, (c) the distance the sphere will have moved relative to the ground at time t1.arrow_forward
- The 7.5-lb disk A has a radius r A = 6 in. and is initially at rest. The 10-lb disk B has a radius r B = 8 in. and an angular velocity w0 of 900 rpm when it is brought into contact with disk A. Neglecting friction in the bearings, determine (a) the final angular velocity of each disk, (b) the total impulse of the friction force exerted on disk A.arrow_forwardA 1.6-kg tube AB can slide freely on rod DE which in turn can rotate freely in a horizontal plane. Initially the assembly is rotating with an angular velocity of magnitude w = 5 rad/s and the tube is held in position by a cord. The moment of inertia of the rod and bracket about the vertical axis of rotation is 0.30 kg.m2 and the centroidal moment of inertia of the tube about a vertical axis is 0.0025 kg.m2If the cord suddenly breaks, determine (a) the angular velocity of the assembly after the tube has moved to end E, (b) the energy lost during the plastic impact at E.arrow_forwardTwo disks A and B, of mass m 1 kg each and of radius 10 cm, are placed on a horizontal table. The disk A is launched in translation with a speed of 10 m/s along the y axis, B is at rest. The coefficient of kinetic friction between the disks is 0.5. The line of impact is at an angle of 60° with the x-axis. The moment of inertia of each disk around its center of mass is I = 1₂ = 0.005 kg.m². The coefficient of restitution between the two disks is e=0.6. a) Determine the velocities of the centers of the two disks, just after the impact. b) Calculate the angular velocities of the disks, just after the impact. c) Calculate the energy loss during the impact.arrow_forward
- Don't just send the answer from Beer's Manual. I want to learn the step by step thank you.arrow_forwardConsider a slender rod AB with a length l and a mass m. The ends are connected to blocks of negligible mass sliding along horizontal and vertical tracks. If the rod is released with no initial velocity from a horizontal position as shown in Fig.A, determine its angular velocity after it has rotated through an angle of θ (see Fig B) using the conservation of energy method. (Hint: Moment of inertia of rod about G = (1/12)ml2 The kinetic energy of a rigid body in plane motion isarrow_forwardA gum with mass m is attached to the rim of a disk with mass M and radius R The disk and the gum Is Initlally rotating with angular speed w around an axis which passes through the center of the disk and is perpendicular to the plane of the disk as shown in the figure. At a certain instant the gum breaks loose and flies off with a speed of v. Which of the followings gives the correct expression for the final angular speed w of the disk. (Igum = mR, Iatak = MR /2) %3D %3D Select one: Mv 2m 1+ M O a. 2mR M. ). 2mv 2m 1+ M O b. - MR 2Mv 2M 1+ :), • d. (1+) - mR MR mv O e. 1+ 2M - 2MRarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Elements Of Electromagnetics
Mechanical Engineering
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Oxford University Press
Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:9780134319650
Author:Russell C. Hibbeler
Publisher:PEARSON
Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:9781259822674
Author:Yunus A. Cengel Dr., Michael A. Boles
Publisher:McGraw-Hill Education
Control Systems Engineering
Mechanical Engineering
ISBN:9781118170519
Author:Norman S. Nise
Publisher:WILEY
Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning
Engineering Mechanics: Statics
Mechanical Engineering
ISBN:9781118807330
Author:James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:WILEY
Dynamics - Lesson 1: Introduction and Constant Acceleration Equations; Author: Jeff Hanson;https://www.youtube.com/watch?v=7aMiZ3b0Ieg;License: Standard YouTube License, CC-BY