Loose Leaf for Vector Mechanics for Engineers: Statics and Dynamics
12th Edition
ISBN: 9781259977206
Author: BEER, Ferdinand P., Johnston Jr., E. Russell, Mazurek, David, Cornwell, Phillip J., SELF, Brian
Publisher: McGraw-Hill Education
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 17.2, Problem 17.80P
A satellite has a total weight (on Earth) of 250 lbs, and each of the solar panels weighs 15 lbs. The body of the satellite has a mass moment of inertia about the z axis of 6 slug·ft2, and the panels can be modeled as flat plates. The satellite spins with a rate of 10 rpm about the z axis when the solar panels are positioned in the xy plane. Determine the spin rate about z after a motor on the satellite has rotated both panels to be positioned in the yz plane (as shown in the figure).
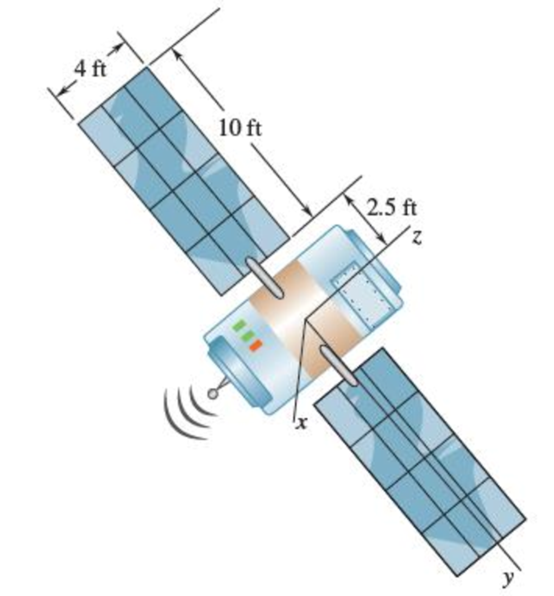
Fig. P17.80
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
(Three-Dimensional Motion)
A homogeneous 20-cm-diameter disk has a mass of 1 kg and is placed on apedestal so that the assembly precesses in a horizontal plane as shown. If theprecessional rate is 20i rpm, determine the magnitude and direction of the spinof the disk about its own axle.
I need the answer as soon as possible
A spinning top consists of a ring (which can be treated as a cylindrical shell) of mass
m = 0.52 kg and a radius of 60 mm is mounted on its central pointed shaft with
spokes. The spokes and pointed shaft have negligible mass meaning that their inertia
can be neglected. The top has a spin velocity of 10,000 rev/min and is released on a
horizontal surface with the point at O remaining at a fixed point. Assuming that the
spin angular velocity is high when compared to the precession, determine the
magnitude of the precession angular velocity of the top.
15°
60
mm
80 mm
10000 rev/min
O 1.2 rad/sec
0.6 rad/sec
0.2 rad/sec
2.1 rad/sec
Chapter 17 Solutions
Loose Leaf for Vector Mechanics for Engineers: Statics and Dynamics
Ch. 17.1 - A round object of mass m and radius r is released...Ch. 17.1 - Prob. 17.2CQCh. 17.1 - Prob. 17.3CQCh. 17.1 - Prob. 17.4CQCh. 17.1 - Slender bar A is rigidly connected to a massless...Ch. 17.1 - A 200-kg flywheel is at rest when a constant 300...Ch. 17.1 - The rotor of an electric motor has an angular...Ch. 17.1 - Prob. 17.3PCh. 17.1 - Two disks of the same material are attached to a...Ch. 17.1 - The flywheel of a punching machine has a weight of...
Ch. 17.1 - Prob. 17.6PCh. 17.1 - Prob. 17.7PCh. 17.1 - Prob. 17.8PCh. 17.1 - The 10-in.-radius brake drum is attached to a...Ch. 17.1 - Prob. 17.10PCh. 17.1 - Each of the gears A and B has a mass of 10 kg and...Ch. 17.1 - Solve Prob. 17.11, assuming that the 6 Nm couple...Ch. 17.1 - Prob. 17.13PCh. 17.1 - The double pulley shown has a mass of 15 kg and a...Ch. 17.1 - Gear A has a mass of 1 kg and a radius of gyration...Ch. 17.1 - A slender rod of length l and mass m is pivoted...Ch. 17.1 - The 15-kg rear hatch of a vehicle opens as shown...Ch. 17.1 - A slender 9-lb rod can rotate in a vertical plane...Ch. 17.1 - An adapted golf device attaches to a wheelchair to...Ch. 17.1 - A 10-kg storm window measuring 900 1500 mm is...Ch. 17.1 - A collar with a mass of 1 kg is rigidly attached...Ch. 17.1 - A collar with a mass of 1 kg is rigidly attached...Ch. 17.1 - Two identical slender rods AB and BC are welded...Ch. 17.1 - Prob. 17.24PCh. 17.1 - A 100-kg solid cylindrical disk, 800 mm in...Ch. 17.1 - Prob. 17.26PCh. 17.1 - Greek engineers had the unenviable task of moving...Ch. 17.1 - A small sphere of mass m and radius r is released...Ch. 17.1 - Prob. 17.29PCh. 17.1 - A half-cylinder with mass m and radius r is...Ch. 17.1 - Prob. 17.31PCh. 17.1 - Two uniform cylinders, each of weight W = 14 lb...Ch. 17.1 - Prob. 17.33PCh. 17.1 - A bar of mass m = 5 kg is held as shown between...Ch. 17.1 - The 1.5-kg uniform slender bar AB is connected to...Ch. 17.1 - The motion of the uniform rod AB is guided by...Ch. 17.1 - Prob. 17.37PCh. 17.1 - Prob. 17.38PCh. 17.1 - The ends of a 9-lb rod AB are constrained to move...Ch. 17.1 - The mechanism shown is one of two identical...Ch. 17.1 - The mechanism shown is one of two identical...Ch. 17.1 - Each of the two rods shown is of length L = 1 m...Ch. 17.1 - The 4-kg rod AB is attached to a collar of...Ch. 17.1 - If in Prob. 17.43 the angular velocity of the...Ch. 17.1 - The uniform rods AB and BC are of mass 3 kg and 8...Ch. 17.1 - The uniform rods AB and BC weigh 2.4 kg and 4 kg,...Ch. 17.1 - The 80-mm-radius gear shown has a mass of 5 kg and...Ch. 17.1 - Prob. 17.48PCh. 17.1 - Three shafts and four gears are used to form a...Ch. 17.1 - The experimental setup shown is used to measure...Ch. 17.1 - Prob. 17.51PCh. 17.2 - The 350-kg flywheel of a small hoisting engine has...Ch. 17.2 - Prob. 17.2IMDCh. 17.2 - Prob. 17.3IMDCh. 17.2 - Prob. 17.52PCh. 17.2 - A bolt located 2 in. from the center of an...Ch. 17.2 - A small grinding wheel is attached to the shaft of...Ch. 17.2 - A uniform 144-lb cube is attached to a uniform...Ch. 17.2 - Prob. 17.56PCh. 17.2 - Prob. 17.57PCh. 17.2 - Prob. 17.58PCh. 17.2 - Prob. 17.59PCh. 17.2 - Each of the double pulleys shown has a centroidal...Ch. 17.2 - Each of the gears A and B has a mass of 675 g and...Ch. 17.2 - Two identical uniform cylinders of mass m and...Ch. 17.2 - Two identical 16-lb uniform cylinders of radius r...Ch. 17.2 - Prob. 17.64PCh. 17.2 - Prob. 17.65PCh. 17.2 - Show that, when a rigid body rotates about a fixed...Ch. 17.2 - Prob. 17.68PCh. 17.2 - A flywheel is rigidly attached to a 1.5-in.-radius...Ch. 17.2 - A wheel of radius r and centroidal radius of...Ch. 17.2 - Prob. 17.71PCh. 17.2 - 17.72 and 17.73The 3-lb carriage C is supported as...Ch. 17.2 - Prob. 17.73PCh. 17.2 - Two uniform cylinders, each of mass m = 6 kg and...Ch. 17.2 - Prob. 17.75PCh. 17.2 - Prob. 17.76PCh. 17.2 - A sphere of radius r and mass m is projected along...Ch. 17.2 - A bowler projects an 8.5-in.-diameter ball...Ch. 17.2 - Prob. 17.79PCh. 17.2 - A satellite has a total weight (on Earth) of 250...Ch. 17.2 - Two 10-lb disks and a small motor are mounted on a...Ch. 17.2 - Prob. 17.82PCh. 17.2 - Prob. 17.83PCh. 17.2 - Prob. 17.84PCh. 17.2 - Prob. 17.85PCh. 17.2 - Prob. 17.86PCh. 17.2 - The 30-kg uniform disk A and the bar BC are at...Ch. 17.2 - Prob. 17.88PCh. 17.2 - A 1.8-kg collar A and a 0.7-kg collar B can slide...Ch. 17.2 - Prob. 17.90PCh. 17.2 - A small 4-lb collar C can slide freely on a thin...Ch. 17.2 - Rod AB has a weight of 6 lb and is attached to a...Ch. 17.2 - A 3-kg uniform cylinder A can roll without sliding...Ch. 17.2 - The 4-kg cylinder B and the 3-kg wedge A are at...Ch. 17.2 - The 6-lb steel cylinder A of radius r and the...Ch. 17.3 - A uniform slender rod AB of mass m is at rest on a...Ch. 17.3 - Prob. 17.5IMDCh. 17.3 - Prob. 17.6IMDCh. 17.3 - At what height h above its center G should a...Ch. 17.3 - A bullet weighing 0.08 lb is fired with a...Ch. 17.3 - In Prob. 17.97, determine (a) the required...Ch. 17.3 - A 16-lb wooden panel is suspended from a pin...Ch. 17.3 - Prob. 17.100PCh. 17.3 - A 45-g bullet is fired with a velocity of 400 m/s...Ch. 17.3 - A 45-g bullet is fired with a velocity of 400 m/s...Ch. 17.3 - The tire shown has a radius R = 300 mm and a...Ch. 17.3 - Prob. 17.104PCh. 17.3 - A uniform slender rod AB of mass m is at rest on a...Ch. 17.3 - A uniform slender rod AB is at rest on a...Ch. 17.3 - A bullet of mass m is fired with a horizontal...Ch. 17.3 - Determine the height h at which the bullet of...Ch. 17.3 - A uniform slender bar of length L = 200 mm and...Ch. 17.3 - A uniform slender rod of length L is dropped onto...Ch. 17.3 - A uniform slender rod AB has a mass m, a length L,...Ch. 17.3 - You have been hired to design a baseball catcher...Ch. 17.3 - The trapeze/lanyard air drop (t/LAD) launch is a...Ch. 17.3 - The uniform rectangular block shown is moving...Ch. 17.3 - The 40-kg gymnast drops from her maximum height of...Ch. 17.3 - A uniform slender rod AB of length L = 600 mm is...Ch. 17.3 - Prob. 17.118PCh. 17.3 - A 1-oz bullet is fired with a horizontal velocity...Ch. 17.3 - For the beam of Prob. 17.119, determine the...Ch. 17.3 - Prob. 17.121PCh. 17.3 - Prob. 17.122PCh. 17.3 - A slender rod AB is released from rest in the...Ch. 17.3 - Prob. 17.124PCh. 17.3 - Block A has a mass m and is attached to a cord...Ch. 17.3 - Prob. 17.126PCh. 17.3 - 17.127 and 17.128Member ABC has a mass of 2.4 kg...Ch. 17.3 - 17.127 and 17.128Member ABC has a mass of 2.4 kg...Ch. 17.3 - Prob. 17.129PCh. 17.3 - Prob. 17.130PCh. 17.3 - A small rubber ball of radius r is thrown against...Ch. 17.3 - Sphere A of mass m and radius r rolls without...Ch. 17.3 - In a game of pool, ball A is rolling without...Ch. 17 - A uniform disk, initially at rest and of constant...Ch. 17 - The 8-in.-radius brake drum is attached to a...Ch. 17 - A uniform slender rod is placed at corner B and is...Ch. 17 - The motion of the slender 250-mm rod AB is guided...Ch. 17 - A baseball attachment that helps people with...Ch. 17 - Disks A and B are made of the same material, are...Ch. 17 - Disks A and B are made of the same material, are...
Additional Engineering Textbook Solutions
Find more solutions based on key concepts
Locate the centroid of the area. Prob. 9-17
Engineering Mechanics: Statics
What parts are included in the vehicle chassis?
Automotive Technology: Principles, Diagnosis, And Service (6th Edition) (halderman Automotive Series)
Define or describe each type of fluid: (a) viscoelastic fluid (b) pseudoplastic fluid (c) dilatant fluid (d) Bi...
Fluid Mechanics: Fundamentals and Applications
What types of polymers are most commonly blow molded?
DeGarmo's Materials and Processes in Manufacturing
A 20-lb force is applied to the control rod AB as shown. Knowing that the length of the rod is 9 in. and that t...
Statics and Mechanics of Materials
Describe the structural changes that take place when a plain-carbon eutectoid steel is slowly cooled from the a...
Foundations of Materials Science and Engineering
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- A spinning top consists of a ring (which can be treated as a cylindrical shell) of mass m = 0.52 kg and a radius of 60 mm is mounted on its central pointed shaft with spokes. The spokes and pointed shaft have negligible mass meaning that their inertia can be neglected. The top has a spin velocity of 10,000 rev/min and is released on a horizontal surface with the point at O remaining at a fixed point. Assuming that the spin angular velocity is high when compared to the precession, determine the magnitude of the precession angular velocity of the top. 15° 60 mm 80 mm 10000 rev/minarrow_forwardHW1 A uniform disc of 220 mm in diameter has a mass of 3.5 kg. It is mounted centrally in bearings that maintain its axle horizontally. The disc spins about its axle with a constant speed of 750 r.p.m. while the axle precesses uniformly about the vertical at 25 r.p.m. The directions of rotation are shown in the figure below. If the distance between the bearings is 200 mm, find the resultant reaction at each bearing due to the mass and gyroscopic effects. 40 +X +Zarrow_forwardA drum can rotate about a fixed-point O. The A block is attached to a cord wrapping around the drum. The mass of the drum is md = 100kg and the radius is r = 0.5 m. The radius of gyration of the drum about point O is ko=0.3 m. The mass of the block is mb= 20kg. The block is released from rest. The acceleration due to gravity is g=9.81 m/s2. (3) Calculate the angular acceleration of the drum α= ________ (rad/s2) (two decimal places)arrow_forward
- A drum can rotate about a fixed-point O. The A block is attached to a cord wrapping around the drum. The mass of the drum is md = 100kg and the radius is r = 0.5 m. The radius of gyration of the drum about point O is ko=0.3 m. The mass of the block is mb= 20kg. The block is released from rest. The acceleration due to gravity is g=9.81 m/s2. (1) Calculate the mass moment of inertia of the drum about the point O, IO_______(kgm2) (two decimal places)arrow_forwardA drum can rotate about a fixed-point O. The A block is attached to a cord wrapping around the drum. The mass of the drum is md = 100kg and the radius is r = 0.5 m. The radius of gyration of the drum about point O is ko=0.3 m. The mass of the block is mb= 20kg. The block is released from rest. The acceleration due to gravity is g=9.81 m/s2. (1) Calculate the mass moment of inertia of the drum about the point O, IO_______(kgm2) (two decimal places)arrow_forwardA drum can rotate about a fixed-point O. The A block is attached to a cord wrapping around the drum. The mass of the drum is md = 100kg and the radius is r = 0.5 m. The radius of gyration of the drum about point O is ko=0.3 m. The mass of the block is mb= 20kg. The block is released from rest. The acceleration due to gravity is g=9.81 m/s2. (3) Calculate the angular acceleration of the drum α= ________ (rad/s2) (two decimal places)arrow_forward
- A drum can rotate about a fixed-point O. The A block is attached to a cord wrapping around the drum. The mass of the drum is md = 100kg and the radius is r = 0.5 m. The radius of gyration of the drum about point O is ko=0.3 m. The mass of the block is mb= 20kg. The block is released from rest. The acceleration due to gravity g=9.81 m/s2 . (2) ) If the mass moment of inertia of the drum about point O is IO, and the angular acceleration of the drum is α, select the correct moment equation of the whole drum-block system about the point O._____________ A. mbgr=rmb(rα) B. 0 C. mbgr=Ioα +rmb(rα) D. mbgr=Ioαarrow_forwardThe top has a mass of 1 kg and radius of gyration of k, = 25 mm about its axis of symmetry. The top spins and precesses about the ball-and-socket joint at point O. It's transverse radius of gyration about point O is k = 63 mm. The spin velocity is ws = 150 rad/s. • Under these conditions. determine the two possible precession velocities op of the top. Determine the kinetic energy of the top for each of the two possible precession velocities.arrow_forwardAn aeroplane makes a complete half circle of 70 metres radius, towards left, when flying at 500 km per hr. The rotary engine and the propeller of the plane has a mass of 600 kg and a radius of gyration of 0.3 m. The engine rotates at 2500 r.p.m. clockwise when viewed from the rear. Find the gyroscopic couple on the aircraft.arrow_forward
- Each paddle wheel of a steamer have a mass of 1600 kg and a radius of gyration of 1.2 meters. The steamer turns to port in a circle of 160 meters radius at 24 km/hr. The speed of the paddle is 90 rpm. Determine the magnitude and effect of the gyroscopic couple acting on the steamer.arrow_forwardQ3 A uniform sphere of 150 mm diameter has a mass of 5 kg. It is mounted centrally in bearings which maintain its axle in a horizontal plane. The sphere spins about its axle with a constant speed of 1000 r.p.m. while the axle precesses uniformly about the vertical at 60 r.p.m. The directions of rotation are as shown in Figure below. If the distance between the bearings is 100 mm, find the resultant reaction at each bearing due to the mass and gyroscopic effects. (35 degrees)arrow_forwardA shaft carries four masses A, B, C and D of magnitudes 18 kg, 15 kg, 27 kg, and 22.5 kg respectively and revolving at radii 20 mm, 25 mm, 30 mm and 15 mm respectively. The masses are rotating in the same plane. The angular position of masses B, C and D are 60 degrees , 135 degrees and 270 degrees from mass A. Find the magnitude and position of the balancing mass at a radius of 50 mm,arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Elements Of Electromagnetics
Mechanical Engineering
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Oxford University Press
Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:9780134319650
Author:Russell C. Hibbeler
Publisher:PEARSON
Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:9781259822674
Author:Yunus A. Cengel Dr., Michael A. Boles
Publisher:McGraw-Hill Education
Control Systems Engineering
Mechanical Engineering
ISBN:9781118170519
Author:Norman S. Nise
Publisher:WILEY
Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning
Engineering Mechanics: Statics
Mechanical Engineering
ISBN:9781118807330
Author:James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:WILEY
Understanding Thermal Radiation; Author: The Efficient Engineer;https://www.youtube.com/watch?v=FDmYCI_xYlA;License: Standard youtube license