Chapter 17, Problem 20P

To determine

Find the Fourier series for the signal shown in Figure 17.58 and verify it using MATLAB and find the value of $f\left(t\right)$ at $t=2$ using the first three nonzero harmonics.

Answer to Problem 20P

The Fourier series $f\left(t\right)$ for the signal in Figure 17.58 is $5+\frac{60}{{\pi }^2}{\displaystyle \sum _{n=1}^{\infty }\left(\frac{1}{n^2}\left(\cos \left(\frac{2n\pi }{3}\right)-\cos \left(\frac{n\pi }{3}\right)\right)\right)\cos \left(\frac{n\pi }{3}\right)t}$ and the value of $f\left(2\right)$ is $9.512$.

Explanation of Solution

Given data:

Refer to Figure 17.58 in the textbook.

Formula used:

Write the expression to calculate the fundamental angular frequency.

${\omega }_0=\frac{2\pi }{T}$        (1)

Here,

$T$ is the period of the function.

Write the general expression to calculate trigonometric Fourier series of $f\left(t\right)$.

$f\left(t\right)=a_0+{\displaystyle \sum _{n=1}^{\infty }\left(a_n\cos n{\omega }_{0}t+b_n\sin n{\omega }_{0}t\right)}$        (2)

Here,

$a_0$ is the dc component of $f\left(t\right)$,

$a_n$ and $b_n$ are the Fourier coefficients,

$n$ is an integer, and

${\omega }_0$ is the angular frequency.

Calculation:

The given waveform is drawn as Figure 1.

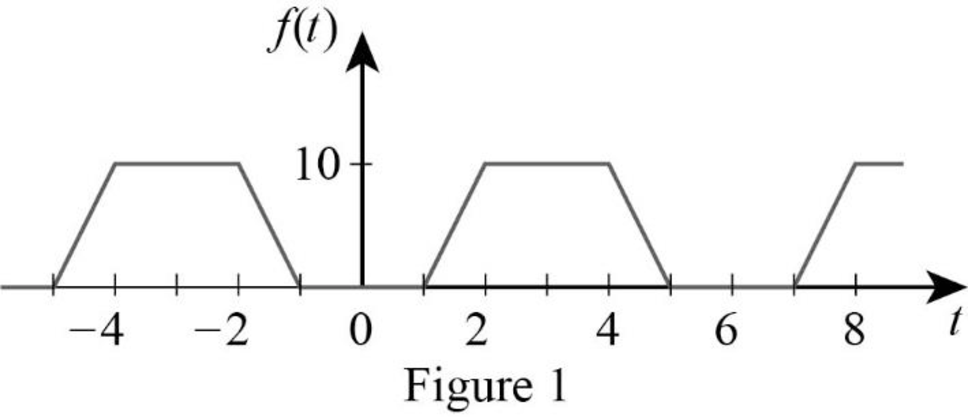
Refer to Figure 1, the waveform is symmetrical about the vertical axis. The signal $f\left(t\right)$ is fold about $t=0$ that is the waveform is unchanged under reflection in the y-axis. Therefore, the waveform is called as an even function and it is expressed as,

$f\left(t\right)=f\left(-t\right)$

Write the expressions for Fourier coefficients for an even function.

$a_0=\frac{2}{T}{\displaystyle \underset{0}{\overset{\frac{T}{2}}{\int }}f\left(t\right)dt}$        (3)

$a_n=\frac{4}{T}{\displaystyle \underset{0}{\overset{\frac{T}{2}}{\int }}f\left(t\right)\cos n{\omega }_{0}tdt}$        (4)

$b_n=0$

Refer to the Figure 1. The Fourier series even function of the waveform is defined as,

$f\left(t\right)=\{\begin{array}{l}0,0<t<1\\ 10t-10,1<t<2\\ 10,2<t<4\\ -10t+50,4<t<5\\ 0,5<t<6\end{array}$

The time period of the function in Figure 1 is,

$T=6$

Substitute $6$ for $T$ in equation (1) to find ${\omega }_0$.

$\begin{array}{c}{\omega }_0=\frac{2\pi }{6}\\ =\frac{\pi }{3}\end{array}$

Substitute $6$ for $T$ in equation (3) to find $a_0$.

$\begin{array}{c}{a}_0=\frac{2}{6}{\displaystyle \underset{0}{\overset{\frac{6}{2}}{\int }}f\left(t\right)dt}\\ =\frac{1}{3}{\displaystyle \underset{0}{\overset{3}{\int }}f\left(t\right)dt}\\ =\frac{1}{3}\left({\displaystyle \underset{0}{\overset{1}{\int }}f\left(t\right)dt}+{\displaystyle \underset{1}{\overset{2}{\int }}f\left(t\right)dt}+{\displaystyle \underset{2}{\overset{3}{\int }}f\left(t\right)dt}\right)\\ =\frac{1}{3}\left({\displaystyle \underset{0}{\overset{1}{\int }}0dt}+{\displaystyle \underset{1}{\overset{2}{\int }}\left(10t-10\right)dt}+{\displaystyle \underset{2}{\overset{3}{\int }}10dt}\right)\end{array}$

Simplify the above equation to find $a_0$.

$\begin{array}{c}{a}_0=\frac{1}{3}\left(0+{\left[10\frac{t^2}{2}-10t\right]}_1^2+{\left[10t\right]}_2^3\right)\\ =\frac{1}{3}\left(10\left[\left(\frac{2^2}{2}-2\right)-\left(\frac{1^2}{2}-1\right)\right]+10\left[3-2\right]\right)\\ =\frac{1}{3}\left(10\left[\left(0\right)+\frac{1}{2}\right]+10\left(1\right)\right)\\ =5\end{array}$

Substitute $6$ for $T$ and $\frac{\pi }{3}$ for ${\omega }_0$ in equation (4) to find $a_n$.

$\begin{array}{c}{a}_n=\frac{4}{6}{\displaystyle \underset{0}{\overset{\frac{6}{2}}{\int }}f\left(t\right)\cos n\left(\frac{\pi }{3}\right)tdt}\\ =\frac{2}{3}\left({\displaystyle \underset{0}{\overset{1}{\int }}f\left(t\right)\cos \left(\frac{n\pi }{3}\right)tdt}+{\displaystyle \underset{1}{\overset{2}{\int }}f\left(t\right)\cos \left(\frac{n\pi }{3}\right)tdt}+{\displaystyle \underset{2}{\overset{3}{\int }}f\left(t\right)\cos \left(\frac{n\pi }{3}\right)tdt}\right)\\ =\frac{2}{3}\left({\displaystyle \underset{0}{\overset{1}{\int }}\left(0\right)\cos \left(\frac{n\pi }{3}\right)tdt}+{\displaystyle \underset{1}{\overset{2}{\int }}\left(10t-10\right)\cos \left(\frac{n\pi }{3}\right)tdt}+{\displaystyle \underset{2}{\overset{3}{\int }}10\cos \left(\frac{n\pi }{3}\right)tdt}\right)\\ =\frac{2}{3}\left(0+{\displaystyle \underset{1}{\overset{2}{\int }}10t\cos \left(\frac{n\pi }{3}\right)tdt}-{\displaystyle \underset{1}{\overset{2}{\int }}10\cos \left(\frac{n\pi }{3}\right)tdt}+{\displaystyle \underset{2}{\overset{3}{\int }}10\cos \left(\frac{n\pi }{3}\right)tdt}\right)\end{array}$

Simplify the above equation to find $a_n$.

$\begin{array}{c}{a}_n=\frac{2}{3}\left(10{\displaystyle \underset{1}{\overset{2}{\int }}t\cos \left(\frac{n\pi }{3}\right)tdt}-10{\left[\frac{\sin \left(\frac{n\pi }{3}\right)t}{\left(\frac{n\pi }{3}\right)}\right]}_1^2+10{\left[\frac{\sin \left(\frac{n\pi }{3}\right)t}{\left(\frac{n\pi }{3}\right)}\right]}_2^3\right)\\ =\frac{2}{3}\left(\begin{array}{l}10{\displaystyle \underset{1}{\overset{2}{\int }}t\cos \left(\frac{n\pi }{3}\right)tdt}-10\left(\frac{3}{n\pi }\right)\left[\sin \left(\frac{n\pi }{3}\right)\left(2\right)-\sin \left(\frac{n\pi }{3}\right)\left(1\right)\right]\\ +10\left(\frac{3}{n\pi }\right)\left[\sin \left(\frac{n\pi }{3}\right)\left(3\right)-\sin \left(\frac{n\pi }{3}\right)\left(2\right)\right]\end{array}\right)\\ =\frac{2}{3}\left(\begin{array}{l}10{\displaystyle \underset{1}{\overset{2}{\int }}t\cos \left(\frac{n\pi }{3}\right)tdt}-\frac{30}{n\pi }\left[\sin \left(\frac{2n\pi }{3}\right)-\sin \left(\frac{n\pi }{3}\right)\right]\\ +\frac{30}{n\pi }\left[\sin n\pi -\sin \left(\frac{2n\pi }{3}\right)\right]\end{array}\right)\\ =\frac{2}{3}\left(\begin{array}{l}10{\displaystyle \underset{1}{\overset{2}{\int }}t\cos \left(\frac{n\pi }{3}\right)tdt}-\frac{30}{n\pi }\left[\sin \left(\frac{2n\pi }{3}\right)-\sin \left(\frac{n\pi }{3}\right)\right]\\ +\frac{30}{n\pi }\left[0-\sin \left(\frac{2n\pi }{3}\right)\right]\end{array}\right)\left\{\because \sin n\pi =0\right\}\end{array}$

Simplify the above equation to find $a_n$.

$a_n=\frac{2}{3}\left(10{\displaystyle \underset{1}{\overset{2}{\int }}t\cos \left(\frac{n\pi }{3}\right)tdt}-\frac{30}{n\pi }\sin \left(\frac{2n\pi }{3}\right)+\frac{30}{n\pi }\sin \left(\frac{n\pi }{3}\right)-\frac{30}{n\pi }\sin \left(\frac{2n\pi }{3}\right)\right)$

$a_n=\frac{2}{3}\left(10{\displaystyle \underset{1}{\overset{2}{\int }}t\cos \left(\frac{n\pi }{3}\right)tdt}-\frac{60}{n\pi }\sin \left(\frac{2n\pi }{3}\right)+\frac{30}{n\pi }\sin \left(\frac{n\pi }{3}\right)\right)$        (5)

Assume the following to reduce the equation (5).

$x={\displaystyle \underset{1}{\overset{2}{\int }}t\cos \left(\frac{n\pi }{3}\right)tdt}$        (6)

Substitute the equations (6) in equation (5) to find $a_n$.

$a_n=\frac{2}{3}\left(10x-\frac{60}{n\pi }\sin \left(\frac{2n\pi }{3}\right)+\frac{30}{n\pi }\sin \left(\frac{n\pi }{3}\right)\right)$        (7)

Consider the following integration formula.

${\displaystyle \underset{a}{\overset{b}{\int }}udv}={\left[uv\right]}_a^b-{\displaystyle \underset{a}{\overset{b}{\int }}vdu}$        (8)

Compare the equations (6) and (8) to simplify the equation (6).

$\begin{array}{l}u=tdv=\cos \left(\frac{n\pi }{3}\right)tdt\\ du=dtv=\frac{\sin \left(\frac{n\pi }{3}\right)t}{\left(\frac{n\pi }{3}\right)}\end{array}$

Using the equation (8), the equation (6) can be reduced as,

$\begin{array}{c}x={\displaystyle \underset{1}{\overset{2}{\int }}t\cos \left(\frac{n\pi }{3}\right)tdt}\\ ={\left[\left(t\right)\frac{\sin \left(\frac{n\pi }{3}\right)t}{\left(\frac{n\pi }{3}\right)}\right]}_1^2-{\displaystyle \underset{1}{\overset{2}{\int }}\frac{\sin \left(\frac{n\pi }{3}\right)t}{\left(\frac{n\pi }{3}\right)}dt}\\ =\frac{3}{n\pi }\left[\left(2\right)\sin \left(\frac{n\pi }{3}\right)\left(2\right)-\left(1\right)\sin \left(\frac{n\pi }{3}\right)\left(1\right)\right]-\frac{3}{n\pi }{\displaystyle \underset{1}{\overset{2}{\int }}\sin \left(\frac{n\pi }{3}\right)tdt}\\ =\frac{3}{n\pi }\left[2\sin \left(\frac{2n\pi }{3}\right)-\sin \left(\frac{n\pi }{3}\right)\right]-\frac{3}{n\pi }{\left[-\frac{\cos \left(\frac{n\pi }{3}\right)t}{\left(\frac{n\pi }{3}\right)}\right]}_1^2\end{array}$

Simplify the above equation to find $x$.

$\begin{array}{c}x=\frac{3}{n\pi }\left[2\sin \left(\frac{2n\pi }{3}\right)-\sin \left(\frac{n\pi }{3}\right)\right]+\frac{3}{n\pi }\left(\frac{3}{n\pi }\right)\left[\cos \left(\frac{n\pi }{3}\right)\left(2\right)-\cos \left(\frac{n\pi }{3}\right)\left(1\right)\right]\\ =\frac{6}{n\pi }\sin \left(\frac{2n\pi }{3}\right)-\frac{3}{n\pi }\sin \left(\frac{n\pi }{3}\right)+\frac{9}{n^2{\pi }^2}\cos \left(\frac{2n\pi }{3}\right)-\frac{9}{n^2{\pi }^2}\cos \left(\frac{n\pi }{3}\right)\end{array}$

Substitute $\left(\frac{6}{n\pi }\sin \left(\frac{2n\pi }{3}\right)-\frac{3}{n\pi }\sin \left(\frac{n\pi }{3}\right)+\frac{9}{n^2{\pi }^2}\cos \left(\frac{2n\pi }{3}\right)-\frac{9}{n^2{\pi }^2}\cos \left(\frac{n\pi }{3}\right)\right)$ for $x$ in equation (7) to find $a_n$.

$\begin{array}{c}{a}_n=\frac{2}{3}\left(\begin{array}{l}10\left(\frac{6}{n\pi }\sin \left(\frac{2n\pi }{3}\right)-\frac{3}{n\pi }\sin \left(\frac{n\pi }{3}\right)+\frac{9}{n^2{\pi }^2}\cos \left(\frac{2n\pi }{3}\right)-\frac{9}{n^2{\pi }^2}\cos \left(\frac{n\pi }{3}\right)\right)\\ -\frac{60}{n\pi }\sin \left(\frac{2n\pi }{3}\right)+\frac{30}{n\pi }\sin \left(\frac{n\pi }{3}\right)\end{array}\right)\\ =\frac{2}{3}\left(\begin{array}{l}\frac{60}{n\pi }\sin \left(\frac{2n\pi }{3}\right)-\frac{30}{n\pi }\sin \left(\frac{n\pi }{3}\right)+\frac{90}{n^2{\pi }^2}\cos \left(\frac{2n\pi }{3}\right)\\ -\frac{90}{n^2{\pi }^2}\cos \left(\frac{n\pi }{3}\right)-\frac{60}{n\pi }\sin \left(\frac{2n\pi }{3}\right)+\frac{30}{n\pi }\sin \left(\frac{n\pi }{3}\right)\end{array}\right)\\ =\frac{2}{3}\left(\frac{90}{n^2{\pi }^2}\right)\left(\cos \left(\frac{2n\pi }{3}\right)-\cos \left(\frac{n\pi }{3}\right)\right)\\ =\frac{60}{n^2{\pi }^2}\left(\cos \left(\frac{2n\pi }{3}\right)-\cos \left(\frac{n\pi }{3}\right)\right)\end{array}$

Substitute $5$ for $a_0$, $\frac{60}{n^2{\pi }^2}\left(\cos \left(\frac{2n\pi }{3}\right)-\cos \left(\frac{n\pi }{3}\right)\right)$ for $a_n$, $0$ for $b_n$, and $\frac{\pi }{3}$ for ${\omega }_0$ in equation (2) to find $f\left(t\right)$.

$\begin{array}{c}f\left(t\right)=5+{\displaystyle \sum _{n=1}^{\infty }\left(\left(\frac{60}{n^2{\pi }^2}\left(\cos \left(\frac{2n\pi }{3}\right)-\cos \left(\frac{n\pi }{3}\right)\right)\right)\cos n\left(\frac{\pi }{3}\right)t+\left(0\right)\sin n\left(\frac{\pi }{3}\right)t\right)}\\ =5+{\displaystyle \sum _{n=1}^{\infty }\left(\left(\frac{60}{n^2{\pi }^2}\left(\cos \left(\frac{2n\pi }{3}\right)-\cos \left(\frac{n\pi }{3}\right)\right)\right)\cos \left(\frac{n\pi t}{3}\right)+0\right)}\\ =5+{\displaystyle \sum _{n=1}^{\infty }\left(\frac{60}{n^2{\pi }^2}\left(\cos \left(\frac{2n\pi }{3}\right)-\cos \left(\frac{n\pi }{3}\right)\right)\right)\cos \left(\frac{n\pi t}{3}\right)}\end{array}$

$f\left(t\right)=5+{\displaystyle \sum _{n=1}^{\infty }\frac{60}{{\pi }^2{n}^2}\left(\cos \left(\frac{2n\pi }{3}\right)-\cos \left(\frac{n\pi }{3}\right)\right)\cos \left(\frac{n\pi t}{3}\right)}$        (9)

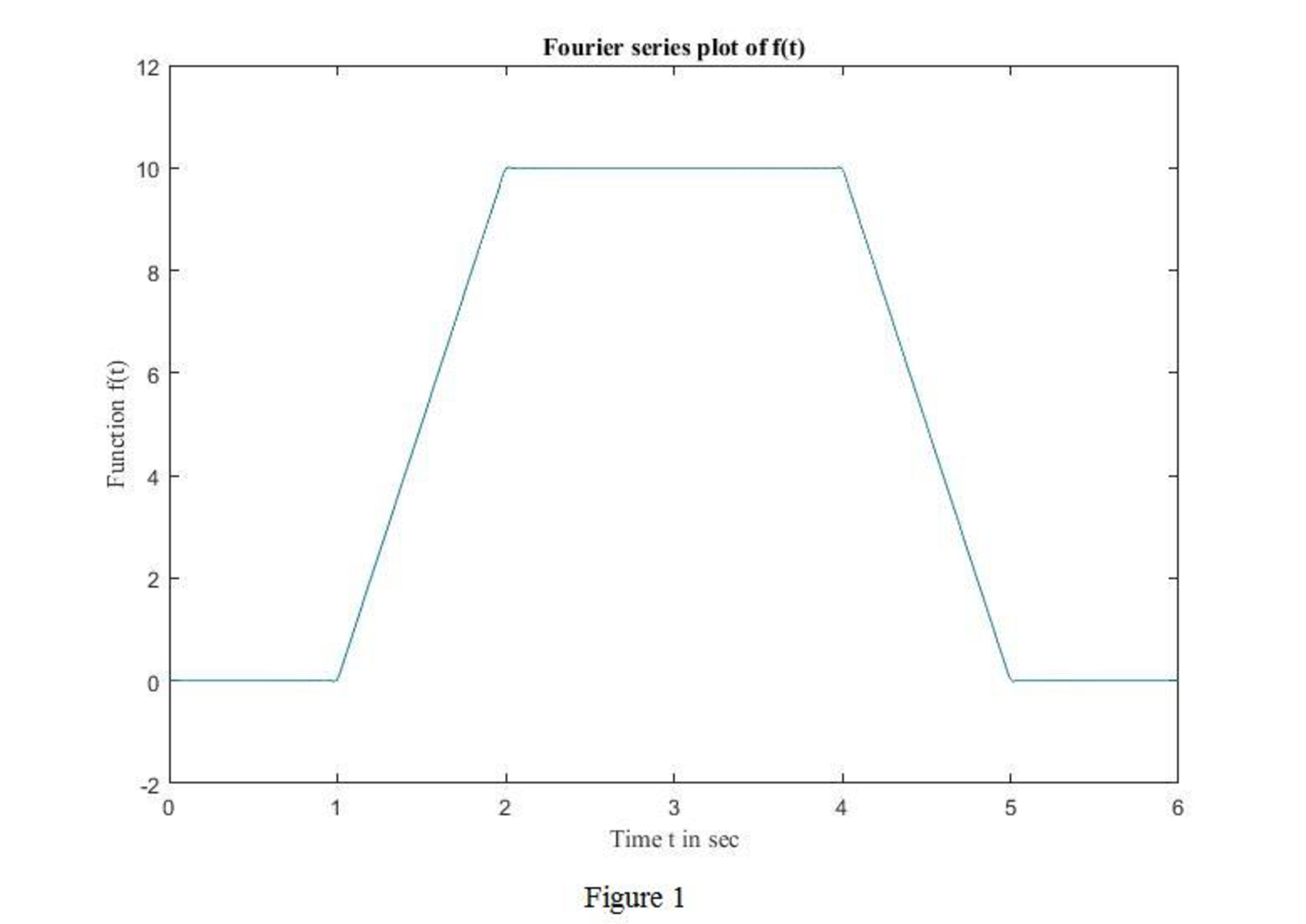
Following includes the MATLAB code to obtain the waveform in Figure 1 using the obtained function $f\left(t\right)$.

MATLAB Code:

t=0:.01:6;

f=5*ones(size(t));

for n=1:1:99

   f=f+(60/(n^2*pi^2))*(cos(2*pi*n/3)-cos(pi*n/3))*cos(n*pi*t/3);

end

plot(t,f)

title('Fourier series plot of f(t)');

xlabel('Time t in sec');

ylabel('Function f(t)');

Output:

The output of the MATLAB is the Fourier series plot of the function $f\left(t\right)$, is shown in below Figure 1.

Substitute $2$ for $t$ in equation (9) to find $f\left(2\right)$.

$\begin{array}{c}f\left(2\right)=5+\frac{60}{{\pi }^2}{\displaystyle \sum _{n=1}^{\infty }\left(\frac{1}{n^2}\left(\cos \left(\frac{2n\pi }{3}\right)-\cos \left(\frac{n\pi }{3}\right)\right)\right)\cos \left(\frac{2n\pi }{3}\right)}\\ =5+\frac{60}{{\pi }^2}\left(\begin{array}{l}\left(\frac{1}{{\left(1\right)}^2}\left(\cos \left(\frac{2\left(1\right)\pi }{3}\right)-\cos \left(\frac{\left(1\right)\pi }{3}\right)\right)\right)\cos \left(\frac{2\left(1\right)\pi }{3}\right)\\ +\left(\frac{1}{{\left(2\right)}^2}\left(\cos \left(\frac{2\left(2\right)\pi }{3}\right)-\cos \left(\frac{\left(2\right)\pi }{3}\right)\right)\right)\cos \left(\frac{2\left(2\right)\pi }{3}\right)\\ +\left(\frac{1}{{\left(3\right)}^2}\left(\cos \left(\frac{2\left(3\right)\pi }{3}\right)-\cos \left(\frac{\left(3\right)\pi }{3}\right)\right)\right)\cos \left(\frac{2\left(3\right)\pi }{3}\right)\\ +\left(\frac{1}{{\left(4\right)}^2}\left(\cos \left(\frac{2\left(4\right)\pi }{3}\right)-\cos \left(\frac{\left(4\right)\pi }{3}\right)\right)\right)\cos \left(\frac{2\left(4\right)\pi }{3}\right)\\ +\left(\frac{1}{{\left(5\right)}^2}\left(\cos \left(\frac{2\left(5\right)\pi }{3}\right)-\cos \left(\frac{\left(5\right)\pi }{3}\right)\right)\right)\cos \left(\frac{2\left(5\right)\pi }{3}\right)+\cdots \end{array}\right)\\ \\ =5+\frac{60}{{\pi }^2}\left(\begin{array}{l}\left(\left(\cos \left(\frac{2\pi }{3}\right)-\cos \left(\frac{\pi }{3}\right)\right)\right)\cos \left(\frac{2\pi }{3}\right)\\ +\left(\frac{1}{4}\left(\cos \left(\frac{4\pi }{3}\right)-\cos \left(\frac{2\pi }{3}\right)\right)\right)\cos \left(\frac{4\pi }{3}\right)\\ +\left(\frac{1}{9}\left(\cos 2\pi -\cos \pi \right)\right)\cos 2\pi \\ +\left(\frac{1}{16}\left(\cos \left(\frac{8\pi }{3}\right)-\cos \left(\frac{4\pi }{3}\right)\right)\right)\cos \left(\frac{8\pi }{3}\right)\\ +\left(\frac{1}{25}\left(\cos \left(\frac{10\pi }{3}\right)-\cos \left(\frac{5\pi }{3}\right)\right)\right)\cos \left(\frac{10\pi }{3}\right)+\cdots \end{array}\right)\\ \\ =5+6.07927\left(0.5+0+0.2222+0+0.02+\cdots \right)\end{array}$

Simplify the above equation to find $f\left(2\right)$.

$\begin{array}{c}f\left(2\right)=5+6.07927\left(0.7422\right)\\ =9.512\end{array}$

Write the MATLAB code to find $f\left(2\right)$ as follows.

MATLAB code:

t=2;

f=5*ones(size(t));

for n=1:1:5

   f=f+(60/(n^2*pi^2))*(cos(2*pi*n/3)-cos(pi*n/3))*cos(n*pi*t/3);

end

disp(f)

The Output displays the value of $f\left(2\right)$ as,

9.5122

This output is approximately satisfied with calculated value of $f\left(2\right)$.

Conclusion:

Thus, the Fourier series $f\left(t\right)$ for the signal in Figure 17.58 is $5+\frac{60}{{\pi }^2}{\displaystyle \sum _{n=1}^{\infty }\left(\frac{1}{n^2}\left(\cos \left(\frac{2n\pi }{3}\right)-\cos \left(\frac{n\pi }{3}\right)\right)\right)\cos \left(\frac{n\pi }{3}\right)t}$ and it is verified using MATLAB and the value of $f\left(t\right)$ at $t=2$ $f\left(2\right)$ is $9.512$.