
Concept explainers
Review. A 150-g glider moves at v1 = 2.30 m/s on an air track toward an originally stationary 200-g glider as shown in Figure P16.53. The gliders undergo a completely inelastic collision and latch together over a time interval of 7.00 ms. A student suggests roughly half the decrease in mechanical energy of the two-glider system is transferred to the environment by sound. Is this suggestion reasonable? To evaluate the idea, find the implied sound level at a position 0.800 m from the gliders. If the student’s idea is unreasonable, suggest a better idea.
Figure P16 53
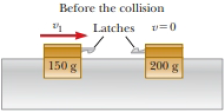
The idea and find the implied sound level at the position
Answer to Problem 17.57AP
It is unreasonable, implying a sound level
Explanation of Solution
The mass of first glider is
The glider stick together and moved with final speed momentum conservation for the two sliders system.
Formula to calculate the momentum of the two glider systems before collision is,
Here,
The velocity of the second slider systemis zero after collision so, formula to calculte the momentum of the two glider systems after collision is,
Here,
From momentum conservation system, the momentum of the two sliders system before collision and after collision is equal.
Substitute
Since, the velocities of the two sliders systems are equal before collision. Hence, the equation (2) can be written as,
Substitute
Formula to calculate the missing kinetic energy is,
Substitute
Imagine that one half of
Formula to calculate the intensity is,
Here,
Formula to calculate the area of the area of the cross section is,
Here,
Substitute
Substitute
Formula to calculate the intensity level is,
Here,
Substitute
It is unreasonable, implying a sound level
Conclusion:
Therefore, it is unreasonable, implying a sound level
Want to see more full solutions like this?
Chapter 17 Solutions
PHYSICS 1250 PACKAGE >CI<
Additional Science Textbook Solutions
General, Organic, and Biological Chemistry: Structures of Life (5th Edition)
Campbell Biology (11th Edition)
Human Biology: Concepts and Current Issues (8th Edition)
Introductory Chemistry (6th Edition)
Microbiology Fundamentals: A Clinical Approach
Fundamentals Of Thermodynamics
- Question B3 Consider the following FLRW spacetime: t2 ds² = -dt² + (dx² + dy²+ dz²), t2 where t is a constant. a) State whether this universe is spatially open, closed or flat. [2 marks] b) Determine the Hubble factor H(t), and represent it in a (roughly drawn) plot as a function of time t, starting at t = 0. [3 marks] c) Taking galaxy A to be located at (x, y, z) = (0,0,0), determine the proper distance to galaxy B located at (x, y, z) = (L, 0, 0). Determine the recessional velocity of galaxy B with respect to galaxy A. d) The Friedmann equations are 2 k 8πG а 4πG + a² (p+3p). 3 a 3 [5 marks] Use these equations to determine the energy density p(t) and the pressure p(t) for the FLRW spacetime specified at the top of the page. [5 marks] e) Given the result of question B3.d, state whether the FLRW universe in question is (i) radiation-dominated, (ii) matter-dominated, (iii) cosmological-constant-dominated, or (iv) none of the previous. Justify your answer. f) [5 marks] A conformally…arrow_forwardSECTION B Answer ONLY TWO questions in Section B [Expect to use one single-sided A4 page for each Section-B sub question.] Question B1 Consider the line element where w is a constant. ds²=-dt²+e2wt dx², a) Determine the components of the metric and of the inverse metric. [2 marks] b) Determine the Christoffel symbols. [See the Appendix of this document.] [10 marks] c) Write down the geodesic equations. [5 marks] d) Show that e2wt it is a constant of geodesic motion. [4 marks] e) Solve the geodesic equations for null geodesics. [4 marks]arrow_forwardPage 2 SECTION A Answer ALL questions in Section A [Expect to use one single-sided A4 page for each Section-A sub question.] Question A1 SPA6308 (2024) Consider Minkowski spacetime in Cartesian coordinates th = (t, x, y, z), such that ds² = dt² + dx² + dy² + dz². (a) Consider the vector with components V" = (1,-1,0,0). Determine V and V. V. (b) Consider now the coordinate system x' (u, v, y, z) such that u =t-x, v=t+x. [2 marks] Write down the line element, the metric, the Christoffel symbols and the Riemann curvature tensor in the new coordinates. [See the Appendix of this document.] [5 marks] (c) Determine V", that is, write the object in question A1.a in the coordinate system x'. Verify explicitly that V. V is invariant under the coordinate transformation. Question A2 [5 marks] Suppose that A, is a covector field, and consider the object Fv=AAμ. (a) Show explicitly that F is a tensor, that is, show that it transforms appropriately under a coordinate transformation. [5 marks] (b)…arrow_forward
- No chatgpt pls will upvote Iarrow_forwardHow would partial obstruction of an air intake port of an air-entrainment mask effect FiO2 and flow?arrow_forward14 Z In figure, a closed surface with q=b= 0.4m/ C = 0.6m if the left edge of the closed surface at position X=a, if E is non-uniform and is given by € = (3 + 2x²) ŷ N/C, calculate the (3+2x²) net electric flux leaving the closed surface.arrow_forward
- No chatgpt pls will upvotearrow_forwardsuggest a reason ultrasound cleaning is better than cleaning by hand?arrow_forwardCheckpoint 4 The figure shows four orientations of an electric di- pole in an external electric field. Rank the orienta- tions according to (a) the magnitude of the torque on the dipole and (b) the potential energy of the di- pole, greatest first. (1) (2) E (4)arrow_forward
 Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning





