Chapter 16.2, Problem 16.138P

Solve Prob. 16.137 when $\theta =90°$ .

To determine

The forces exerted on the connecting rod at points B and D.

Explanation of Solution

Given information:

Connecting rod length, $l=250mm$

Crank length $b=100mm$

Rod mass, $m=1.2kg$

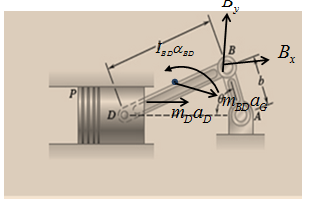
Position vector,$r_{B/A}=0.1j$

Points D and A horizontal distance,

$x=\sqrt{l^2-b^2}$

$=\sqrt{{\left(250\right)}^2-{\left(100\right)}^2}$

$x=0.229m$

Position vector $r_{D/B}$ ,

$r_{D/B}=-\left(0.229\right)i-\left(0.1\right)j$

Crank angular velocity in the form of vector,

${\omega }_{AB}=-600k$

Point B velocity,

$v_B={\omega }_{AB}\times r_{B/A}$

$v_B=\left(-600\times \frac{2\pi }{60}\right)k\times 0.1j$

$=-62.83k\times 0.1j$

$v_B=-\left(6.283\right)\left(k\times j\right)$

$v_B=\left(6.283m/s\right)i$

Point B angular velocity in the form of vector is ${\omega }_{BD}k$

Point D velocity is given by,

$v_D=v_B+\left({\omega }_{BD}\times r_{D/B}\right)$

$v_D=v_{D}i$

${\omega }_{BD}={\omega }_{BD}k$

$v_{D}i=6.283i+\left({\omega }_{BD}k\times -\left(0.229\right)i-\left(0.1\right)j\right)$

$v_{D}i=6.283i-\left(0.229{\omega }_{BD}\right)\left(k\times i\right)-\left(0.1{\omega }_{BD}\right)\left(k\times j\right)$ …………..(Equation A)

$v_{D}i=6.283i-\left(0.229{\omega }_{BD}\right)j-\left(0.1{\omega }_{BD}\right)i$

Compare j terms from equation A,

$0=-\left(0.229{\omega }_{BD}\right)$

${\omega }_{BD}=0$

Compare i terms from equation A,

$v_D=6.283+0.1{\omega }_{BD}$

$v_D=6.283+0.1\times 0$

$v_D=6.283m/s$

Crank AB angular acceleration is zero i.e. ${\alpha }_{AB}=0$

Vector form of point B relative angular acceleration is ${\alpha }_{BD}k$

Vector form of point D acceleration is $a_{D}i$

Point B acceleration,

$a_B={\alpha }_{AB}{r}_{B/A}-{\omega }^2{}_{AB}{r}_{B/A}$

$a_B=-{\left(\left(-600\times \frac{2\pi }{60}\right)k\right)}^2\times 0.1j$

$a_B=-\left(394.78m/s^2\right)j$

Point D acceleration,

$a_D=a_B+{\alpha }_{BD}\times r_{D/B}-{\omega }^2{}_{BD}\times r_{D/B}$

Here, $\begin{array}{l}{a}_D=a_{D}i\\ {\alpha }_{BD}={\alpha }_{BD}k\\ {\omega }_{BD}=0\end{array}$

$a_{D}i=\left\{-\left(394.78\right)j+\left({\alpha }_{BD}k\times -\left(0.229\right)i-\left(0.1\right)j\right)-0\right\}$

$a_{D}i=-\left(394.78\right)j-\left(\left(0.229{\alpha }_{BD}\right)\left(k\times i\right)-\left(0.1{\alpha }_{BD}\right)\left(k\times j\right)\right)$

$a_{D}i=-\left(394.78\right)j-\left(\left(0.229{\alpha }_{BD}\right)j+\left(0.1{\alpha }_{BD}\right)i\right)$ ………… (Equation B)

Compare j terms in equation B,

$0=-\left(394.78\right)-\left(0.229{\alpha }_{BD}\right)$

${\alpha }_{BD}=\frac{-394.78}{0.229}$

${\alpha }_{BD}=-1,723.19rad/s^2$

Vector form of angular acceleration of connecting rod BD is

${\alpha }_{BD}=-\left(1,723.19rad/s^2\right)k$

Compare i terms in equation B,

$a_D=0.1{\alpha }_{BD}$

$a_D=0.1\times -1,723.19$

$a_D=-172.319m/s^2$

Vector form of acceleration of point D is $a_D=-\left(172.319m/s^2\right)i$

$r_{G/D}$ position vector is $r_{G/D}=0.1145i+0.05j$

Pont G acceleration,

$a_G=a_D+{\alpha }_{BD}\times r_{G/D}-{\omega }^2{}_{BD}\times r_{G/D}$

Here, $\begin{array}{l}{a}_D=-172.31i\\ r_{G/D}=0.1145i+0.05j\\ {\alpha }_{BD}=1723.19k\\ {\omega }_{BD}=0\end{array}$

$a_G=-172.31i+\left(1723.19k\times 0.1145i+0.05j\right)$

$a_G=-172.31i+\left(197.34\left(k\times i\right)-86.159\left(k\times j\right)\right)$

$a_G=-172.31i+\left(197.34j-86.159i\right)$

$a_G=86.159i-197.34j$

Point G inertial force,

$F_G=m_{BD}{a}_G$

Here, $m_{BD}=1.2kg$

$F_G=1.2\times \left(86.159i-197.34j\right)$

Force horizontal component from figure 2,

$B_x=m_D{a}_D+{\left(m_{BD}{a}_G\right)}_x$

Here, $m_D=1.8kg$

$B_x=\left(1.8\right)\left(-172.319\right)-103.391$

$B_x=-413.52N$

Connected rod BD moment of inertia,

$I_{BD}=\frac{m_{BD}{l}^2}{12}$

$I_{BD}=\frac{1.2\times {\left(0.25\right)}^2}{12}$

$I_{BD}=0.00625kg.m^2$

Moment at B from figure 2,

$\left(-xN\right)k=\left(I_{BD}{\alpha }_{BD}\right)k+r_{D/B}\times \left(m_D{a}_D\right)+r_{G/D}\times \left(m_{BD}{a}_G\right)$

Here, $\begin{array}{l}{I}_{BD}=0.00625kg.m^2\\ {\alpha }_{BD}=-1,723.19rad/s^2\\ r_{D/B}=-\left(0.229\right)i-\left(0.1\right)j\\ m_D=1.8kg\\ a_D=-172.31i\\ r_{G/D}=0.1145i+0.05j\\ m_{BD}{a}_G=103.39i-236.808j\end{array}$

$\begin{array}{l}\left(-0.229\times N\right)k=\left(0.00625\times -1,723.19\right)k+\left(-\left(0.229\right)i-\left(0.1\right)j\right)\left(1.8\right)\left(-172.31i\right)+\left(0.1145i+0.05j\right)\\ \times \left(103.39i-236.808j\right)\end{array}$ ……..(Equation C)

Compare K terms from equation C,

$-0.229N=-19.918$

$N=86.92N$

Force vertical component from figure 2,

$N+B_y={\left(m_{BD}{a}_G\right)}_y$

${\left(m_{BD}{a}_G\right)}_y=-236.808$

$86.92N+B_y=-236.808$

$B_y=-323.8N$

Point B resultant reaction,

$B_R=\sqrt{B^2{}_x+B^2{}_y}$

Here, $B_x=-413.52N$

$B_R=\sqrt{{\left(-413.52N\right)}^2+{\left(-323.8N\right)}^2}$

$B_R=525N$

Resultant angle,

$\varphi ={\tan }^{-1}\left(\frac{B_y}{B_x}\right)$

$\varphi ={\tan }^{-1}\left(\frac{-323.8N}{-413.52N}\right)$

$\varphi =38.1°$

The force exerted at point B is $B_R=525N$ at an angle of $\varphi =38.1°$

Forces along connecting rod from figure 3,

$F_D+Nj=\left(m_D{a}_D\right)i$

$F_D+\left(86.92N\right)j=1.8\times \left(-172.319\right)i$

$F_D=-\left(310.14N\right)i-\left(86.92N\right)j$

Hence, piston exerts force in rod which is $-F_D$ i.e.

$F_D\text{'}=\left(310.14N\right)i+\left(86.92N\right)j$

Point D resultant reaction,

${\left(F_D\right)}_R=\sqrt{{\left(310.14N\right)}^2+{\left(86.92N\right)}^2}$

${\left(F_D\right)}_R=322N$

Resultant angle,

$\beta ={\tan }^{-1}\left(\frac{86.92N}{310.14N}\right)$

$\beta =15.7°$

The force exerted at point D is ${\left(F_D\right)}_R=322N$ at an angle of $\beta =15.7°$

Conclusion:

The force exerted at point B is $B_R=525N.$

The force exerted at point D is ${\left(F_D\right)}_R=322N.$