Vector Mechanics for Engineers: Statics and Dynamics
12th Edition
ISBN: 9781259977251
Author: BEER
Publisher: MCG
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 14.2, Problem 14.55P
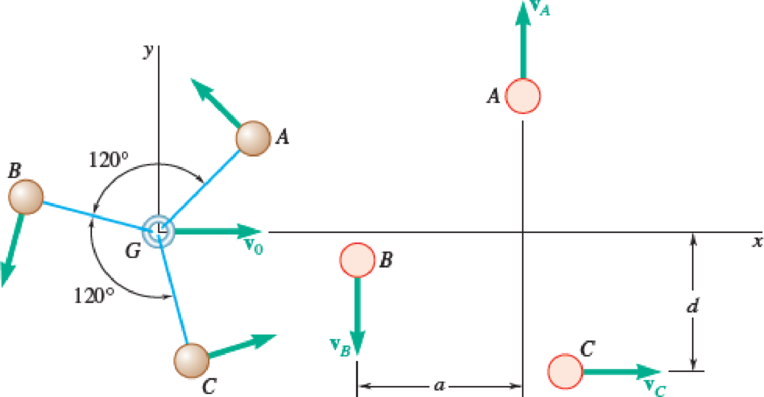
Three small identical spheres A, B, and C, which can slide on a horizontal, frictionless surface, are attached to three strings of length l, which are tied to a ring G. Initially the spheres rotate about the ring, which moves along the x axis with a velocity v0. Suddenly the ring breaks, and the three spheres move freely in the xy plane. Knowing that vA = (8.66 ft/s)j, vC = (15 ft/s)i, a = 0.866 ft, and d = 0.5 ft, determine (a) the initial velocity of the ring, (b) the length l of the strings, (c) the rate in rad/s at which the spheres were rotating about G.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Slider C has a mass of 0.5 kg and
may move in a slot cut in arm AB,
which rotates at constant speed in a
horizontal plane. The slider is
attached to a spring of constant
k = 150 N/m, which is unstretched
when r = 0.
When arm AB rotates about the
vertical axis, the slider moves
without friction outward along the
smooth slot cut. Determine for the
position r = 80 mm:
a) The constant speed (V) of the
slider.
b) The normal force (N) exerted on
the slider by arm AB.
A
r=80mm
B
2) A ball with a weight of 0.3N is attached to the end of an elastic thin cord of which unstretched
length is 59cm. The other end of the cord is fixed at a point on the horizontal frictionless surface
of a table. The stiffness of the cord is 20 N/m. At an instant, the ball moves with a velocity of
2.95 m/s which is perpendicular to the radius of curvature of the path of the ball and the length
of the cord is 89cm at that instant. For the elastic cord to be stretched all the time during the
motion of this ball, what is the minimum value of the velocity of the ball?
Kindly answer it as fast as you can.. and correctly.
Chapter 14 Solutions
Vector Mechanics for Engineers: Statics and Dynamics
Ch. 14.1 - A 30-g bullet is fired with a horizontal velocity...Ch. 14.1 - Two identical 1350-kg automobiles A and B are at...Ch. 14.1 - An airline employee tosses two suitcases in rapid...Ch. 14.1 - Car A weighing 4000 lb and car B weighing 3700 lb...Ch. 14.1 - Two swimmers A and B, of weight 190 lb and 125 lb,...Ch. 14.1 - A 180-lb man and a 120-lb woman stand side by side...Ch. 14.1 - A 40-Mg boxcar A is moving in a railroad...Ch. 14.1 - Two identical cars A and B are at rest on a...Ch. 14.1 - A 20-kg base satellite deploys three...Ch. 14.1 - For the satellite system of Prob. 14.9, assuming...
Ch. 14.1 - A system consists of three identical 19.32-lb...Ch. 14.1 - A system consists of three identical 19.32-lb...Ch. 14.1 - A system consists of three particles A, B, and C....Ch. 14.1 - For the system of particles of Prob. 14.13,...Ch. 14.1 - A 13-kg projectile is passing through the origin O...Ch. 14.1 - Prob. 14.16PCh. 14.1 - A 2-kg model rocket is launched vertically and...Ch. 14.1 - An 18-kg cannonball and a 12-kg cannonball are...Ch. 14.1 - 14.19 and 14.20 Cruiser A was traveling east at 60...Ch. 14.1 - 14.19 and 14.20 Cruiser A was traveling east at 60...Ch. 14.1 - Prob. 14.21PCh. 14.1 - Two spheres, each of mass m, can slide freely on a...Ch. 14.1 - In a game of pool, ball A is moving with a...Ch. 14.1 - Prob. 14.24PCh. 14.1 - Prob. 14.25PCh. 14.1 - In a scattering experiment, an alpha particle A is...Ch. 14.1 - Derive the relation HO=rmv+HG between the angular...Ch. 14.1 - Prob. 14.28PCh. 14.1 - Prob. 14.29PCh. 14.1 - Show that the relation MA=HA, where HA is defined...Ch. 14.2 - Determine the energy lost due to friction and the...Ch. 14.2 - In Prob. 14.3, determine the energy lost (a) when...Ch. 14.2 - Prob. 14.33PCh. 14.2 - Determine the energy lost as a result of the...Ch. 14.2 - Prob. 14.35PCh. 14.2 - Prob. 14.36PCh. 14.2 - Prob. 14.37PCh. 14.2 - Ball B is suspended from a cord of length l...Ch. 14.2 - A 15-lb block B starts from rest and slides on the...Ch. 14.2 - A 40-lb block B is suspended from a 6-ft cord...Ch. 14.2 - Prob. 14.41PCh. 14.2 - 14.41 and 14.42 In a game of pool, ball A is...Ch. 14.2 - Prob. 14.43PCh. 14.2 - In a game of pool, ball A is moving with the...Ch. 14.2 - Prob. 14.45PCh. 14.2 - Prob. 14.46PCh. 14.2 - Four small disks A, B, C, and D can slide freely...Ch. 14.2 - In the scattering experiment of Prob. 14.26, it is...Ch. 14.2 - Three identical small spheres, each weighing 2 lb,...Ch. 14.2 - Three small spheres A, B, and C, each of mass m,...Ch. 14.2 - Prob. 14.51PCh. 14.2 - Prob. 14.52PCh. 14.2 - Two small disks A and B of mass 3 kg and 1.5 kg,...Ch. 14.2 - Two small disks A and B of mass 2 kg and 1 kg,...Ch. 14.2 - Three small identical spheres A, B, and C, which...Ch. 14.2 - Prob. 14.56PCh. 14.3 - A stream of water with a density of = 1000 kg/m3...Ch. 14.3 - A jet ski is placed in a channel and is tethered...Ch. 14.3 - Tree limbs and branches are being fed at A at the...Ch. 14.3 - Prob. 14.60PCh. 14.3 - Prob. 14.61PCh. 14.3 - Prob. 14.62PCh. 14.3 - Prob. 14.63PCh. 14.3 - Prob. 14.64PCh. 14.3 - Prob. 14.65PCh. 14.3 - Prob. 14.66PCh. 14.3 - Prob. 14.67PCh. 14.3 - Prob. 14.68PCh. 14.3 - Prob. 14.69PCh. 14.3 - Prob. 14.70PCh. 14.3 - Prob. 14.71PCh. 14.3 - Prob. 14.72PCh. 14.3 - Prob. 14.73PCh. 14.3 - Prob. 14.74PCh. 14.3 - Prob. 14.75PCh. 14.3 - Prob. 14.76PCh. 14.3 - The propeller of a small airplane has a...Ch. 14.3 - Prob. 14.78PCh. 14.3 - Prob. 14.79PCh. 14.3 - Prob. 14.80PCh. 14.3 - Prob. 14.81PCh. 14.3 - Prob. 14.82PCh. 14.3 - Prob. 14.83PCh. 14.3 - Prob. 14.84PCh. 14.3 - Prob. 14.85PCh. 14.3 - Prob. 14.86PCh. 14.3 - Solve Prob. 14.86, assuming that the chain is...Ch. 14.3 - Prob. 14.88PCh. 14.3 - Prob. 14.89PCh. 14.3 - Prob. 14.90PCh. 14.3 - Prob. 14.91PCh. 14.3 - Prob. 14.92PCh. 14.3 - A rocket sled burns fuel at the constant rate of...Ch. 14.3 - Prob. 14.94PCh. 14.3 - Prob. 14.95PCh. 14.3 - Prob. 14.96PCh. 14.3 - Prob. 14.97PCh. 14.3 - Prob. 14.98PCh. 14.3 - Determine the distance traveled by the spacecraft...Ch. 14.3 - A rocket weighs 2600 lb, including 2200 lb of...Ch. 14.3 - Determine the altitude reached by the spacecraft...Ch. 14.3 - Prob. 14.102PCh. 14.3 - Prob. 14.103PCh. 14.3 - Prob. 14.104PCh. 14 - Three identical cars are being unloaded from an...Ch. 14 - A 50-kg mother and her 26-kg son are sledding down...Ch. 14 - An 80-Mg railroad engine A coasting at 6.5 km/h...Ch. 14 - Prob. 14.108RPCh. 14 - Mass C, which has a mass of 4 kg, is suspended...Ch. 14 - Prob. 14.110RPCh. 14 - A 6000-kg dump truck has a 1500-kg stone block...Ch. 14 - For the ceiling-mounted fan shown, determine the...Ch. 14 - Prob. 14.113RPCh. 14 - Prob. 14.114RPCh. 14 - Prob. 14.115RPCh. 14 - A chain of length l and mass m falls through a...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- Solve all sub parts of the question please.arrow_forwardASAParrow_forwardProblem 2.841 Cars A and B are traveling at V 72 mph and va 67 mph, respectively, when the driver of car B applies the brakes abruptly, causing the car to slide to a stop. The driver of car A takes 1.5s to react to the situation and applies the brakes in turn, causing car A to slide as well. If A and B slide with equal accelerations, i.e., SA = -g, where p = 0.83 is the kinetic friction coefficient and g is the acceleration of gravity, compute the minimum distance ď between A and B at the time B starts sliding to avoid a collision.arrow_forward
- a particle m suspended in a rigid container by two massless, inextensible strings. A force is applied to the container so that it accelerates to the right at a constant rate x¨. Determine the maximum value of x¨ for which both supporting strings remain taut.arrow_forwardCollision at an Angle To apply conservation of linear momentum in an inelastic collision. Two cars, both of mass m, collide and stick together. Prior to the collision, one car had been traveling north at a speed 2v, while the second was traveling in a southeastern direction at an angle ϕ with respect to the east-west direction and at a speed v. After the collision, the two-car system travels in a northeastern direction at an angle θ with respect to the north-south direction and at a speed v final. What is the angle θ (with respect to north) made by the velocity vector of the two cars after the collision? Express your answer in terms of ϕ. Your answer should contain an inverse trigonometric function.arrow_forwardCollision at an Angle To apply conservation of linear momentum in an inelastic collision. Two cars, both of mass m, collide and stick together. Prior to the collision, one car had been traveling north at a speed 2v, while the second was traveling in a southeastern direction at an angle ϕ with respect to the east-west direction and at a speed v. After the collision, the two-car system travels in a northeastern direction at an angle θ with respect to the north-south direction and at a speed v final. Find v final, the speed of the joined cars after the collision. Express your answer in terms of v and ϕ.arrow_forward
- A particle, of mass 9 kg, is attached to two identical springs. The other ends of the springs are attached to fixed points, A and B, which are 1.2 metres apart on a smooth horizontal surface. The springs have modulus of elasticity 45 N and natural length 0.4 m. The particle is released from rest at a distance of 0.5 metres from B and moves on the line AB. The midpoint of AB is C. At timet seconds after release, the displacement of the particle from C is x metres, where the direction from A to B is taken to be positive. www C www В (а) Show that the resultant force on the particle, at time t, is -225x newtons. (b) Hence show that the particle moves with simple harmonic motion. (c) State the period of this motion. (d) Find the speed of the particle when it is 0.05 metres from C. (e) Write down an expression for x in terms of t.arrow_forwardJourney through the Center of the Earth. A 1024-kg blue ball is dropped from an initial z-position of 2.3 x 106 m through the center of a planet with radius 7.6 x 106 m. If the mass of the planet is 33.9 x 1015 kg, measure the displacement of the ball at time t = 9 s?arrow_forward1. A wood block of mass mw = 200 g is projected from the ground at a speed vo = 35 m/s and at an angle 60 = 50°. When at height h= 24 m the wood block was hit by a bullet of mass m = 25 g which was moving at v= 220 m/s and at an angle 6 = 70°. After the collision, the bullet embedded itself in the block and move together and land at point A on the ground. Then they move on the ground that has coefficient of kinetic friction u: = 0.5 and start to compress a horizontal spring at point B. The distance between point À and B is 18 m and the spring constant k = 3x10? N/m. Right end of the spring is fixed, and all motions are confined in x-y plane. Wood block + Bullet Bullet Wood B block (a) Was the collision between the wood block and the bullet elastic or inelastic? Explain quantitively. (b) Find the maximum height from the ground the block with the bullet reached. (c) How far is point A from the launch point of the wood block? (d) Calculate the velocity at which the block-bullet system…arrow_forward
- Three small spheres A, B, and C , each of mass m , are connected to a small ring D of negligible mass by means of three inextensible, inelastic cords of length I . The spheres can slide freely on a frictionless horizontal surface and are rotating initially at a speed v0 about ring D which is at rest. Suddenly the cord CD breaks. After the other two cords have again become taut, determine (C ) the speed of ring D, (b) the relative speed at which spheres A and B rotate about D , (c) the fraction of the original energy of spheres A and B that is dissipated when cords AD and BD again became taut.arrow_forwardA particle of mass 2.6 kg is attached to two cables CB and CA as shown. it revolves in a horizontal circle of radius 1.3 m at a constant speed of 8.45 m/s.arrow_forwardIn slow pitch softball, the underhand pitch must reach a maximum height of between 1.8 m and 3.7 m above the ground. A pitch is made with an initial velocity vo with a magnitude of 13 m/s at an angle of 33° with the horizontal. Determine (a) if the pitch meets the maximum height requirement, (b) the height of the ball as it reaches the batter.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Elements Of Electromagnetics
Mechanical Engineering
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Oxford University Press
Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:9780134319650
Author:Russell C. Hibbeler
Publisher:PEARSON
Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:9781259822674
Author:Yunus A. Cengel Dr., Michael A. Boles
Publisher:McGraw-Hill Education
Control Systems Engineering
Mechanical Engineering
ISBN:9781118170519
Author:Norman S. Nise
Publisher:WILEY
Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning
Engineering Mechanics: Statics
Mechanical Engineering
ISBN:9781118807330
Author:James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:WILEY
Dynamics - Lesson 1: Introduction and Constant Acceleration Equations; Author: Jeff Hanson;https://www.youtube.com/watch?v=7aMiZ3b0Ieg;License: Standard YouTube License, CC-BY