Chapter 14, Problem 14.17P

Interpretation Introduction

(a)

Interpretation: The number of peaks present in the given NMR signal of labeled proton is to be calculated.

Concept introduction: In NMR spectrum, peaks are known as resonances, lines or absorptions. The number of NMR signal in a compound is equal to the number of chemically non-equivalent protons present in that compound. In ${}^1\text{H}-\text{NMR}$ all chemically equivalent protons generates one signal or one peak, whereas non-equivalent proton generates different signals. The number of peaks is calculated by the formula,

$P=n+1$

Answer to Problem 14.17P

The labeled proton splits into septet in NMR spectrum of the given compound.

Explanation of Solution

The given compound is . The labeled proton is bonded to a carbon atom that has two methyl groups that has six hydrogen atoms. The number of peaks is calculated by the formula,

$P=n+1$

Where,

➢   $P$ is the number of peaks.

➢   $n$ is the number of protons present on the adjacent carbon atoms.

Here, $n=6$.

Substitute the value of $n=6$ in the above formula to calculate the number of peaks.

$\begin{array}{rll}P& =& 6+1\\ & =& 7\end{array}$

Hence, the labeled proton gives seven peaks in NMR spectrum.

Conclusion

The labeled proton splits into septet in NMR spectrum of the given compound.

Interpretation Introduction

(b)

Interpretation: The number of peaks present in the given NMR signal of labeled proton is to be calculated.

Concept introduction: In NMR spectrum, peaks are known as resonances, lines or absorptions. The number of NMR signal in a compound is equal to the number of chemically non-equivalent protons present in that compound. In ${}^1\text{H}-\text{NMR}$ all chemically equivalent protons generates one signal or one peak, whereas non-equivalent proton generates different signals. The number of peaks is calculated by the formula,

$P=n+1$

Answer to Problem 14.17P

The labeled proton (a), (b), and (c) splits into triplet, multiplet and quintet in NMR spectrum of the given compound.

Explanation of Solution

The given compound is $\text{C}{\underset{\text{a}}{\text{H}}}_{\text{3}}-\text{C}{\underset{\text{b}}{\text{H}}}_{\text{2}}-\text{C}{\underset{\text{c}}{\text{H}}}_2-{\text{CH}}_{\text{2}}-{\text{CH}}_{\text{3}}$. There are three labeled protons, (a), (b), and (c). Proton (a) is bonded to a methylene group that has two hydrogen atoms. The number of peaks is calculated by the formula,

$P=n+1$

Where,

➢   $P$ is the number of peaks.

➢   $n$ is the number of protons present on the adjacent carbon atoms.

Here, $n=2$.

Substitute the value of $n=2$ in the above formula to calculate the number of peaks.

$\begin{array}{rll}P& =& 2+1\\ & =& 3\end{array}$

Hence, the labeled proton (a) gives three peaks (triplet) in NMR spectrum.

Proton (b) is bonded to one methylene group that has two hydrogen atoms and one methyl group that has three hydrogen atoms. The number of peaks is calculated by the formula,

$P=\left(n+1\right)\left(m+1\right)$

Where,

➢   $P$ is the number of peaks.

➢   $n$ and $m$ are the number of protons present on the adjacent carbon atoms.

Here, $n=2$ and $m=3$.

Substitute the value of $n=2$ and $m=3$ in the above formula to calculate the number of peaks.

$\begin{array}{rll}P& =& \left(2+1\right)\left(3+1\right)\\ & =& 3\times 4\\ & =& 12\end{array}$

Hence, the labeled proton (b) splits into a multiplet in NMR spectrum.

Proton (c) is bonded to two methylene groups that have four hydrogen atoms. The number of peaks is calculated by the formula,

$P=n+1$

Where,

➢   $P$ is the number of peaks.

➢   $n$ is the number of protons present on the adjacent carbon atoms.

Here, $n=4$.

Substitute the value of $n=4$ in the above formula to calculate the number of peaks.

$\begin{array}{rll}P& =& 4+1\\ & =& 5\end{array}$

Hence, the labeled proton (c) gives quintet in NMR spectrum.

Therefore, protons (a), (b), and (c) give $3$, $12$ and $5$ peaks in NMR spectrum.

Conclusion

The labeled proton (a), (b), and (c) splits into triplet, multiplet and quintet in NMR spectrum of the given compound.

Interpretation Introduction

(c)

Interpretation: The number of peaks present in the given NMR signal of labeled proton is to be calculated.

Concept introduction: In NMR spectrum, peaks are known as resonances, lines or absorptions. The number of NMR signal in a compound is equal to the number of chemically non-equivalent protons present in that compound. In ${}^1\text{H}-\text{NMR}$ all chemically equivalent protons generates one signal or one peak, whereas non-equivalent proton generates different signals. The number of peaks is calculated by the formula,

$P=n+1$

Answer to Problem 14.17P

The labeled proton (a) and (b) splits into doublet and sextet in NMR spectrum of the given compound.

Explanation of Solution

The given compound is shown below.

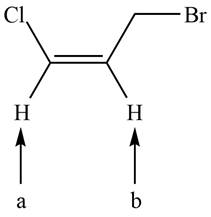
Figure 1

There are two labeled protons, (a) and (b). Proton (b) is bonded to a carbon atom that has one hydrogen and one methylene group. The number of peaks is calculated by the formula,

$P=\left(n+1\right)\left(m+1\right)$

Where,

➢   $P$ is the number of peaks.

➢   $n$ and $m$ are the number of protons present on the adjacent carbon atoms.

Here, $n=1$ and $m=2$.

Substitute the value of $n=1$ and $m=2$ in the above formula to calculate the number of peaks.

$\begin{array}{rll}P& =& \left(1+1\right)\left(2+1\right)\\ & =& 2\times 3\\ & =& 6\end{array}$

Hence, the labeled proton (b) splits into a sextet in NMR spectrum.

Proton (a) is bonded to a carbon atom that has only one hydrogen atom. The number of peaks is calculated by the formula,

$P=n+1$

Where,

➢   $P$ is the number of peaks.

➢   $n$ is the number of protons present on the adjacent carbon atoms.

Here, $n=1$.

Substitute the value of $n=1$ in the above formula to calculate the number of peaks.

$\begin{array}{rll}P& =& 1+1\\ & =& 2\end{array}$

Hence, the labeled proton (a) gives two peaks (doublet) in NMR spectrum.

Therefore, protons (a) and (b) give $2$ and $6$ peaks in NMR spectrum.

Conclusion

The labeled proton (a) and (b) splits into doublet and sextet in NMR spectrum of the given compound.

Interpretation Introduction

(d)

Interpretation: The number of peaks present in the given NMR signal of labeled proton is to be calculated.

Concept introduction: In NMR spectrum, peaks are known as resonances, lines or absorptions. The number of NMR signal in a compound is equal to the number of chemically non-equivalent protons present in that compound. In ${}^1\text{H}-\text{NMR}$ all chemically equivalent protons generates one signal or one peak, whereas non-equivalent proton generates different signals. The number of peaks is calculated by the formula,

$P=n+1$

Answer to Problem 14.17P

The labeled proton (a) and (b) splits into triplet, doublet and doublet in NMR spectrum of the given compound.

Explanation of Solution

The given compound is shown below.

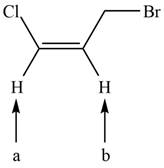
Figure 2

There are two labeled protons, (a) and (b). Proton (a) is bonded to a carbon atom that has two hydrogen atoms. The number of peaks is calculated by the formula,

$P=n+1$

Where,

➢   $P$ is the number of peaks.

➢   $n$ is the number of protons present on the adjacent carbon atoms.

Here, $n=2$.

Substitute the value of $n=2$ in the above formula to calculate the number of peaks.

$\begin{array}{rll}P& =& 2+1\\ & =& 3\end{array}$

Hence, the labeled proton (a) gives three peaks (triplet) in NMR spectrum.

Both protons (b) are bonded to a carbon atom that has only one hydrogen atom. The number of peaks is calculated by the formula,

$P=n+1$

Where,

➢   $P$ is the number of peaks.

➢   $n$ is the number of protons present on the adjacent carbon atoms.

Here, $n=1$.

Substitute the value of $n=1$ in the above formula to calculate the number of peaks.

$\begin{array}{rll}P& =& 1+1\\ & =& 2\end{array}$

Hence, the labeled protons (b) give two peaks (doublet) in NMR spectrum.

Therefore, protons (a) and (b) give $3$ and $2$ peaks in NMR spectrum.

Conclusion

The labeled proton (a) and (b) splits into triplet, doublet and doublet in NMR spectrum of the given compound.