
a.
To find: The slope of the curve at the given point.
a.
Answer to Problem 12E
Explanation of Solution
Given information:
The function is
Concept used:
Slope of any function f at any point a is given by:
Calculation:
Slope
Conclusion:
The slope of the curve at the given point is
b.
To find: the equation of the tangent line.
b.
Answer to Problem 12E
Explanation of Solution
Given information:
The function is
Concept used:
For the equation for the tangent line to
Then find the slope of the tangent line at
The equation of the tangent line is
Calculation:
From part (a) it is obtained that slope at
Find
Hence
Put
Hence the equation of tangent line is
c.
To find: The equation of the normal.
c.
Answer to Problem 12E
Explanation of Solution
Given information:
The function is
Concept used:
If the slope of a line is m, then the slope of the normal will be
Calculation:
For
Hence the equation of the normal is
d.
To graph: The curve, tangent line and the normal in the same square viewing window.
d.
Answer to Problem 12E
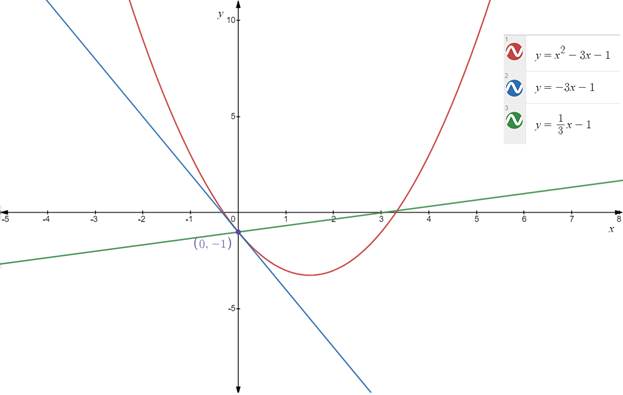
Explanation of Solution
Given information:
The function is
Graph:
Using graphing calculator:
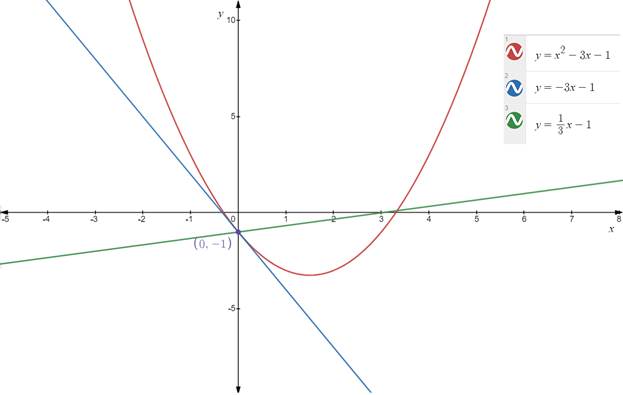
Interpretation:
From the graph it is clear that all the curve passes through the point
Chapter 1 Solutions
AP CALCULUS TEST PREP-WORKBOOK
- Let g(z) = z-i z+i' (a) Evaluate g(i) and g(1). (b) Evaluate the limits lim g(z), and lim g(z). 2-12 (c) Find the image of the real axis under g. (d) Find the image of the upper half plane {z: Iz > 0} under the function g.arrow_forwardk (i) Evaluate k=7 k=0 [Hint: geometric series + De Moivre] (ii) Find an upper bound for the expression 1 +2x+2 where z lies on the circle || z|| = R with R > 10. [Hint: Use Cauchy-Schwarz]arrow_forward21. Determine for which values of m the function (x) = x™ is a solution to the given equation. a. 3x2 d²y dx² b. x2 d²y +11x dy - 3y = 0 dx dy dx2 x dx 5y = 0arrow_forward
- Question Find the following limit. Select the correct answer below: 1 2 0 4 5x lim sin (2x)+tan 2 x→arrow_forward12. [0/1 Points] DETAILS MY NOTES SESSCALCET2 5.5.022. Evaluate the indefinite integral. (Use C for the constant of integration.) sin(In 33x) dxarrow_forward2. [-/1 Points] DETAILS MY NOTES SESSCALCET2 5.5.003.MI. Evaluate the integral by making the given substitution. (Use C for the constant of integration.) x³ + 3 dx, u = x² + 3 Need Help? Read It Watch It Master It SUBMIT ANSWER 3. [-/1 Points] DETAILS MY NOTES SESSCALCET2 5.5.006.MI. Evaluate the integral by making the given substitution. (Use C for the constant of integration.) | +8 sec² (1/x³) dx, u = 1/x7 Need Help? Read It Master It SUBMIT ANSWER 4. [-/1 Points] DETAILS MY NOTES SESSCALCET2 5.5.007.MI. Evaluate the indefinite integral. (Use C for the constant of integration.) √x27 sin(x28) dxarrow_forward
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning





