
(a.)
To explain: Why limit is restricted to
(a.)
Answer to Problem 77E
From the figure it can be observed that the graph of the function lies between
Explanation of Solution
Given:
The given figure is shown below:
Consider the given graph.
It is known that an output value that a function approaches for the specified input values is referred to as a limit.
From the given figure it can be observed that the value of the function or the limit of the function when x approaching to 0 is depends only for the positive x values near zero. This is why limit is restricted to
(b.)
To Prove: The area of
(b.)
Explanation of Solution
Given:
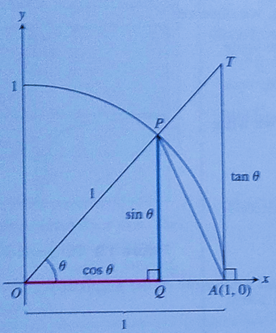
The given figure is shown below:
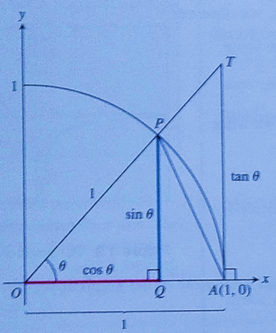
Concept used:
Area of triangle is:
Area of sector is:
Calculation:
Consider the given figure.
From the figure it can be observed that, in
Put
Consider the sector
From the figure it can be observed that, in sector
Use the area of the sector formula:
Consider the triangle
From the figure it can be observed that, in
Put
(c.)
To Prove: That
(c.)
Explanation of Solution
Given:
From part (b) The area of
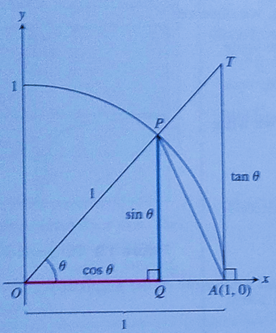
The given figure is shown below:
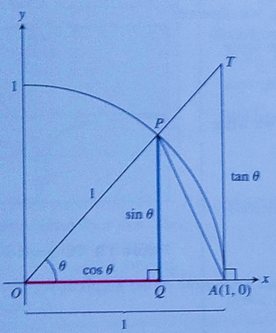
Concept used:
In a right-angled triangle one of its angles is
Calculation:
Consider the given information.
The triangle given in the figure is right angle triangle and in a right angle triangle one angle is
Also, it is known that the area of
Now observe the value of
Also, from the figure it can be observed that
Therefore,
(d.)
To Prove: That
(d.)
Explanation of Solution
Given:
The given inequality is:
Concept used:
Domain of a function is the set of input values which a function can take.
Calculation:
Consider the given inequality.
Mulitiply each side of the inequality by 2.
Now divide each side by
(e.)
To Prove: That
(e.)
Explanation of Solution
Given:
The given inequality is:
Concept used:
Domain of a function is the set of input values which a function can take.
Calculation:
Consider the given inequality.
Taking reciprocal of the above inequality and change the direction of the inequlaity.
(f.)
To Prove:
(f.)
Explanation of Solution
Given:
The given limit is
Concept used:
The squeeze theorem states that if
Calculation:
Consider the given information.
From part (e) it is known that
Applying limit on each function.
Let
Thus,
Let
Thus,
Therefore, by the squeeze theorem
(g.)
To Prove: That
(g.)
Explanation of Solution
Given:
The given function is
Concept used:
A function is said to be even if
Calculation:
Consider the given function.
Replace
Since
(h.)
To Prove: That
(h.)
Explanation of Solution
Given:
From part (g) it is known that
Concept used:
The squeeze theorem states that if
Calculation:
Consider the given limit.
Take
Therefore,
(i.)
To Prove:
(i.)
Explanation of Solution
Given:
From part (f) and (h) it is known that
Concept used:
The limit of a function as it approaches from the left is referred to as the left-hand limit. The limit of a function as it approaches from the right-hand side is referred to as a right-hand limit.
Calculation:
Consider the given information.
From part (f) and (h) it is known that
If the function has both limits defined at a particular x value c and those values match, then the limit will exist and will be equal to the value of the one-sided limits.
Since the left- and right-hand limits are equal so
Therefore,
Chapter 1 Solutions
AP CALCULUS TEST PREP-WORKBOOK
- EXAMPLE 3 Find S X √√2-2x2 dx. SOLUTION Let u = 2 - 2x². Then du = Χ dx = 2- 2x² = 信 du dx, so x dx = du and u-1/2 du (2√u) + C + C (in terms of x).arrow_forwardLet g(z) = z-i z+i' (a) Evaluate g(i) and g(1). (b) Evaluate the limits lim g(z), and lim g(z). 2-12 (c) Find the image of the real axis under g. (d) Find the image of the upper half plane {z: Iz > 0} under the function g.arrow_forwardk (i) Evaluate k=7 k=0 [Hint: geometric series + De Moivre] (ii) Find an upper bound for the expression 1 +2x+2 where z lies on the circle || z|| = R with R > 10. [Hint: Use Cauchy-Schwarz]arrow_forward
- Hint: You may use the following derivative rules: ddxsin(x)=cos(x) ddxcos(x)=−sin(x) ddxln(x)=1x Find the equation of the tangent line to the curve y=4sinx at the point (π6,2).The equation of this tangent line isarrow_forwardQuestion Find the following limit. Select the correct answer below: 1 2 0 4 5x lim sin (2x)+tan 2 x→arrow_forward12. [0/1 Points] DETAILS MY NOTES SESSCALCET2 5.5.022. Evaluate the indefinite integral. (Use C for the constant of integration.) sin(In 33x) dxarrow_forward
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning





