MyLab Statistics with Pearson eText -- Standalone Access Card -- for Elementary Statistics
13th Edition
ISBN: 9780134748535
Author: Mario F. Triola
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 13.4, Problem 7BSC
Wilcoxon Rank-Sum Test. In Exercises 5–8, use the Wilcoxon rank-sum test.
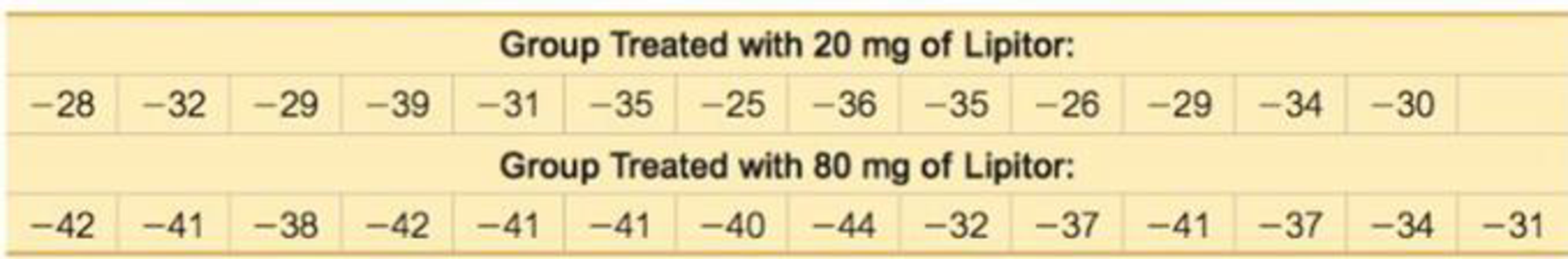
7. Clinical Trials of Lipitor The sample data below are changes in LDL cholesterol levels in clinical trials of Lipitor (atorvastatin). It was claimed that Lipitor had an effect on LDL cholesterol. (The data are based on results given in a Parke-Davis memo from David G. Orloff, M.D., the medical team leader for clinical trials of Lipitor. Pfizer declined to provide the author with the original data values.) Negative values represent decreases in LDL cholesterol. Use a 0.05 significance level to test the claim that for those treated with 20 mg of Lipitor and those treated with 80 mg of Lipitor, changes in LDL cholesterol have the same
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
use the Wilcoxon rank-sum test.
Clinical Trials of Lipitor The sample data below are changes in LDL cholesterol levels in clinical trials of Lipitor (atorvastatin). It was claimed that Lipitor had an effect on LDL cholesterol. (The data are based on results given in a Parke-Davis memo from David G. Orloff, M.D., the medical team leader for clinical trials of Lipitor. Pfizer declined to provide die author with the original data values.) Negative values represent decreases in LDL cholesterol. Use a 0.05 significance level to test the claim that for those treated with 20 mg of atorvastatin and those treated widi 80 mg of atorvastatin, changes in LDL cholesterol have the same median. What do the results suggest?
Group treated with 20 mg of atorvastatin:
−28 −32 −29 −39 −31 −35 −25 −36 −35 −26 −29 −34 −30
Group treated with 80 mg of atorvastatin:
−42 −41 −38 −42 −41 −41 −40 −44 −32 −37 −41 −37 −34 −31
can someone use minitab for this question
Are you an impulse shopper? A survey of 1000 grocery shoppers indicated that 24% of males and 57%of females make an impulse purchase every time they shop. Assume that the survey consisted of
500 males and 500 females. Complete parts (a) and (b) below.
Question content area bottom
Part 1
a. At the 0.01 level of significance, is there evidence of a difference in the proportion of males and females who make an impulse purchase every time they shop?
Let group 1 be the males, and let group 2 be the females. State the null and alternative hypotheses. Choose the correct answer below.
A. H0:π1≥π2 H1:π1<π2
BH0:π1≠π2 H1:π1=π2
C.H0:π1≤π2 H1:π1>π2
D.H0:π1=π2 H1:π1≠π2
E.H0:π1<π2 H1:π1≥π2
F.H0:π1>π2 H1:π1≤π2
Part 2
Calculate the test statistic.
χ2STAT=
(Round to three decimal places as needed.)
Part 3
Determine the critical value.
The critical value is -
(Round to three decimal places as needed.)
Part 4
State…
Chapter 13 Solutions
MyLab Statistics with Pearson eText -- Standalone Access Card -- for Elementary Statistics
Ch. 13.2 - Prob. 1BSCCh. 13.2 - Prob. 2BSCCh. 13.2 - Contradicting H1 An important step in conducting...Ch. 13.2 - Efficiency of the Sign Test Refer to Table 13-2 on...Ch. 13.2 - Matched Pairs. In Exercises 58, use the sign test...Ch. 13.2 - Matched Pairs. In Exercises 58, use the sign test...Ch. 13.2 - Matched Pairs. In Exercises 58, use the sign test...Ch. 13.2 - Matched Pairs. In Exercises 58, use the sign test...Ch. 13.2 - Nominal Data. In Exercises 912, use the sign test...Ch. 13.2 - Nominal Data. In Exercises 912, use the sign test...
Ch. 13.2 - Nominal Data. In Exercises 912, use the sign test...Ch. 13.2 - Nominal Data. In Exercises 912, use the sign test...Ch. 13.2 - Appendix B Data Sets. In Exercises 1316, refer to...Ch. 13.2 - Appendix B Data Sets. In Exercises 1316, refer to...Ch. 13.2 - Appendix B Data Sets. In Exercises 1316, refer to...Ch. 13.2 - Appendix B Data Sets. In Exercises 1316, refer to...Ch. 13.2 - Procedures for Handling Ties In the sign lest...Ch. 13.2 - Finding Critical Values Table A-7 lists critical...Ch. 13.3 - Wilcoxon Signed-Ranks Test for Body Temperatures...Ch. 13.3 - Prob. 2BSCCh. 13.3 - Prob. 3BSCCh. 13.3 - Prob. 4BSCCh. 13.3 - Using the Wilcoxon Signed-Ranks Test. In Exercises...Ch. 13.3 - Using the Wilcoxon Signed-Ranks Test. In Exercises...Ch. 13.3 - Using the Wilcoxon Signed-Ranks Test. In Exercises...Ch. 13.3 - Using the Wilcoxon Signed-Ranks Test. In Exercises...Ch. 13.3 - Prob. 9BSCCh. 13.3 - Prob. 10BSCCh. 13.3 - Prob. 11BSCCh. 13.3 - Prob. 12BSCCh. 13.3 - Rank Sums Exercise 12 uses Data Set 23 Old...Ch. 13.4 - Prob. 1BSCCh. 13.4 - Rank Sum After ranking the combined list of...Ch. 13.4 - Prob. 3BSCCh. 13.4 - Prob. 4BSCCh. 13.4 - Wilcoxon Rank-Sum Test. In Exercises 58, use the...Ch. 13.4 - Wilcoxon Rank-Sum Test. In Exercises 58, use the...Ch. 13.4 - Wilcoxon Rank-Sum Test. In Exercises 58, use the...Ch. 13.4 - Wilcoxon Rank-Sum Test. In Exercises 58, use the...Ch. 13.4 - Prob. 9BSCCh. 13.4 - Appendix B Data Sets. In Exercises 912, refer to...Ch. 13.4 - Appendix B Data Sets. In Exercises 912, refer to...Ch. 13.4 - Appendix B Data Sets. In Exercises 912, refer to...Ch. 13.4 - Prob. 13BBCh. 13.4 - Finding Critical Values Assume that we have two...Ch. 13.5 - Prob. 1BSCCh. 13.5 - Requirements Assume that we want to use the data...Ch. 13.5 - Notation For the data given in Exercise 1,...Ch. 13.5 - Efficiency Refer to Table 13-2 on page 600 and...Ch. 13.5 - Prob. 5BSCCh. 13.5 - Prob. 6BSCCh. 13.5 - Prob. 7BSCCh. 13.5 - Prob. 8BSCCh. 13.5 - Appendix B Data Sets. In Exercises 912, use the...Ch. 13.5 - Prob. 10BSCCh. 13.5 - Appendix B Data Sets. In Exercises 912, use the...Ch. 13.5 - Appendix B Data Sets. In Exercises 912, use the...Ch. 13.5 - Correcting the H Test Statistic for Ties In using...Ch. 13.6 - Regression If the methods of this section are used...Ch. 13.6 - Level of Measurement Which of the levels of...Ch. 13.6 - Notation What do r, rs , and ps denote? Why is the...Ch. 13.6 - Prob. 4BSCCh. 13.6 - In Exercises 5 and 6, use the scatterplot to find...Ch. 13.6 - In Exercises 5 and 6, use the scatterplot to find...Ch. 13.6 - Testing for Rank Correlation. In Exercises 712,...Ch. 13.6 - Prob. 8BSCCh. 13.6 - Testing for Rank Correlation. In Exercises 712,...Ch. 13.6 - Testing for Rank Correlation. In Exercises 712,...Ch. 13.6 - Prob. 11BSCCh. 13.6 - Testing for Rank Correlation. In Exercises 712,...Ch. 13.6 - Prob. 13BSCCh. 13.6 - Appendix B Data Sets. In Exercises 1316, use the...Ch. 13.6 - Appendix B Data Sets. In Exercises 1316, use the...Ch. 13.6 - Prob. 16BSCCh. 13.6 - Prob. 17BBCh. 13.7 - In Exercises 14, use the following sequence of...Ch. 13.7 - Prob. 2BSCCh. 13.7 - Prob. 3BSCCh. 13.7 - Prob. 4BSCCh. 13.7 - Using the Runs Test for Randomness. In Exercises...Ch. 13.7 - Prob. 6BSCCh. 13.7 - Prob. 7BSCCh. 13.7 - Using the Runs Test for Randomness. In Exercises...Ch. 13.7 - Prob. 9BSCCh. 13.7 - Prob. 10BSCCh. 13.7 - Runs Test with Large Samples. In Exercises 912,...Ch. 13.7 - Prob. 12BSCCh. 13 - Prob. 1CQQCh. 13 - Prob. 2CQQCh. 13 - Prob. 3CQQCh. 13 - Prob. 4CQQCh. 13 - Prob. 5CQQCh. 13 - Prob. 6CQQCh. 13 - Prob. 7CQQCh. 13 - Prob. 8CQQCh. 13 - Prob. 9CQQCh. 13 - Which Test? Three different judges give the same...Ch. 13 - Prob. 1RECh. 13 - Using Nonparametric Tests. In Exercises 110, use a...Ch. 13 - Prob. 3RECh. 13 - Prob. 4RECh. 13 - Prob. 5RECh. 13 - Prob. 6RECh. 13 - Using Nonparametric Tests. In Exercises 110, use a...Ch. 13 - Prob. 8RECh. 13 - Using Nonparametric Tests. In Exercises 1-10, use...Ch. 13 - Prob. 10RECh. 13 - Prob. 1CRECh. 13 - Prob. 2CRECh. 13 - In Exercises 13, use the data listed below. The...Ch. 13 - Prob. 4CRECh. 13 - Prob. 5CRECh. 13 - Prob. 6CRECh. 13 - Prob. 7CRECh. 13 - Prob. 8CRECh. 13 - Fear of Heights Among readers of a USA Today...Ch. 13 - Cell Phones and Crashes: Analyzing Newspaper...Ch. 13 - Prob. 1TPCh. 13 - Prob. 1FDD
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, statistics and related others by exploring similar questions and additional content below.Similar questions
- Rental revenue (in millions of dollars) 4. Can movie rental revenue be predicted? A movie studio wishes to determine the relationship between the revenue from rental of comedies on streaming services and the revenue generated from the theatrical release of such movies. The studio has the following bivariate data from a sample of fifteen comedies released over the past five years. These data give the revenue x from theatrical release (in millions of dollars) and the revenue y from streaming service rentals (in millions of dollars) for each of the fifteen movies. Also shown are the scatter plot and the least- squares regression line for the data. The equation for this line is y=3.30 +0.15x. Theater revenue, X Rental revenue, y (in millions of dollars) (in millions of dollars) 61.1 16.5 44.8 6.1 个y 18- 36.8 12.1 16 61.4 9.6 14- 13.3 9.7 12- 25.8 9.0 10- ャ★ 20.2 4.6 X. 8 26.3 7.7 7.3 2.6 48.5 15.9 27.7 11.7 20 30 70 28.3 2.8 Theater revenue 66.3 10.1 (in millions of dollars) 29.9 6.3 22arrow_forwardYou perform an ANOVA to test that there are no main effects of factor A, no main effects of factor B, and no interaction between factors A and B. Some of the results are presented in the following ANOVA table. Source Between treatments Factor A Factor B A X B interaction Within treatments Total ANOVA Table SS 6.5667 0.0667 12.1667 df 5 29 MS 4.0333 1.2334 F 17.29 5.29 Work through the following steps to complete the preceding ANOVA table. 1. The main effect for factor A evaluates the mean differences between the levels of factor A. The main effect for factor B evaluates the mean differences between the levels of factor B. Select the correct values for the sums of squares for factors A and B in the ANOVA table. 2. Select the correct value for the within-treatments sum of squares in the ANOVA table. 3. Select the correct degrees of freedom for all the sums of squares in the ANOVA table. 4. Select the correct values for the mean square due to A X B interaction, the within treatments mean…arrow_forwardChest Injury Rating Car Driver Passenger Car Driver Passenger 1 42 35 10 36 37 2 42 35 11 36 37 3 34 45 12 43 58 4 34 45 13 40 42 5 45 45 14 43 58 6 40 42 15 37 41 7 42 46 16 37 41 8 43 58 17 44 57 9 45 43 18 42 42 Generate test results (α = .01) using data analysis (EXCEL).arrow_forward
- 9arrow_forwardData in the following example are selected from a larger experiment on the use of drugs in the treatment of leprosy (Snedecor and Cochran 1967). Variables in the study are as follows: Drug two antibiotics (A and D) and a control (F) Pre-Treatment (X) a pretreatment score of leprosy bacilli Post-Treatment (Y) a posttreatment score of leprosy bacilli Ten patients are selected for each drug treatment, and six sites on each patient are measured for leprosy bacilli. The covariate (a pretreatment score) is included in the model for increased precision in determining the effect of drug treatments on the posttreatment count of bacilli. An Control Earrow_forwardplease show diagram of p valuearrow_forward
- Treating Chronic Fatigue Syndrome: Patients with chronic fatigue syndrome were tested, and then retested after being treated with fludrocortisones. Listed below are the changes in fatigue after the treatment (based on data from “The Relationship Between Neurally Mediated Hypotension and the Chronic Fatigue Syndrome” by Bou-Holaigah, Rowe, Kan, and Calkins, Journal of the American Medical Association, 274, No. 12). A standard scale from -7 to +7 was used, with positive values representing improvements. Use a 0.05 significance level to test the claim that the mean change is not zero. Does the treatment appear to be effective? 6 5 0 5 6 7 3 3 2 6 5 5 0 6 3 4 3 7 0 4 4 Hypotheses: __________________________________________________________________________________ P-value: ____________________ Conclusion on null hypothesis: Conclusion on claim and answer to the question:arrow_forwarded weight (in kg) and total serum cholesterol (TSC in mg/dl) data for five men (n = 5). They tested for association between weight and TSC at 5% level of significance. weight, X TSC, Y X*X Y*Y X*Y 37 2.9 1369 8.41 107.3 50 0.7 2500 0.49 35.0 67 3.3 4489 10.89 221.1 70 4.2 4900 17.64 294.0 57 3.8 3249 14.44 216.6 SUM: 281 14.9 16507 51.87 874.0 What are the Null and Alternate hypotheses for this test? Clearly label your answers as HỌ or H1. (Do not use a numerical format. Both of your statements must be in sentence form, and say something about the weightarrow_forwardLemons and Car Crashes Listed below are annual data for various years. The data are weights (metric tons) of lemons imported from Mexico and U.S. car crash fatality rates per 100,000 population [based on data from “The Trouble with QSAR (or How I Learned to Stop Worrying and Embrace Fallacy)” by Stephen Johnson, Journal of Chemical Information and Modeling, Vol. 48, No. 1]. Lemon Imports 230 265 358 480 530 Crash Fatality Rate 15.9 15.7 15.4 15.3 14.9 Question ---- > Find the critical values of r using α = 0.05 and use test statistic r = -0.959 to determine whether there is sufficient evidence to support a claim of a linear correlation between the two variables. Group of answer choices r = ± 0.811, There is enough evidence to Support the Claim r = ± 0.878, There NOT is enough evidence to Support the Claim r = ± 0.811, There NOT is enough evidence to Support the Claim r = ± 0.878, There is enough evidence to Support the Claimarrow_forward
- Lemons and Car Crashes Listed below are annual data for various years. The data are weights (metric tons) of lemons imported from Mexico and U.S. car crash fatality rates per 100,000 population [based on data from “The Trouble with QSAR (or How I Learned to Stop Worrying and Embrace Fallacy)” by Stephen Johnson, Journal of Chemical Information and Modeling, Vol. 48, No. 1]. Lemon Imports 230 265 358 480 530 Crash Fatality Rate 15.9 15.7 15.4 15.3 14.9 Question ---- > Find the critical values of r using α = 0.05 and write Ho and H1 Group of answer choices r = ± 0.878 Ho : ρ = 0 H1: ρ < 0 r = ± 0.811 Ho : ρ = 0 H1: ρ > 0 r = ± 0.878 Ho : ρ = 0 H1: ρ ≠ 0 r = 0.878 Ho : ρ > 0 H1: ρ ≠ 0arrow_forwardUsing the data below: How do we create a bivariate analysis?arrow_forwardThe data show the population (in thousands) for a recent year of a sample of aities in South Carolina. 24 19 19 18 62 18 21 24 20 11 22 33 93 13 21 29 13 12 108 45 20 46 108 39 30 51 Send data to Excel Part 1 of 8 The data value 21 corresponds to the 41" percentile. Part: 1/8 Part 2 of 8 The data value corresponds to the 71" percentile.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
Hypothesis Testing using Confidence Interval Approach; Author: BUM2413 Applied Statistics UMP;https://www.youtube.com/watch?v=Hq1l3e9pLyY;License: Standard YouTube License, CC-BY
Hypothesis Testing - Difference of Two Means - Student's -Distribution & Normal Distribution; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=UcZwyzwWU7o;License: Standard Youtube License