Thinking Like an Engineer: An Active Learning Approach (3rd Edition)
3rd Edition
ISBN: 9780133593211
Author: Elizabeth A. Stephan, David R. Bowman, William J. Park, Benjamin L. Sill, Matthew W. Ohland
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 13, Problem 12RQ
When a fluid flows around an object, it creates a force, called the drag force, that pulls on the object. The coefficient of drag (Cd) is a dimensionless number that describes the relationship between the force created and the fluid and object properties, given as
Where FD is drag force, ρ is the fluid density, and υ is the velocity of the object relative to the fluid. The area of the object the force acts upon is AP, and for spheres is given by the area of a circle. The Reynolds number in this situation is written as
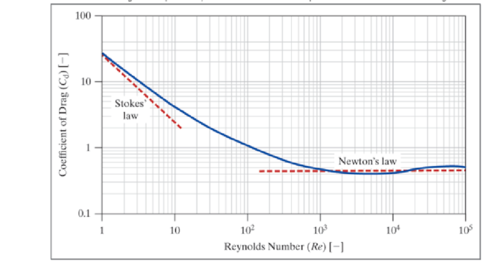
where DP is the diameter of the object the force acts upon. The following chart shows this relationship. The dashed lines show the predicted theories of Stokes and Newton compared to the solid line of actual results.
- a. If the Reynolds number is 500, what is the coefficient of drag?
- b. If the coefficient of drag is 2, what is the Reynolds number?
Ethylene glycol has a dynamic viscosity of 9.13 centipoise and a specific gravity of 1.109.
- c. If the fluid flows around a sphere of diameter 1 centimeter travelling at a velocity of 2.15 centimeters per second, determine the drag force on the particle in units of newtons. (Hint: First determine the Reynolds number.)
- d. If a coefficient of drag of 10 is produced, what is the diameter of the particle? Assume the fluid moving at 1 centimeter per second (Hint: First determine the Reynolds number.)
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
using the theorem of three moments, find all the reactions and supports
(An ellipsoidal trapping region for the Lorenz equations) Show that there is a certain ellipsoidal region E of the form rx2 + σy2 + σ(z − 2r)2 ≤ C such that all trajectories of the Lorenz equations eventually enter E and stay in there forever. For a much stiffer challenge, try to obtain the smallest possible value of C with this property.
A) In a factory, an s-type pitot tube was used to calculate the velocity of dry air for a point
inside a stack.
Calculate the velocity at that point (ft/sec) using following conditions:
●
•
•
Pressure = 30.23 ± 0.01 in Hg (ambient)
Pitot tube coefficient = 0.847 ± 0.03
Temperature = 122 ± 0.1 F (stack)
Temperature = 71.2 ± 0.1 F (ambient)
AP = 0.324 ± 0.008 in H2O (pitot tube)
•
AP = 0.891 ± 0.002 in H2O (stack)
B) Find the dominant error(s) when determining precision for the problem.
C) For part A, what is the precision in ft/sec for the velocity?
Chapter 13 Solutions
Thinking Like an Engineer: An Active Learning Approach (3rd Edition)
Ch. 13.2 - An unknown amount of oxygen, kept in 8 piston-type...Ch. 13.2 - The data shown graphically in the figure describe...Ch. 13.4 - Prob. 3CCCh. 13.4 - Prob. 4CCCh. 13 - Prob. 1ICACh. 13 - Prob. 2ICACh. 13 - Prob. 3ICACh. 13 - Prob. 4ICACh. 13 - Prob. 5ICACh. 13 - Referring to the previous ICA 13-5, Angus is also...
Ch. 13 - Prob. 7ICACh. 13 - The following instructions apply to ICA 13-7 to...Ch. 13 - The following instructions apply to ICA 13-7 to...Ch. 13 - Prob. 10ICACh. 13 - Prob. 11ICACh. 13 - Prob. 12ICACh. 13 - The following instructions apply to ICA 13-10 to...Ch. 13 - Prob. 14ICACh. 13 - Prob. 15ICACh. 13 - Prob. 16ICACh. 13 - Prob. 17ICACh. 13 - Prob. 18ICACh. 13 - Prob. 19ICACh. 13 - Prob. 20ICACh. 13 - Prob. 21ICACh. 13 - As a reminder, the Reynolds number is discussed in...Ch. 13 - As a reminder, the Reynolds number is discussed in...Ch. 13 - Prob. 1RQCh. 13 - Prob. 2RQCh. 13 - 3. An environmental engineer has obtained a...Ch. 13 - 4. A growing field of inquiry that poses both...Ch. 13 - If an object is heated, the temperature of the...Ch. 13 - The Volcanic Explosivity Index (VEI) is based...Ch. 13 - You are an engineer for a plastics manufacturing...Ch. 13 - A Pitot tube is a device used to measure the...Ch. 13 - As part of an electronic music synthesizer you...Ch. 13 - The following data were collected during testing...Ch. 13 - The relationship of the power required by a...Ch. 13 - When a fluid flows around an object, it creates a...
Additional Engineering Textbook Solutions
Find more solutions based on key concepts
Write a statement that defines a file stream object named places. The object will be used for both output and i...
Starting Out with C++: Early Objects (9th Edition)
Automobile Costs Write a program that asks the user to enter the monthly costs for the following expenses incur...
Starting Out with Python (4th Edition)
Describe two properties that each candidate key must satisfy.
Modern Database Management
What are some of the attractive features of resistance welding when viewed from a manufacturing standpoint?
Degarmo's Materials And Processes In Manufacturing
A file that contains a Flash animation uses the __________ file extension. a. .class b. .swf c. .mp3 d. .flash
Web Development and Design Foundations with HTML5 (8th Edition)
What is the importance of modeling in engineering? How are the mathematical models for engineering processes pr...
HEAT+MASS TRANSFER:FUND.+APPL.
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- Q1/ For what value of x do the power series converge: 8 (-1)n-1. x2n-1 2n-1 x3 x5 = X n=1 3 Q2/ Find the Interval of convergence and Radius of convergence of the series: 8 n Σ 3+1 n=1 (x)"arrow_forwardExample-1: l D A uniform rotor of length 0.6 m and diameter 0.4 m is made of steel (density 7810 kg/m³) is supported by identical short bearings of stiffness 1 MN/m in the horizontal and vertical directions. If the distance between the bearings is 0.7 m, determine the natural frequencies and plot whirl speed map. Solution: Barrow_forwardfind the laplace transform for the flowing function 2(1-e) Ans. F(s)=- S 12) k 0 Ans. F(s)= k s(1+e) 0 a 2a 3a 4a 13) 2+ Ans. F(s)= 1 s(1+e") 3 14) f(t)=1, 0arrow_forwardFind the solution of the following Differential Equations Using Laplace Transforms 1) 4y+2y=0. y(0)=2. y'(0)=0. 2) y+w²y=0, (0)=A, y'(0)=B. 3) +2y-8y 0. y(0)=1. y'(0)-8. 4)-2-3y=0, y(0)=1. y'(0)=7. 5) y-ky'=0, y(0)=2, y'(0)=k. 6) y+ky'-2k²y=0, y(0)=2, y'(0) = 2k. 7) '+4y=0, y(0)=2.8 8) y+y=17 sin(21), y(0)=-1. 9) y-y-6y=0, y(0)=6, y'(0)=13. 10) y=0. y(0)=4, y' (0)=0. 11) -4y+4y-0, y(0)=2.1. y'(0)=3.9 12) y+2y'+2y=0, y(0)=1, y'(0)=-3. 13) +7y+12y=21e". y(0)=3.5. y'(0)=-10. 14) "+9y=10e". y(0)=0, y'(0)=0. 15) +3y+2.25y=91' +64. y(0)=1. y'(0) = 31.5 16) -6y+5y-29 cos(2t). y(0)=3.2, y'(0)=6.2 17) y+2y+2y=0, y(0)=0. y'(0)=1. 18) y+2y+17y=0, y(0)=0. y'(0)=12. 19) y"-4y+5y=0, y(0)=1, y'(0)=2. 20) 9y-6y+y=0, (0)-3, y'(0)=1. 21) -2y+10y=0, y(0)=3, y'(0)=3. 22) 4y-4y+37y=0, y(0)=3. y'(0)=1.5 23) 4y-8y+5y=0, y(0)=0, y'(0)=1. 24) ++1.25y-0, y(0)=1, y'(0)=-0.5 25) y 2 cos(r). y(0)=2. y'(0) = 0. 26) -4y+3y-0, y(0)=3, y(0) 7. 27) y+2y+y=e y(0)=0. y'(0)=0. 28) y+2y-3y=10sinh(27), y(0)=0. y'(0)=4. 29)…arrow_forwardAuto Controls A union feedback control system has the following open loop transfer function where k>0 is a variable proportional gain i. for K = 1 , derive the exact magnitude and phase expressions of G(jw). ii) for K = 1 , identify the gaincross-over frequency (Wgc) [where IG(jo))| 1] and phase cross-overfrequency [where <G(jw) = - 180]. You can use MATLAB command "margin" to obtain there quantities. iii) Calculate gain margin (in dB) and phase margin (in degrees) ·State whether the closed-loop is stable for K = 1 and briefly justify your answer based on the margin . (Gain marginPhase margin) iv. what happens to the gain margin and Phase margin when you increase the value of K?you You can use for loop in MATLAB to check that.Helpful matlab commands : if, bode, margin, rlocus NO COPIED SOLUTIONSarrow_forwardThe 120 kg wheel has a radius of gyration of 0.7 m. A force P with a magnitude of 50 N is applied at the edge of the wheel as seen in the diagram. The coefficient of static friction is 0.3, and the coefficient of kinetic friction is 0.25. Find the acceleration and angular acceleration of the wheel.arrow_forwardAuto Controls Using MATLAB , find the magnitude and phase plot of the compensators NO COPIED SOLUTIONSarrow_forward4-81 The corner shown in Figure P4-81 is initially uniform at 300°C and then suddenly exposed to a convection environment at 50°C with h 60 W/m². °C. Assume the = 2 solid has the properties of fireclay brick. Examine nodes 1, 2, 3, 4, and 5 and deter- mine the maximum time increment which may be used for a transient numerical calculation. Figure P4-81 1 2 3 4 1 cm 5 6 1 cm 2 cm h, T + 2 cmarrow_forwardAuto Controls A union feedback control system has the following open loop transfer function where k>0 is a variable proportional gain i. for K = 1 , derive the exact magnitude and phase expressions of G(jw). ii) for K = 1 , identify the gaincross-over frequency (Wgc) [where IG(jo))| 1] and phase cross-overfrequency [where <G(jw) = - 180]. You can use MATLAB command "margin" to obtain there quantities. iii) Calculate gain margin (in dB) and phase margin (in degrees) ·State whether the closed-loop is stable for K = 1 and briefly justify your answer based on the margin . (Gain marginPhase margin) iv. what happens to the gain margin and Phase margin when you increase the value of K?you You can use for loop in MATLAB to check that.Helpful matlab commands : if, bode, margin, rlocus NO COPIED SOLUTIONSarrow_forwardAuto Controls Hand sketch the root Focus of the following transfer function How many asymptotes are there ?what are the angles of the asymptotes?Does the system remain stable for all values of K NO COPIED SOLUTIONSarrow_forward-400" 150" in Datum 80" 90" -280"arrow_forwardUsing hand drawing both of themarrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_ios
Recommended textbooks for you
Principles of Heat Transfer (Activate Learning wi...Mechanical EngineeringISBN:9781305387102Author:Kreith, Frank; Manglik, Raj M.Publisher:Cengage Learning
Principles of Heat Transfer (Activate Learning wi...
Mechanical Engineering
ISBN:9781305387102
Author:Kreith, Frank; Manglik, Raj M.
Publisher:Cengage Learning
Unit Conversion the Easy Way (Dimensional Analysis); Author: ketzbook;https://www.youtube.com/watch?v=HRe1mire4Gc;License: Standard YouTube License, CC-BY