
Concept explainers
Assuming that the earth is a perfectly round, smooth ball of radius 4000 miles and that 1 mile = 5280 feet, how far away does the horizon appear to be to a 5-foot-tall person on a clear day? Explain your reasoning. To solve this problem, start by making a math drawing that shows the cross-section of the earth, a person standing on the surface of the earth, and the straight line of the person's gaze reaching to the horizon. (Obviously, you won’t want to draw this to scale.) You will need to use the following geometric fact: If a line is tangent to a circle at a point P (meaning itjust “grazes” the circle at the point P; it meets the circle only at that one point), then that line is perpendicular to the line connecting P and the center of the circle, as illustrated in Figure 12.105
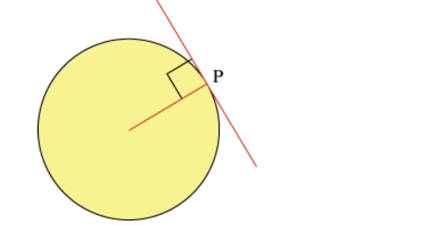
Figure 12.105 A line tangent to a circle.
Want to see the full answer?
Check out a sample textbook solution
Chapter 12 Solutions
Mathematics for Elementary Teachers with Activities (5th Edition)
Additional Math Textbook Solutions
Pathways To Math Literacy (looseleaf)
Finite Mathematics for Business, Economics, Life Sciences and Social Sciences
Elementary Statistics
Precalculus: A Unit Circle Approach (3rd Edition)
Excursions in Modern Mathematics (9th Edition)
Finite Mathematics & Its Applications (12th Edition)
- 4 3 2 -5 4-3 -2 -1 1 2 3 4 5 12 23 -4 The function graphed above is: Increasing on the interval(s) Decreasing on the interval(s)arrow_forwardQuestion 4 The plot below represents the function f(x) 8 7 3 pts O -4-3-2-1 6 5 4 3 2 + 1 2 3 5 -2+ Evaluate f(3) f(3) = Solve f(x) = 3 x= Question 5arrow_forwardQuestion 14 6+ 5 4 3 2 -8-2 2 3 4 5 6 + 2 3 4 -5 -6 The graph above is a transformation of the function f(x) = |x| Write an equation for the function graphed above g(x) =arrow_forward
- Question 8 Use the graph of f to evaluate the following: 6 f(x) 5 4 3 2 1 -1 1 2 3 4 5 -1 t The average rate of change of f from 4 to 5 = Question 9 10 ☑ 4parrow_forwardQuestion 15 ✓ 6 pts 1 Details The function shown below is f(x). We are interested in the transformed function g(x) = 3f(2x) - 1 a) Describe all the transformations g(x) has made to f(x) (shifts, stretches, etc). b) NEATLY sketch the transformed function g(x) and upload your graph as a PDF document below. You may use graph paper if you want. Be sure to label your vertical and horizontal scales so that I can tell how big your function is. 1- 0 2 3 4 -1- Choose File No file chosen Question 16 0 pts 1 Detailsarrow_forwardAND B A Ꭰarrow_forward
- ANBNC ND B こ Ꭰarrow_forward1 Matching 10 points Factor and Solve 1)x3-216 0, x = {6,[B]} 2) 16x3 = 54 x-[3/2,[D]] 3)x4x2-42 0 x= [ +/-isqrt(7), [F] } 4)x+3-13-9x x=[+/-1.[H]] 5)x38x2+16x=0, x = {0,[K}} 6) 2x6-10x-48x2-0 x-[0, [M], +/-isqrt(3)) 7) 3x+2x²-8 x = {+/-i sqrt(2), {Q}} 8) 5x³-3x²+32x=2x+18 x = {3/5, [S]} [B] [D] [F] [H] [K] [M] [Q] +/-2 sqrt(2) +/- i sqrt(6) (-3+/-3 i sqrt(3))/4 +/- 1 +/-sqrt(6) +/- 2/3 sqrt(3) 4 -3 +/- 3 i sqrt(3) [S]arrow_forwardD U(AUBUC) B Darrow_forward
- helparrow_forwardAnswer question 2.28 please.arrow_forwardQuestion 2 Let F be a solenoidal vector field, suppose V × F = (-8xy + 12z², −9x² + 4y² + 9z², 6y²), and let (P,Q,R) = V²F(.725, —.283, 1.73). Then the value of sin(2P) + sin(3Q) + sin(4R) is -2.024 1.391 0.186 -0.994 -2.053 -0.647 -0.588 -1.851 1 ptsarrow_forward
 Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGALAlgebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGALAlgebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning, Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning





