Chapter 12.5, Problem 52ES

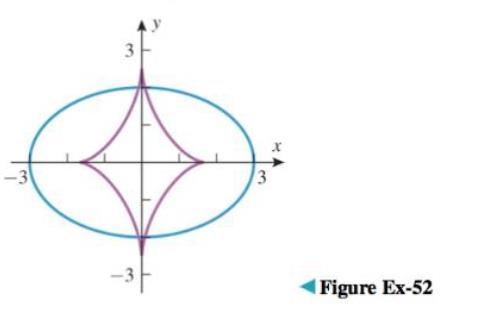
The evolute of a smooth parametric curve C in 2-space is the curve formed from the centers of curvature of C. The accompanying figure shows the ellipse $x=3\text{cos}t,y=2\text{sin}t(0\le t\le 2\pi )$ and its evolute graphed together.

(a) Which points on the evolute correspond to $t=0\text{ and }t=\pi /2?$

(b) In what direction is the evolute traced as t increases from $0\text{ to }2\pi ?$

(c) What does the evolute of a circle look like? Explain your reasoning.