MECHANICS OF MATERIALS
11th Edition
ISBN: 9780137605521
Author: HIBBELER
Publisher: RENT PEARS
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 12, Problem 9RP
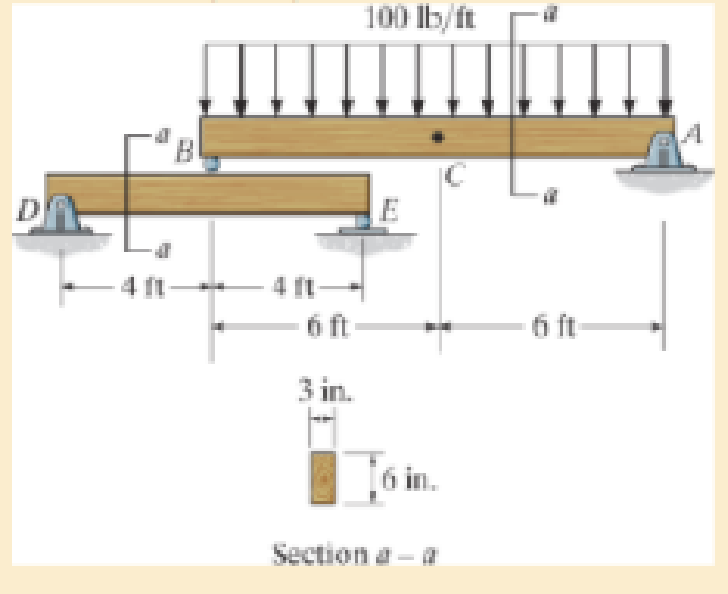
Using the method of superposition, determine the deflection at C of beam AB. The beams are made of wood having a modulus of elasticity of E = 1.5(103) ksi.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
1- Determine the following: 1- RSHF? 2- C.C.C in tons-ref. 3- Mass
of supply air?
Fresh
Spray chilled
water
S
air
100% RH
To 34 C db & 26 wbt
S
Operation
fan
room I
Exhaust
air
Ti 22 C db & 50% RH
How do I solve this task
A weight for a lift is suspended using an adapter. The counterweight is held up with 4 screws. The weight F is 3200kg.
The screws have a strength class of 8.8. Safety factor 3
Which is the smallest bunch size that can be used?+_Sr/Fm =0,16Gr=0,71ơ=800·0.8=640 MPaAs=?Fmax= As·ơ·GrFs=ơs·AsFFm= Fs· GFSF =SF / FFm · FFm Fpreload =Fload / SF → Fload /3Fpreload per screw =Fload / SF → Fload /4As=Fpreload per screw /ơ·Gr → As= Fpreload per screw / 640· 0.71
The correct answer should be M12 with As=84.3mm²
...
TELEGRAM
ديسمبر
۲۰۲ عند الساعة
سوأل الوجه البينة
۲۷
- Find the equivalent resistance between
A and B
bellows
For the circuit shown.
• All resistances in Ohms.
2
C
2
A
4
B
www
4
E
5
www
ww
8
bar K.
Dr. Abduljabbo
Hammade
27/12/2024
Chapter 12 Solutions
MECHANICS OF MATERIALS
Ch. 12.2 - Determine the slope and deflection of end A of the...Ch. 12.2 - Determine the slope and deflection of end A of the...Ch. 12.2 - Determine the slope of end A of the cantilevered...Ch. 12.2 - Determine the maximum deflection of the simply...Ch. 12.2 - Determine the maximum deflection of the simply...Ch. 12.2 - Determine the slope of the simply supported beam...Ch. 12.2 - An L2 steel strap having a thickness of 0.125 in....Ch. 12.2 - The L2 steel blade of the band saw wraps around...Ch. 12.2 - A picture is taken of a man performing a pole...Ch. 12.2 - A torque wrench is used to tighten the nut on a...
Ch. 12.2 - The pipe can be assumed roller supported at its...Ch. 12.2 - Determine the equations of the elastic curve for...Ch. 12.2 - Determine the equations of the elastic curve using...Ch. 12.2 - Determine the maximum deflection of the solid...Ch. 12.2 - Determine the equation of the elastic curve using...Ch. 12.2 - Determine the equations of the elastic curve using...Ch. 12.3 - The shaft supports the two pulley loads shown....Ch. 12.3 - Determine the equation of the elastic curve, the...Ch. 12.3 - Determine the equation of the elastic curve and...Ch. 12.3 - Determine the maximum deflection of the...Ch. 12.3 - Prob. 45PCh. 12.3 - Prob. 46PCh. 12.3 - Prob. 47PCh. 12.3 - Prob. 48PCh. 12.4 - Determine the slope and deflection of end A of the...Ch. 12.4 - Determine the slope and deflection of end A of the...Ch. 12.4 - Determine the slope and deflection of end A of the...Ch. 12.4 - Determine the slope and deflection at A of the...Ch. 12.4 - Prob. 11FPCh. 12.4 - Determine the maximum deflection of the simply...Ch. 12.4 - Determine the slope and deflection at C. El is...Ch. 12.4 - Prob. 54PCh. 12.4 - The composite simply supported steel shaft is...Ch. 12.4 - Determine the maximum deflection of the...Ch. 12.4 - Prob. 60PCh. 12.4 - Determine the slope at A and the maximum...Ch. 12.4 - Determine the displacement of the 20-mm-diameter...Ch. 12.4 - The two force components act on the tire of the...Ch. 12.4 - Determine the slope at B and deflection at C. El...Ch. 12.4 - Prob. 79PCh. 12.5 - The W10 15 cantilevered beam is made of A-36...Ch. 12.5 - The W14 43 simply supported beam is made of A992...Ch. 12.5 - The W14 43 simply supported beam is made of A992...Ch. 12.5 - The W14 43 simply supported beam is made of A-36...Ch. 12.7 - Determine the reactions at the supports A and B,...Ch. 12.7 - Determine the reactions at the supports A, B, and...Ch. 12.7 - Determine the reactions at the supports A and B,...Ch. 12.7 - The beam has a constant E1I1 and is supported by...Ch. 12.8 - Determine the reaction at the supports, then draw...Ch. 12.9 - Determine the reactions at the fixed support A and...Ch. 12.9 - Determine the reactions at the fixed support A and...Ch. 12.9 - Determine the reactions at the fixed support A and...Ch. 12.9 - Determine the reaction at the roller B. EI is...Ch. 12.9 - Determine the reaction at the roller B. EI is...Ch. 12.9 - Determine the reaction at the roller support B if...Ch. 12.9 - Determine the reactions at the journal bearing...Ch. 12.9 - Determine the reactions at the supports, then draw...Ch. 12.9 - Determine the reactions at the supports, then draw...Ch. 12.9 - The rim on the flywheel has a thickness t, width...Ch. 12.9 - Determine the moment developed in each corner....Ch. 12 - Determine the equation of the elastic curve. Use...Ch. 12 - Draw the bending-moment diagram for the shaft and...Ch. 12 - Determine the moment reactions at the supports A...Ch. 12 - Specify the slope at A and the maximum deflection....Ch. 12 - Determine the maximum deflection between the...Ch. 12 - Determine the slope at B and the deflection at C....Ch. 12 - Determine the reactions, then draw the shear and...Ch. 12 - El is constant.Ch. 12 - Using the method of superposition, determine the...
Additional Engineering Textbook Solutions
Find more solutions based on key concepts
17–1C A high-speed aircraft is cruising in still air. How does the temperature of air at the nose of the aircra...
Thermodynamics: An Engineering Approach
What types of coolant are used in vehicles?
Automotive Technology: Principles, Diagnosis, And Service (6th Edition) (halderman Automotive Series)
CONCEPT QUESTIONS
15.CQ3 The ball rolls without slipping on the fixed surface as shown. What is the direction ...
Vector Mechanics for Engineers: Statics and Dynamics
This optional Google account security feature sends you a message with a code that you must enter, in addition ...
SURVEY OF OPERATING SYSTEMS
A byte is made up of eight a. CPUs b. addresses c. variables d. bits
Starting Out with Java: From Control Structures through Objects (7th Edition) (What's New in Computer Science)
The following C++ program will not compile because the lines have been mixed up. cout Success\n; cout Success...
Starting Out with C++ from Control Structures to Objects (9th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- P₂ 7+1 * P₁ ART 2 P (P₁ - P₂- Zgp) 21 / Prove that :- m² a cda A₂ == * Cde actual mip Solutionarrow_forwardQ1/ Show that (actual 02/ A simple iet == Cda Cdf х Af 2/Y - Y+1/Y 2P(P1-P2-zxgxpr)arrow_forward5. Determine the transfer function of G(s) = 01(s)/T₁(s) and 02(s)/T₁ for the mechanical system shown in Figure Q5. (Hints: assume zero initial condition) T₁(t) 01(t) 102(1) Ол N1 D1 D2 No. 1790220000 N2 Figure Q5 K2arrow_forward
- A spring package with two springs and an external force, 200N. The short spring has a loin of 35 mm. Constantly looking for spring for short spring so that total compression is 35 mm (d). Known values: Long spring: Short spring:C=3.98 N/mm Lo=65mmLo=87.4mmF=c·fTotal compression is same for both spring. 200 = (3.98(c1) × 35) + (c₂ × 35) 200 = 139.3 + 35c₂ 200 - 139.3 = 35c₂ 60.7 = 35c₂ c₂ = 60.7/35 Short spring (c₂) = 1.73 N/mm According to my study book, the correct answer is 4.82N/mm What is wrong with the calculating?arrow_forwardWhat is the reason for this composition?arrow_forwardHomework: ANOVA Table for followed design B AB Dr -1 -1 1 (15.18,12) 1 -1 -1 (45.48.51) -1 1 -1 (25,28,19) 1 1 (75.75,81)arrow_forward
- 20. [Ans. 9; 71.8 mm] A semi-elliptical laminated spring is made of 50 mm wide and 3 mm thick plates. The length between the supports is 650 mm and the width of the band is 60 mm. The spring has two full length leaves and five graduated leaves. If the spring carries a central load of 1600 N, find: 1. Maximum stress in full length and graduated leaves for an initial condition of no stress in the leaves. 2. The maximum stress if the initial stress is provided to cause equal stress when loaded. [Ans. 590 MPa ; 390 MPa ; 450 MPa ; 54 mm] 3. The deflection in parts (1) and (2).arrow_forwardQ6/ A helical square section spring is set inside another, the outer spring having a free length of 35 mm greater than the inner spring. The dimensions of each spring are as follows: Mean diameter (mm) Side of square section (mm) Active turns Outer Inner Spring Spring 120 70 8 7 20 15 Determine the (1) Maximum deflection of the two springs and (2) Equivalent spring rate of the two springs after sufficient load has been applied to deflect the outer spring 60 mm. Use G = 83 GN/m².arrow_forwardQ2/ The bumper springs of a railway carriage are to be made of rectangular section wire. The ratio of the longer side of the wire to its shorter side is 1.5, and the ratio of mean diameter of spring to the longer side of wire is nearly equal to 6. Three such springs are required to bring to rest a carriage weighing 25 kN moving with a velocity of 75 m/min with a maximum deflection of 200 mm. Determine the sides of the rectangular section of the wire and the mean diameter of coils when the shorter side is parallel to the axis of the spring. The allowable shear stress is not to exceed 300 MPa and G = 84 kN/mm². Q6/ A belicalarrow_forward
- 11. A load of 2 kN is dropped axially on a close coiled helical spring, from a height of 250 mm. The spring has 20 effective turns, and it is made of 25 mm diameter wire. The spring index is 8. Find the maximum shear stress induced in the spring and the amount of compression produced. The modulus of rigidity for the material of the spring wire is 84 kN/mm². [Ans. 287 MPa; 290 mm]arrow_forwardWhat is the reason for this composition?arrow_forwardHomework: ANOVA Table for followed design B AB Dr -1 -1 1 (15.18,12) 1 -1 -1 (45.48.51) -1 1 -1 (25,28,19) 1 1 (75.75,81)arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Elements Of Electromagnetics
Mechanical Engineering
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Oxford University Press
Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:9780134319650
Author:Russell C. Hibbeler
Publisher:PEARSON
Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:9781259822674
Author:Yunus A. Cengel Dr., Michael A. Boles
Publisher:McGraw-Hill Education
Control Systems Engineering
Mechanical Engineering
ISBN:9781118170519
Author:Norman S. Nise
Publisher:WILEY
Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning
Engineering Mechanics: Statics
Mechanical Engineering
ISBN:9781118807330
Author:James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:WILEY
Solids: Lesson 53 - Slope and Deflection of Beams Intro; Author: Jeff Hanson;https://www.youtube.com/watch?v=I7lTq68JRmY;License: Standard YouTube License, CC-BY