Bundle: Physics for Scientists and Engineers with Modern Physics, Loose-leaf Version, 9th + WebAssign Printed Access Card, Multi-Term
9th Edition
ISBN: 9781305932302
Author: Raymond A. Serway, John W. Jewett
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 12, Problem 5P
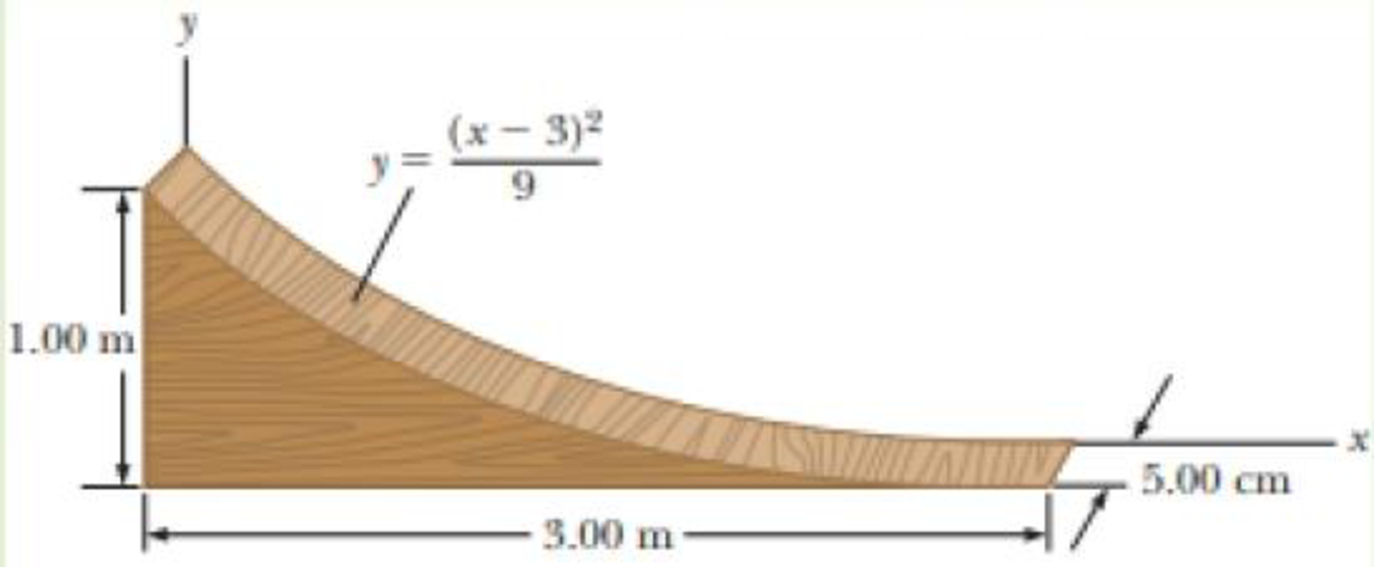
Your brother is opening a skateboard shop. He has created a sign for his shop made from a uniform material and in the shape shown in Figure P12.5. The shape of the sign represents one of the hills in the skateboard park he plans on building on land adjacent to the shop. The curve on the top of the sign is described by the function y = (x − 3)2/9. When the sign arrives in his shop, your brother wants to hang it from a single wire outside the shop. But he doesn’t know where on the sign to attach the wire so that the bottom edge of the sign will hang in a horizontal orientation. He asks for your help.
Figure P12.5
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
5
A rectangular cantilever beam AB has point A positioned at the origin of the coordinate system and
point B at coordinates (1.2, -0.3, 2.4) m. The vector r₁ = (1î + 12ĵ + 1ê) m is oriented parallel to the
thin cross-sectional dimension, and is perpendicular to to FAB (directed along the beam axis AB).
Z
P
a Verify that r₁ is perpendicular to TAB
2
TAB
X
b Determine the unit vector 2 that is perpendicular to both r₁ and rab and has the orientation
shown in the figure.
c Assuming that the force P applied to point B of the beam has 1000 N magnitude and direction
angles x 144°, 0y = 72°, and 0₂ = 60°; Determine the components of the 1000 N force, namely
PAB, P₁, and P2, in the directions AB, 7₁, and 2, respectively.
=
=
For a summer job you are working as an engineering intern for a metal fabricator. You are assigned the task of determining the center of mass an object to be made from a very thin sheet of airplane
aluminum. The rough drawing you are given to work from is as shown below, and the outside radius of the object is to be 1.36 m.
Xcm
m
Ycm =
m
R
R
2
Chapter 12 Solutions
Bundle: Physics for Scientists and Engineers with Modern Physics, Loose-leaf Version, 9th + WebAssign Printed Access Card, Multi-Term
Ch. 12.1 - Consider the object subject to the two forces of...Ch. 12.1 - Consider the object subject to the three forces in...Ch. 12.2 - A meterstick of uniform density is hung from a...Ch. 12.4 - For the three parts of this Quick Quiz, choose...Ch. 12 - Prob. 1OQCh. 12 - Prob. 2OQCh. 12 - Prob. 3OQCh. 12 - Prob. 4OQCh. 12 - In the cabin of a ship, a soda can rests in a...Ch. 12 - Prob. 6OQ
Ch. 12 - Prob. 7OQCh. 12 - Prob. 8OQCh. 12 - Prob. 9OQCh. 12 - Prob. 10OQCh. 12 - Prob. 1CQCh. 12 - Prob. 2CQCh. 12 - Prob. 3CQCh. 12 - Prob. 4CQCh. 12 - Prob. 5CQCh. 12 - Prob. 6CQCh. 12 - Prob. 7CQCh. 12 - What kind of deformation does a cube of Jell-O...Ch. 12 - Prob. 1PCh. 12 - Why is the following situation impossible? A...Ch. 12 - Prob. 3PCh. 12 - Prob. 4PCh. 12 - Your brother is opening a skateboard shop. He has...Ch. 12 - A circular pizza of radius R has a circular piece...Ch. 12 - Prob. 7PCh. 12 - Prob. 8PCh. 12 - Prob. 9PCh. 12 - Prob. 10PCh. 12 - A uniform beam of length 7.60 m and weight 4.50 ...Ch. 12 - Prob. 12PCh. 12 - Prob. 13PCh. 12 - A uniform ladder of length L and mass m1 rests...Ch. 12 - A flexible chain weighing 40.0 N hangs between two...Ch. 12 - A uniform beam of length L and mass m shown in...Ch. 12 - Figure P12.13 shows a claw hammer being used to...Ch. 12 - A 20.0-kg floodlight in a park is supported at the...Ch. 12 - Prob. 19PCh. 12 - Review. While Lost-a-Lot ponders his next move in...Ch. 12 - John is pushing his daughter Rachel in a...Ch. 12 - Prob. 22PCh. 12 - Prob. 23PCh. 12 - A 10.0-kg monkey climbs a uniform ladder with...Ch. 12 - Prob. 25PCh. 12 - A steel wire of diameter 1 mm can support a...Ch. 12 - The deepest point in the ocean is in the Mariana...Ch. 12 - Assume Youngs modulus for bone is 1.50 1010 N/m2....Ch. 12 - A child slides across a floor in a pair of...Ch. 12 - Evaluate Youngs modulus for the material whose...Ch. 12 - Prob. 31PCh. 12 - When water freezes, it expands by about 9.00%....Ch. 12 - Prob. 33PCh. 12 - Prob. 34PCh. 12 - Prob. 35PCh. 12 - Review. A 30.0-kg hammer, moving with speed 20.0...Ch. 12 - A bridge of length 50.0 m and mass 8.00 104 kg is...Ch. 12 - A uniform beam resting on two pivots has a length...Ch. 12 - Prob. 39APCh. 12 - The lintel of prestressed reinforced concrete in...Ch. 12 - Prob. 41APCh. 12 - When a person stands on tiptoe on one foot (a...Ch. 12 - A hungry bear weighing 700 N walks out on a beam...Ch. 12 - Prob. 44APCh. 12 - A uniform sign of weight Fg and width 2L hangs...Ch. 12 - Prob. 46APCh. 12 - Prob. 47APCh. 12 - Assume a person bends forward to lift a load with...Ch. 12 - A 10 000-N shark is supported by a rope attached...Ch. 12 - Prob. 50APCh. 12 - A uniform beam of mass m is inclined at an angle ...Ch. 12 - Prob. 52APCh. 12 - When a circus performer performing on the rings...Ch. 12 - Figure P12.38 shows a light truss formed from...Ch. 12 - Prob. 55APCh. 12 - A stepladder of negligible weight is constructed...Ch. 12 - A stepladder of negligible weight is constructed...Ch. 12 - Prob. 58APCh. 12 - Two racquetballs, each having a mass of 170 g, are...Ch. 12 - Review. A wire of length L, Youngs modulus Y, and...Ch. 12 - Review. An aluminum wire is 0.850 m long and has a...Ch. 12 - Prob. 62APCh. 12 - A 500-N uniform rectangular sign 4.00 m wide and...Ch. 12 - A steel cable 3.00 cm2 in cross-sectional area has...Ch. 12 - Prob. 65CPCh. 12 - In the What If? section of Example 12.2, let d...Ch. 12 - Prob. 67CPCh. 12 - A uniform rod of weight Fg and length L is...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- While working on homework together, your friend stands her pencil on its sharpened tip. You say, Nice trick. How did you do it? Why do you think some trick had to be involved? Would you ask the same question if the pencil were lying on its side or if the pencil were unsharpened?arrow_forwardConsider a nanotube with a Youngs modulus of 2.130 1012 N/m2 that experiences a tensile stress of 5.3 1010 N/m2. Steel has a Youngs modulus of about 2.000 1011 Pa. How much stress would cause a piece of steel to experience the same strain as the nanotube?arrow_forward6arrow_forward
- l. Calculate F · dS where S F (3xy², xe², z³) and S is the surface of the solid bounded by the cylinder y + z² = 4 and the planes x = 5 and - 2.arrow_forwardYou use a millimeter ruler to measure the height of a rail curb. The bottom of the ruler is resting on the table. The place where the height measurement is taken is a little curved, so it is difficult to identify very precisely where the measurement should be taken. Also, it's a bit tricky to make sure the ruler is perfectly vertical (it might be leaning forward or backward a bit). When you take the measurement, you measure 12.9 mm. By moving the ruler a little, you are confident that the correct measurement is somewhere between 12.7 mm and 13.3 mm. Indicate the value that you will record in your logbook, as well as the uncertainty that you will attribute to it. Give your answers in mm. Warning: Keep at least 3 significant digits in your answers, even if it does not respect the rules of presentation of data in the laboratory.arrow_forwardYou use a millimeter ruler to measure the height of a rail curb. The bottom of the ruler is resting on the table. The place where the height measurement is taken is a little curved, so it is difficult to identify very precisely where the measurement should be taken. Also, it's a bit tricky to make sure the ruler is perfectly vertical (it might be leaning forward or backward a bit). When you take the measurement, you measure 12.9 mm. By moving the ruler a little, you are confident that the correct measurement is somewhere between 12.7 mm and 13.3 mm. Indicate the value that you will record in your logbook, as well as the uncertainty that you will attribute to it. Give your answers in mm.arrow_forward
- a) A 3.00-m-long, 290 N, uniform rod at the zoo is held in a horizontal position by two ropes at its ends in (Figure 1). The left rope makes an angle of 150∘ with the rod and the right rope makes an angle θ with the horizontal. A 86 N howler monkey (Alouatta seniculus) hangs motionless 0.50 m from the right end of the rod as he carefully studies you. a) Calculate the tension in the left rope. b) Calculate the tension in the right rope. c) Calculate the angle θ.arrow_forwardA flexible chain weighing 40.0 N hangs between two hooks located at the same height (as shown). At each hook, the tangent to the chain makes an angle θ = 42.0° with the horizontal. Find (a) the magnitude of the force each hook exerts on the chain and (b) the tension in the chain at its midpoint.Suggestion: For part (b), make a force diagram for half of the chain.arrow_forwardW A large block of wood has the shape of an isosceles trapezoid, as shown in the figure. The bottom end of the 1.79 m, and the top end has a block has a width of w %3D width w = 1.15 m. %3D If the center of gravity of the block is located a distance = 1.15 m directly above the midpoint of the base, what heg is the maximum tipping angle that the block can undergo before tumbling over? Wb maximum tipping angle: Assume the total height of the block is 2.485 m. If the block were resting on its narrow end, how far could it be tipped from that position without it falling over? maximum tipping angle:arrow_forward
- The bunchberry flower has the fastest-moving parts ever seen in a plant. Initially, the stamens are held by the petals in a bent position, storing energy like a coiled spring. As the petals release, the tips of the stamens fly up and quickly release a burst of pollen. Shown are the details of the motion. The tips of the stamens act like a catapult, flipping through a 60° angle; the times on the earlier photos show that this happens in just 0.30 ms. We can model a stamen tip as a 1.0-mm-long, 10 μg rigid rod with a 10 mg anther sac at one end and a pivot point at the opposite end. Though an oversimplification, we will model the motion by assuming the angular acceleration is constant throughout the motion. What is the speed of the anther sac as it releases its pollen?A. 3.5 m/s B. 7.0 m/sC. 10 m/s D. 14 m/sarrow_forwardThe bunchberry flower has the fastest-moving parts ever seen in a plant. Initially, the stamens are held by the petals in a bent position, storing energy like a coiled spring. As the petals release, the tips of the stamens fly up and quickly release a burst of pollen. Shown are the details of the motion. The tips of the stamens act like a catapult, flipping through a 60° angle; the times on the earlier photos show that this happens in just 0.30 ms. We can model a stamen tip as a 1.0-mm-long, 10 μg rigid rod with a 10 mg anther sac at one end and a pivot point at the opposite end. Though an oversimplification, we will model the motion by assuming the angular acceleration is constant throughout the motion. How large is the “straightening torque”? (You can omit gravitational forces from your calculation; the gravitational torque is much less than this.)A. 2.3 x 10-7 N ⋅ m B. 3.1 x 10-7 N ⋅ mC. 2.3 x 10-5 N ⋅ m D. 3.1 x 10-5 N ⋅ marrow_forwardThe bunchberry flower has the fastest-moving parts ever seen in a plant. Initially, the stamens are held by the petals in a bent position, storing energy like a coiled spring. As the petals release, the tips of the stamens fly up and quickly release a burst of pollen. Shown are the details of the motion. The tips of the stamens act like a catapult, flipping through a 60° angle; the times on the earlier photos show that this happens in just 0.30 ms. We can model a stamen tip as a 1.0-mm-long, 10 μg rigid rod with a 10 mg anther sac at one end and a pivot point at the opposite end. Though an oversimplification, we will model the motion by assuming the angular acceleration is constant throughout the motion. What is the angular acceleration of the anther sac during the motion?A. 3.5 x 103 rad/s2 B. 7.0 x 103 rad/s2C. 1.2 x 107 rad/s2 D. 2.3 x 107 rad/s2arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Principles of Physics: A Calculus-Based Text
Physics
ISBN:9781133104261
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Physics for Scientists and Engineers
Physics
ISBN:9781337553278
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...
Physics
ISBN:9781337553292
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...
Physics
ISBN:9781133939146
Author:Katz, Debora M.
Publisher:Cengage Learning
Fluids in Motion: Crash Course Physics #15; Author: Crash Course;https://www.youtube.com/watch?v=fJefjG3xhW0;License: Standard YouTube License, CC-BY