Chapter 12, Problem 5P

(a)

To determine

Draw the influence lines for the reactions $R_B,R_D,\text{and}{R}_F$ of the beam and the shear and

moment at E.

Explanation of Solution

Calculation:

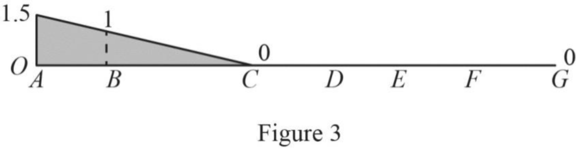
Influence line for reaction $R_B$.

Consider the portion AC $\left(0\le x<12\text{ft}\right)$.

Apply a 1 kip unit moving load at a distance of $x$ from A in the portion AC.

Sketch the free body diagram of beam as shown in Figure 1.

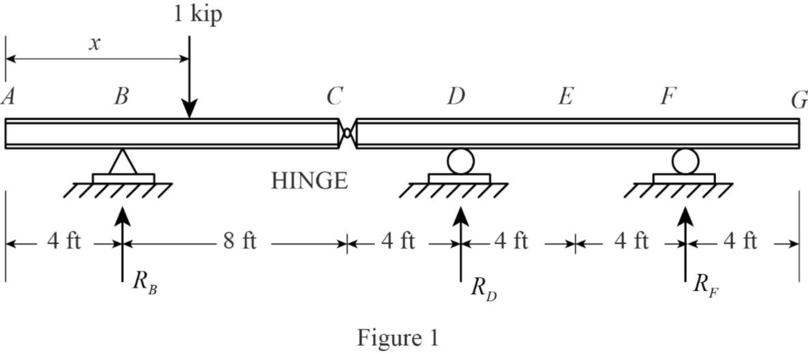
Refer Figure 1.

Consider clockwise moment as negative and anticlockwise moment as positive.

Find the equation support reaction $\left(R_B\right)$.

Take moment about point C from A.

$\begin{array}{l}\Sigma M_C=0\\ R_B\left(8\right)-1\left(12-x\right)=0\\ 8R_B=12-x\\ R_B=1.5-\frac{x}{8}\end{array}$

Consider the portion CG $\left(12\text{ft}<x\le 28\text{ft}\right)$.

Apply a 1 kip unit moving load at a distance of $x$ from A in the portion CG.

Sketch the free body diagram of beam as shown in Figure 2.

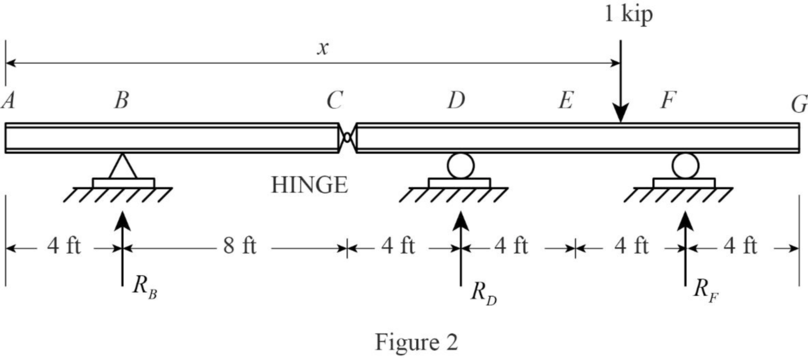
Refer Figure 2.

Find the equation support reaction $\left(R_B\right)$.

Take moment about point B from A.

$\begin{array}{l}\Sigma M_C=0\\ R_B\left(6\right)=0\\ R_B=0\end{array}$

Thus, the equations of the influence line for $R_B$ are,

$R_B=1.5-\frac{x}{8}0\le x<12\text{ft}$        (1)

$R_B=012\text{ft}<x\le 28\text{ft}$        (2)

Find the value of influence line ordinate of $R_B$ for various points of x using the Equations (1) and (2) and summarize the value as in Table 1.

Points	x $\left(\text{ft}\right)$	$R_C$
A	0	1.5
$B$	4	1
C	12	0
D	16	0
E	20	0
F	24	0
G	28	0

Draw the influence lines for $R_B$ using Table 1 as shown in Figure 3.

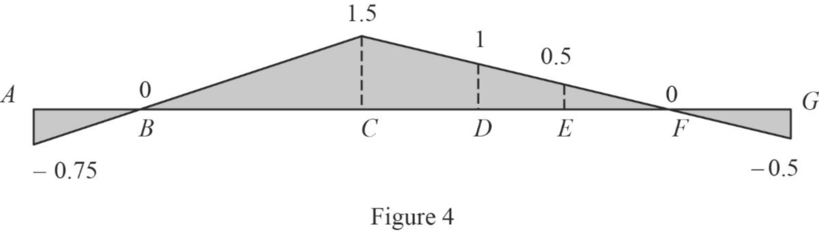
Influence line for reaction $R_D$.

Consider the portion AC $\left(0\le x<12\text{ft}\right)$.

Refer Figure 1.

Find the equation support reaction $\left(R_D\right)$.

The moment at F from G is zero.

Take moment about point C from A.

$\begin{array}{l}\Sigma M_F=0\\ R_B\left(20\right)-1\left(24-x\right)+R_D\left(8\right)=0\\ \left(1.5-\frac{x}{8}\right)\left(20\right)-24+x+8R_D=0\\ 30-2.5x-24+x+8R_D=0\\ 8R_D=1.5x-6\\ R_D=0.1875x-0.75\end{array}$

Consider the portion CG $\left(12\text{ft}<x\le 28\text{ft}\right)$.

Apply a 1 kip unit moving load at a distance of $x$ from A in the portion CF.

Refer Figure 2.

Find the equation support reaction $\left(R_D\right)$.

The moment at F from G is zero.

Take moment about point C from A.

$\begin{array}{l}\Sigma M_F=0\\ R_B\left(20\right)-1\left(24-x\right)+R_D\left(8\right)=0\\ \left(0\right)\left(20\right)-24+x+8R_D=0\\ -24+x+8R_D=0\\ 8R_D=24-x\\ R_D=3-\frac{x}{8}\end{array}$

Thus, the equations of the influence line for $R_D$ are,

$R_D=0.1875x-0.750\le x<12\text{ft}$        (3)

$R_D=3-\frac{x}{8}12\text{ft}<x\le 28\text{ft}$        (4)

Find the value of influence line ordinate of $R_D$ for various points of x using the Equations (3) and (4) and summarize the value as in Table 2.

Points	x $\left(\text{ft}\right)$	$R_D$
A	0	‑0.75
$B$	4	0
C	12	1.5
D	16	1
E	20	0.5
F	24	0
G	28	‑0.5

Draw the influence lines for $R_D$ using Table 2 as shown in Figure 4.

Influence line for reaction $R_F$.

Consider the portion AC $\left(0\le x<12\text{ft}\right)$.

Refer Figure 1.

Find the equation support reaction $\left(R_F\right)$.

Consider vertical equilibrium equation.

$\begin{array}{l}\Sigma F_y=0\\ R_B+R_D+R_F-1=0\\ \left(1.5-\frac{x}{8}\right)+\left(0.1875x-0.75\right)+R_F=1\\ 0.75+0.0625x+R_F=1\\ R_F=0.25-0.0625x\end{array}$

Consider the portion CG $\left(12\text{ft}<x\le 28\text{ft}\right)$.

Apply a 1 kip unit moving load at a distance of $x$ from A in the portion CF.

Refer Figure 2.

Find the equation support reaction $\left(R_F\right)$.

Consider vertical equilibrium equation.

$\begin{array}{l}\Sigma F_y=0\\ R_B+R_D+R_F-1=0\\ \left(0\right)+\left(3-\frac{x}{8}\right)+R_F=1\\ R_F=1-3+\frac{x}{8}\\ R_F=\frac{x}{8}-2\end{array}$

Thus, the equations of the influence line for $R_F$ are,

$R_F=0.25-0.0625x0\le x<12\text{ft}$        (5)

$R_F=\frac{x}{8}-212\text{ft}<x\le 28\text{ft}$        (6)

Find the value of influence line ordinate of $R_F$ for various points of x using the Equations (5) and (6) and summarize the value as in Table 3.

Points	x $\left(\text{ft}\right)$	$R_F$
A	0	0.25
$B$	4	0
C	12	‑0.5
D	16	0
E	20	0.5
F	24	1
G	28	1.5

Draw the influence lines for $R_F$ using Table 3 as shown in Figure 5.

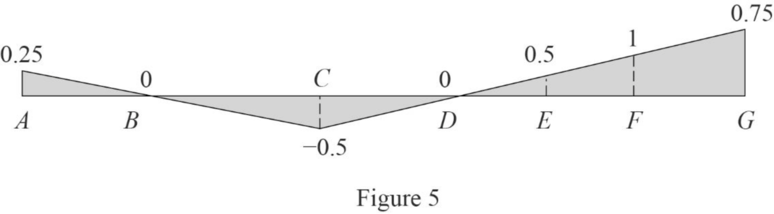
Influence line for the shear at section E:

Consider portion AC $\left(0\le x\le 12\text{ft}\right)$.

Find the equation of shear and moment at E for portion AC.

Apply a 1 kip in the portion AC from A.

Sketch the free body diagram of the section EG as shown in Figure 6.

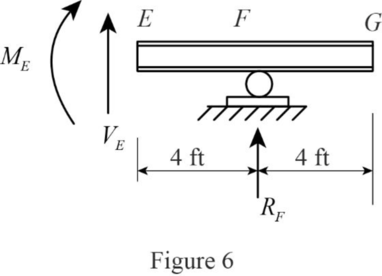
Find the equation of shear at E of portion AC.

$\begin{array}{l}{R}_F+V_E=0\\ V_E=-R_F\\ V_E=-\left(0.25-0.0625x\right)\\ V_E=0.0625x-0.25\end{array}$

Find the equation of moment at E of portion AC.

$\begin{array}{c}{R}_F\left(4\right)-M_E=0\\ M_E=\left(0.25-0.0625x\right)\left(4\right)\\ M_E=1-0.25x\end{array}$

Consider portion CE $\left(12\le x<20\text{ft}\right)$:

Find the equation of shear and moment at E for portion CE.

Apply a 1 kip in the portion CE from A.

Sketch the free body diagram of the section EG as shown in Figure 7.

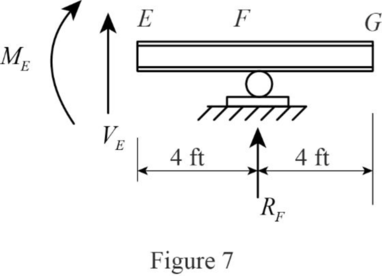
Refer Figure 7.

Find the equation of shear at E of portion CE.

$\begin{array}{l}{R}_F+V_E=0\\ V_E=-R_F\\ V_E=-\left(\frac{x}{8}-2\right)\\ V_E=2-0.125x\end{array}$

Find the equation of moment at E of portion CE.

$\begin{array}{l}{R}_F\left(4\right)-M_E=0\\ M_E=\left(\frac{x}{8}-2\right)\left(4\right)\\ M_E=0.5x-8\end{array}$

Consider portion EG $\left(20<x\le 28\text{ft}\right)$:

Find the equation of shear and moment at E for portion EG.

Apply a 1 kip in the portion EG from A.

Sketch the free body diagram of the section AE as shown in Figure 8.

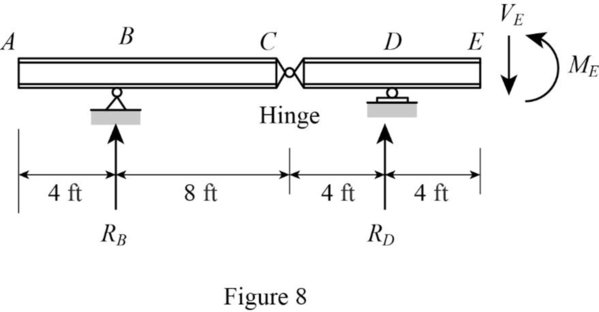
Refer Figure 8.

Find the equation of shear at E of portion EG.

$\begin{array}{l}{R}_B+R_D-V_E=0\\ V_E=R_B+R_D\\ V_E=0+\left(3-\frac{x}{8}\right)\\ V_E=3-0.125x\end{array}$

Find the equation of moment at E of portion EG.

$\begin{array}{l}{R}_B\left(16\right)+R_D\left(4\right)-M_E=0\\ 0\left(16\right)+\left(3-\frac{x}{8}\right)\left(4\right)-M_E=0\\ M_E=12-0.5x\end{array}$

Thus, the equations of the influence line for $V_E$ are,

$V_E=0.0625x-0.250\le x<12\text{ft}$        (7)

$V_E=2-0.125x12\text{ft}<x\le 20\text{ft}$        (8)

$V_E=3-0.125x20\text{ft}<x\le 28\text{ft}$        (9)

$M_E=1-0.25x0\le x<12\text{ft}$        (10)

$M_E=0.5x-812\text{ft}<x\le 20\text{ft}$        (11)

$M_E=12-0.5x20\text{ft}<x\le 28\text{ft}$        (12)

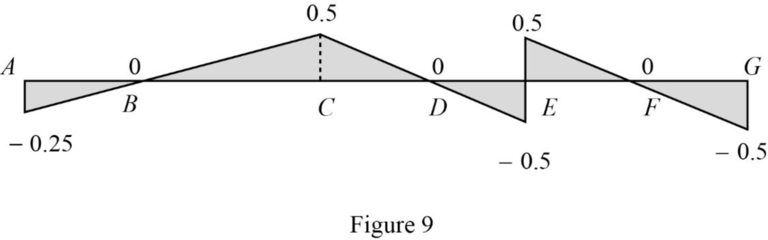
Find the value of influence line ordinate of shear force at E various points of x using the Equations (7), (8), and (9) and summarize the value as in Table 4.

Points	x $\left(\text{ft}\right)$	$V_E$
A	0	‑0.25
$B$	4	0
$C$	12	0.5
D	16	0
$E^{-}$	20	‑0.5
$E^{+}$	20	‑0.5
F	24	0
G	28	‑0.5

Draw the influence lines for the shear force at point B using Table 4 as shown in Figure 9.

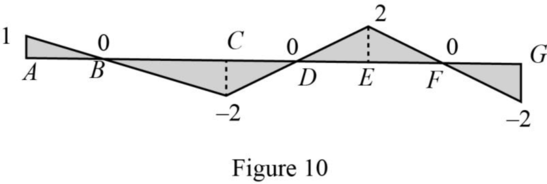
Find the value of influence line ordinate of moment at $E$ for various points of x using the Equation (10) (11), and (12) and summarize the value as in Table 5.

Points	x $\left(\text{ft}\right)$	$V_E$
A	0	1
$B$	4	0
$C$	12	‑2
D	16	0
$E$	20	2
F	24	0
G	28	‑2

Sketch the influence lines for the moment at point E using Table 5 as shown in Figure 10.

(b)

To determine

Determine the maximum positive and negative values of the reactions.

Explanation of Solution

Given Information:

The uniform load acts on the beam (w) is 1.2 kips/ft

Calculation:

Refer Figure 3.

Determine the maximum positive value of the reaction $R_B$.

$\begin{array}{c}{\left(R_B\right)}_{\max (+)}=A_{R_B(+)}\left(w\right)\\ =\left[\frac{1}{2}\left(12-0\right)\left(1.5\right)\right]\left(1.2\right)\\ =10.8\text{kips}\end{array}$

Therefore, the maximum positive value of the reaction $R_B$ is $\underset{\_}{10.8\text{kips}}$.

Determine the maximum negative value of the reaction $R_B$.

$\begin{array}{c}{\left(R_B\right)}_{\max (-)}=A_{R_A(-)}\left(w\right)\\ =\left[0\right]\left(1.2\right)\\ =0\end{array}$

Therefore, the maximum negative value of the reaction $R_B$ is $\underset{\_}{0}$.

Refer Figure 4.

Determine the maximum positive value of the reaction $R_D$.

$\begin{array}{c}{\left(R_D\right)}_{\max (+)}=A_{R_D(+)}\left(w\right)\\ =\left[\frac{1}{2}\left(24-4\right)\left(1.5\right)\right]\left(1.2\right)\\ =18\text{kips}\end{array}$

Therefore, the maximum positive value of the reaction $R_D$ is $\underset{\_}{18\text{kips}}$.

Determine the maximum negative value of the reaction $R_D$.

$\begin{array}{c}{\left(R_D\right)}_{\max (-)}=A_{R_D(-)}\left(w\right)\\ =\left[\frac{1}{2}\left(4-0\right)\left(-\frac{3}{4}\right)+\frac{1}{2}\left(28-24\right)\left(-\frac{1}{2}\right)\right]\left(1.2\right)\\ =3\text{kips}\end{array}$

Therefore, the maximum negative value of the reaction $R_D$ is $\underset{\_}{3\text{kips}}$.

Refer Figure 5.

Determine the maximum positive value of the reaction $R_F$.

$\begin{array}{c}{\left(R_F\right)}_{\max (+)}=A_{R_F(+)}\left(w\right)\\ =\left[\frac{1}{2}\left(4-0\right)\left(0.25\right)+\frac{1}{2}\left(28-16\right)\left(1.5\right)\right]\left(1.2\right)\\ =11.4\text{kips}\end{array}$

Therefore, the maximum positive value of the reaction ${\left(R_F\right)}_{\max (+)}$ is $\underset{\_}{11.4\text{kips}}$.

Determine the maximum negative value of the reaction $M_A$.

$\begin{array}{c}{\left(R_F\right)}_{\max (-)}=A_{R_F(-)}\left(w\right)\\ =\left[\frac{1}{2}\left(16-4\right)\left(-0.5\right)\right]\left(1.2\right)\\ =-3.6\text{kips}\end{array}$

Therefore, the maximum negative value of the reaction ${\left(R_F\right)}_{\max (-)}$ is $\underset{\_}{-3.6\text{kips}}$.