
Concept explainers
Mechanical/Aerospace Engineering
Consider the three mass-four spring system in Fig. P12.37. Determining the equations of motion from
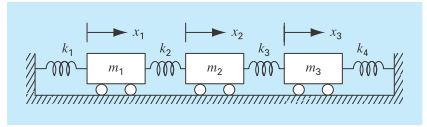
FIGURE P12.37
Where
At a specific time when x 1 5 0.05 m, x 2 5 0.04 m, and x 3 5 0.03 m, this forms a tridiagonal matrix. Solve for the acceleration of each mass.
Want to see the full answer?
Check out a sample textbook solution
Chapter 12 Solutions
Numerical Methods for Engineers
- 18) Find all the complex cube roots of -2i. Leave your answers in polar form with the argument in degrees.arrow_forward9) Write an equation for the hyperbola. 2+ -6-5-4-3-2 -2- -4- -5+ + 23 45 6xarrow_forward8) Find an equation for the hyperbola with vertices at vertices at (±7, 0) and foci at (±9, 0).arrow_forward
- 3) Find an equation for the parabola with a vertex at (1, 2) and focus at (1, 4). A) (x - 1)² = 8(y-2) C) (x - 1)² = -8(y - 2) B) (y - 2)² = -12(x − 1) - D) (y - 2)² = 12(x-1)arrow_forward2) Graph the equation. y² = 12x 5 10 -10 -5 5 + 10 xarrow_forward15) Solve the triangles if possible. B = 11°, b = 15.5, a = 40.62arrow_forward
 Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
