
Concept explainers
(a)
To find: A quadratic model using a graphic utility.
(a)
Answer to Problem 67E
A quadratic model is
Explanation of Solution
Given:
The data set is:
| Year | Revenue |
| 2007 | 7.67 |
| 2008 | 8.54 |
| 2009 | 8.73 |
| 2010 | 9.16 |
| 2011 | 11.65 |
| 2012 | 14.07 |
| 2013 | 16.05 |
Calculation:
Follow the provided steps of Ti-83 plus calculator to compute a quadraticas:
- Turn on the calculator.
- Click on STAT > Edit > Enter.
- Enter the data of x in L1 and data of y in L2.
- Click om STAT > CALC >QuadReg> ENTER.
- Hit 2nd and 1 to choose L1 and Hit 2nd and 2 to choose L2.
- Hit Enter.
The obtained output is:
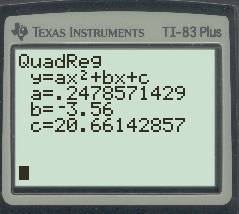
From the above output, the quadratic model is
(b)
To construct: The model using the graphing utility. Also, estimate the slope of the graph if t is 12 and interpret the result.
(b)
Answer to Problem 67E
The slope is 13.6534.
Explanation of Solution
Graph:
Follow the provided steps of Ti-83 plus calculator to construct the figure:
- Turn on the calculator.
- Click on STAT > Edit > Enter.
- Hit Y= and enter the regression.
- Press 2nd key and Y and press twice and chose the mark of rectangle.
- Hit zoom button and 9th key.
The obtained output is:
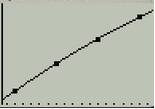
Calculation:
From the above part, the quadratic model is:
Then the slope is approximately 13.6534.
It implies that the population is increasing by the rate of 1365.34 per year
(c)
To find: The derivative of the model computed in part (a) and evaluate it for t = 12
(c)
Answer to Problem 67E
The value is 2.392.
Explanation of Solution
Calculation:
The quadratic model is:
Now, take the derivative of above function with respect to t as:
Now, plug in t = 12 in above expression as:
Thus, the required value is 2.392.
(d)
To draw:The conclusion regarding the results the previous parts.
(d)
Explanation of Solution
Since, the quadratic model is:
And, its derivative is:
Because it is depending on the time. Thus, it could be said that it is a good model
Chapter 11 Solutions
Precalculus with Limits: A Graphing Approach
- Can you answer this question and give step by step and why and how to get it. Can you write it (numerical method)arrow_forwardCan you answer this question and give step by step and why and how to get it. Can you write it (numerical method)arrow_forwardThere are three options for investing $1150. The first earns 10% compounded annually, the second earns 10% compounded quarterly, and the third earns 10% compounded continuously. Find equations that model each investment growth and use a graphing utility to graph each model in the same viewing window over a 20-year period. Use the graph to determine which investment yields the highest return after 20 years. What are the differences in earnings among the three investment? STEP 1: The formula for compound interest is A = nt = P(1 + − − ) n², where n is the number of compoundings per year, t is the number of years, r is the interest rate, P is the principal, and A is the amount (balance) after t years. For continuous compounding, the formula reduces to A = Pert Find r and n for each model, and use these values to write A in terms of t for each case. Annual Model r=0.10 A = Y(t) = 1150 (1.10)* n = 1 Quarterly Model r = 0.10 n = 4 A = Q(t) = 1150(1.025) 4t Continuous Model r=0.10 A = C(t) =…arrow_forward
- Use a graphing utility to find the point of intersection, if any, of the graphs of the functions. Round your result to three decimal places. (Enter NONE in any unused answer blanks.) y = 100e0.01x (x, y) = y = 11,250 ×arrow_forward5. For the function y-x³-3x²-1, use derivatives to: (a) determine the intervals of increase and decrease. (b) determine the local (relative) maxima and minima. (e) determine the intervals of concavity. (d) determine the points of inflection. (e) sketch the graph with the above information indicated on the graph.arrow_forwardCan you solve this 2 question numerical methodarrow_forward
- 1. Estimate the area under the graph of f(x)-25-x from x=0 to x=5 using 5 approximating rectangles Using: (A) right endpoints. (B) left endpoints.arrow_forward9. Use fundamental theorem of calculus to find the derivative d a) *dt sin(x) b)(x)√1-2 dtarrow_forward3. Evaluate the definite integral: a) √66x²+8dx b) x dx c) f*(2e* - 2)dx d) √√9-x² e) (2-5x)dx f) cos(x)dx 8)²₁₂√4-x2 h) f7dx i) f² 6xdx j) ²₂(4x+3)dxarrow_forward
- 2. Consider the integral √(2x+1)dx (a) Find the Riemann sum for this integral using right endpoints and n-4. (b) Find the Riemann sum for this same integral, using left endpoints and n=4arrow_forwardProblem 11 (a) A tank is discharging water through an orifice at a depth of T meter below the surface of the water whose area is A m². The following are the values of a for the corresponding values of A: A 1.257 1.390 x 1.50 1.65 1.520 1.650 1.809 1.962 2.123 2.295 2.462|2.650 1.80 1.95 2.10 2.25 2.40 2.55 2.70 2.85 Using the formula -3.0 (0.018)T = dx. calculate T, the time in seconds for the level of the water to drop from 3.0 m to 1.5 m above the orifice. (b) The velocity of a train which starts from rest is given by the fol- lowing table, the time being reckoned in minutes from the start and the speed in km/hour: | † (minutes) |2|4 6 8 10 12 14 16 18 20 v (km/hr) 16 28.8 40 46.4 51.2 32.0 17.6 8 3.2 0 Estimate approximately the total distance ran in 20 minutes.arrow_forwardX Solve numerically: = 0,95 In xarrow_forward
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning





