
An air conditioner operates on the vapor-compression refrigeration cycle with refrigerant-134a as the refrigerant. The air conditioner is used to keep a space at 21°C while rejecting the waste heat to the ambient air at 37°C. The refrigerant enters the compressor at 180 kPa superheated by 2.7°C at a rate of 0.06 kg/s and leaves the compressor at 1200 kPa and 60°C. R-134a is subcooled by 6.3°C at the exit of the condenser. Determine (a) the rate of cooling provided to the space, in Btu/h, and the COP, (b) the isentropic efficiency and the exergy efficiency of the compressor, (c) the exergy destruction in each component of the cycle and the total exergy destruction in the cycle, and (d) the minimum power input and the second-law efficiency of the cycle.
(a)
The rate of cooling provided to the space.
The coefficient of performance of the air conditioner.
Answer to Problem 118RP
The rate of cooling provided to the space is
The coefficient of performance of the air conditioner is
Explanation of Solution
Show the T-s diagram as in Figure (1).
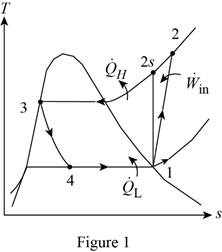
Express the refrigeration load.
Here, specific enthalpy at state 1 and 4 is
Express the rate of work input.
Express the coefficient of performance of the air conditioner.
Conclusion:
Perform the unit conversion of pressure at state 1 from
Refer to Table A-13, obtain the saturation temperature at a pressure of 0.18 MPa as
Calculate the temperature at state 1.
Substitute
Refer Table A-13, “superheated refrigerant-134a”, and write the properties corresponding to pressure and inlet temperature of
Here, specific enthalpy and entropy is
Write the formula of interpolation method of two variables.
Here, the variables denote by x and y is specific entropy at state 2 and specific enthalpy at state 2s respectively.
Show the specific enthalpy at state 2s corresponding to specific entropy by referring the table A-13 at pressure of 1.2 MPa and specific entropy of
|
Specific entropy at state 2 |
Specific enthalpy at state 2s |
| 0.9268 | 278.28 |
| 0.9484 | |
| 0.9615 | 289.66 |
Substitute the Table (1) values in Equation (IV) to get 285.34 kJ/kg.
Thus, the specific enthalpy at state 2s is,
Refer Table A-13, “superheated refrigerant-134a”, and write the properties corresponding to exit state of pressure and temperature of
Here, specific enthalpy and entropy at exit state are
Refer to Table A-13, obtain the saturation temperature at a pressure of 1.2 MPa as
Refer Table A-13, “superheated refrigerant-134a”, and write the properties corresponding to state 3 of pressure and temperature of
Here, specific enthalpy and entropy at state 3 are
Refer Table A-13, “superheated refrigerant-134a”, and write the properties corresponding to state 4 of pressure and specific enthalpy of
Substitute 0.06 kg/s for
Thus, the rate of cooling provided to the space is
Substitute 0.06 kg/s for
Substitute 2.670 kW for
Thus, the coefficient of performance of the air conditioner is
(b)
The isentropic efficiency of the compressor.
The exergy efficiency of the compressor.
Answer to Problem 118RP
The isentropic efficiency of the compressor is
The exergy efficiency of the compressor is
Explanation of Solution
Express the isentropic efficiency of the compressor.
Here, specific enthalpy at state 2s is
Calculate the reversible power for the compressor.
Here, ambient air temperature is
Calculate the exergy efficiency for the compressor.
Conclusion:
Substitute 285.34 kJ/kg for
Thus, the isentropic efficiency of the compressor is
Substitute 0.06 kg/s for
Substitute 2.428 kW for
Thus, the exergy efficiency of the compressor is
(c)
The exergy destruction in each component of the cycle.
The total exergy destruction in the cycle.
Answer to Problem 118RP
The exergy destruction in each component of the cycle are
The total exergy destruction in the cycle is
Explanation of Solution
Compressor
Express the entropy generation for cycle 1-2.
Express the exergy destruction for the cycle 1-2.
Condenser
Express the entropy generation for cycle 2-3.
Here, the rate of heat rejected is
Calculate the value of
Substitute
Express the exergy destruction for the cycle 2-3.
Expansion valve
Express the entropy generation for cycle 3-4.
Express the exergy destruction for the cycle 3-4.
Evaporator
Express the entropy generation for cycle 4-1.
Here, temperature at refrigeration load is
Express the exergy destruction for the cycle 4-1.
Calculate the total exergy destruction.
Conclusion:
Substitute 0.06 kg/s for
Substitute
Substitute 0.06 kg/s for
Substitute
Substitute 0.06 kg/s for
Substitute
Substitute 0.06 kg/s for
Substitute
Thus, the exergy destruction in each component of the cycle are
Substitute 0.2426 kW for
Thus, the total exergy destruction in the cycle is
(d)
The minimum power input of the cycle.
The second law efficiency of the cycle.
Answer to Problem 118RP
The minimum power input of the cycle is
The second law efficiency of the cycle is
Explanation of Solution
Express the exergy of the heat transferred from the low-temperature medium.
Here, minimum power input of the cycle is the exergy of the heat transferred from the low-temperature medium.
Calculate the second law efficiency of the cycle.
Calculate the total exergy destruction in the cycle.
Conclusion:
Substitute 8.213 kW for
Thus, the minimum power input to the cycle is
Substitute 0.4470 kW for
Thus, the second law efficiency of the cycle is
Want to see more full solutions like this?
Chapter 11 Solutions
THERMODYNAMICS-SI ED. EBOOK >I<
- what is an air preheater, what are formulas, and their importance, define the diagram, and give me a script on how to explain the design of an air preheater, and how did values end up in that number. based on standardsarrow_forwardQf, Qa,Qm, Qcon,Qfg, Qbd, Qref,Qloss ( meaning, formula, percentage, and importance of higher value na qf, qa etc)arrow_forwardThe beam is supported by a fixed support at point C and a roller at point A. It also has an internal hinge at point B. The beam supports a point load at point D, a moment at point A and a distributed load on segment BC. a. calculate the support reactions at points A and C b. calculate the internal resultant loadings (N, V, M) at points E and F, which lies in the middle between points A and D P = 4 kip Ma = 5 kip-ft w1 = 3 kip/ft and w2 = 4 kip/ft a = 3 ftarrow_forward
- From the image of the pyramid, I want to find what s1 hat, s2 hat, and s3 hat are. I think s3 hat is just equal to e3 hat right? What about the others?arrow_forward(a) What kind of equation is it?(b) Is it linear or non-linear?(c) Is it a coupled system or uncoupled?arrow_forwardWhat kind of system is presented in Figure 2? Open loop or closed loop?arrow_forward
- What are the control hardware shown in the Figure?arrow_forwardQuestion 1. A tube rotates in the horizontal ry plane with a constant angular velocity w about the z-axis. A particle of mass m is released from a radial distance R when the tube is in the position shown. This problem is based on problem 3.2 in the text. R m 2R Figure 1 x a) Draw a free body diagram of the particle if the tube is frictionless. b) Draw a free body diagram of the particle if the coefficient of friction between the sides of the tube and the particle is = k = p. c) For the case where the tube is frictionless, what is the radial speed at which the particle leaves the tube? d) For the case where there is friction, derive a differential equation that would allow you to solve for the radius of the particle as a function of time. I'm only looking for the differential equation. DO NOT solve it. 1 e) If there is no friction, what is the angle of the tube when the particle exits? • Hint: You may need to solve a differential equation for the last part. The "potentially useful…arrow_forwardQuestion 2. A smooth uniform sphere of mass m and radius r is squeezed between two massless levers, each of length 1, which are inclined at an angle with the vertical. A mechanism at pivot point O ensures that the angles & remain the same at all times so that the sphere moves straight upward. This problem is based on Problem 3-1 in the text. P P r Figure 2 a) Draw appropriate freebody diagrams of the system assuming that there is no friction. b) Draw appropriate freebody diagrams of the system assuming that there is a coefficient of friction between the sphere and the right lever of μ. c) If a force P is applied between the ends of the levers (shown in the diagram), and there is no friction, what is the acceleration of the sphere when = 30°arrow_forward
- If you had a matrix A = [1 2 3; 4 5 6; 7 8 9] and a matrix B = [1 2 3], how would you cross multiply them i.e. what is the cross product of AxB. what would be the cross product of a dyadic with a vector?arrow_forwardProblem 3: The inertia matrix can be written in dyadic form which is particularly useful when inertia information is required in various vector bases. On the next page is a right rectangular pyramid of total mass m. Note the location of point Q. (a) Determine the inertia dyadic for the pyramid P, relative to point Q, i.e., 7%, for unit vectors ₁₁, 2, 3.arrow_forwardCan you solve for v? Also, what is A x uarrow_forward
 Refrigeration and Air Conditioning Technology (Mi...Mechanical EngineeringISBN:9781305578296Author:John Tomczyk, Eugene Silberstein, Bill Whitman, Bill JohnsonPublisher:Cengage Learning
Refrigeration and Air Conditioning Technology (Mi...Mechanical EngineeringISBN:9781305578296Author:John Tomczyk, Eugene Silberstein, Bill Whitman, Bill JohnsonPublisher:Cengage Learning
