Finite Mathematics for Business, Economics, Life Sciences and Social Sciences
14th Edition
ISBN: 9780134677972
Author: Barnett
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 11.1, Problem 45E
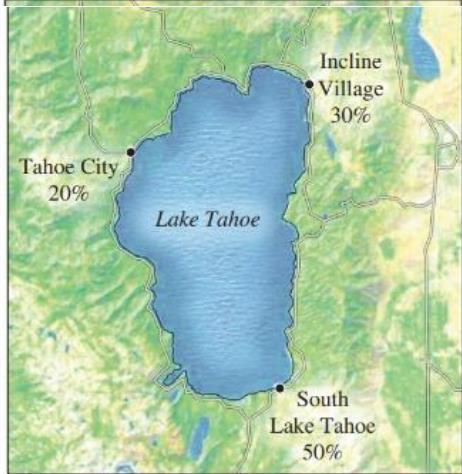
Store location two competitive pet shops want to open stores at Lake Tahoe, where there are currently no pet shops. The following figure shows the percentages of the total Tahoe population serviced by each of the three main business centers. If both shops open in the same business center, then they split all the business equally; if they open in two different centers, then they each get all the business in the center in which they open plus half the business in the third center. Where should the two pet shops open? Set up a game matrix and solve.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Can you help me with D and E
Q1.1
1 Point
Any set {V1, V2, V3, V4} that consists of four different vectors from R cannot possibly span Rº.
True
False
Save Answer
Find:
lim x →-6 f (x)
limx-4 f (x)
lim x-1 f (x)
lim x →4 f (x)
(-6,3) •
(-1,5)
-8
-7
(-6,-2)
4+
(4,5)
(4,2) •
(-1,1)
-6
Chapter 11 Solutions
Finite Mathematics for Business, Economics, Life Sciences and Social Sciences
Ch. 11.1 - Suppose that a and k are both saddle values of the...Ch. 11.1 - Repeat Example 1 for the HDTV game matrix...Ch. 11.1 - Determine which of the matrix games below are...Ch. 11.1 - In Problems 1-8, is the matrix game strictly...Ch. 11.1 - In Problems 1-8, is the matrix game strictly...Ch. 11.1 - In Problems 1-8, is the matrix game strictly...Ch. 11.1 - In Problems 1-8, is the matrix game strictly...Ch. 11.1 - In Problems 1-8, is the matrix game strictly...Ch. 11.1 - In Problems 1-8, is the matrix game strictly...Ch. 11.1 - In Problems 1-8, is the matrix game strictly...
Ch. 11.1 - In Problems 1-8, is the matrix game strictly...Ch. 11.1 - In Problems 9-16, the matrix for a strictly...Ch. 11.1 - In Problems 9-16 , the matrix for a strictly...Ch. 11.1 - In Problems 9-16, the matrix for a strictly...Ch. 11.1 - In Problems 9-16, the matrix for a strictly...Ch. 11.1 - In Problems 9-16, the matrix for a strictly...Ch. 11.1 - In Problems 9-16, the matrix for a strictly...Ch. 11.1 - In Problems 9-16, the matrix for a strictly...Ch. 11.1 - In Problems 9-16, the matrix for a strictly...Ch. 11.1 - Prob. 17ECh. 11.1 - Prob. 18ECh. 11.1 - Prob. 19ECh. 11.1 - Prob. 20ECh. 11.1 - Prob. 21ECh. 11.1 - Prob. 22ECh. 11.1 - Prob. 23ECh. 11.1 - Prob. 24ECh. 11.1 - Prob. 25ECh. 11.1 - Prob. 26ECh. 11.1 - Prob. 27ECh. 11.1 - Prob. 28ECh. 11.1 - Prob. 29ECh. 11.1 - Prob. 30ECh. 11.1 - Prob. 31ECh. 11.1 - Prob. 32ECh. 11.1 - For the matrix game of Problem 31, would you...Ch. 11.1 - For the matrix game of Problem 32, would you...Ch. 11.1 - In Problems 35-40, discuss the validity of each...Ch. 11.1 - In Problems 35-40, discuss the validity of each...Ch. 11.1 - In Problems 35-40, discuss the validity of each...Ch. 11.1 - In Problems 35-40, discuss the validity of each...Ch. 11.1 - In Problems 35-40, discuss the validity of each...Ch. 11.1 - In Problems 35-40, discuss the validity of each...Ch. 11.1 - Is there a value of m such that the following is...Ch. 11.1 - Prob. 42ECh. 11.1 - Price war a small town on a major highway has only...Ch. 11.1 - Investment Suppose that you want to invest $10,000...Ch. 11.1 - Store location two competitive pet shops want to...Ch. 11.1 - Store location Two competing auto parts companies...Ch. 11.2 - Let M=abcd (A) Show that if the row minima belong...Ch. 11.2 - (A) Using Theorem 4, give conditions on a,b,c, and...Ch. 11.2 - Solve the following version of the two-finger...Ch. 11.2 - Solve the matrix game: M=112324113Ch. 11.2 - In Problems 1-8, calculate the matrix product. (If...Ch. 11.2 - In Problems 1-8, calculate the matrix product. (If...Ch. 11.2 - In Problems 1-8, calculate the matrix product. (If...Ch. 11.2 - In Problems 1-8, calculate the matrix product. (If...Ch. 11.2 - In Problems 1-8, calculate the matrix product. (If...Ch. 11.2 - In Problems 1-8, calculate the matrix product. (If...Ch. 11.2 - In Problems 1-8, calculate the matrix product. (If...Ch. 11.2 - In Problems 1-8, calculate the matrix product. (If...Ch. 11.2 - In Problems 9-18, which rows and columns of the...Ch. 11.2 - In Problems 9-18, which rows and columns of the...Ch. 11.2 - In Problems 9-18, which rows and columns of the...Ch. 11.2 - In Problems 9-18, which rows and columns of the...Ch. 11.2 - In Problems 9-18, which rows and columns of the...Ch. 11.2 - In Problems 9-18, which rows and columns of the...Ch. 11.2 - In Problems 9-18, which rows and columns of the...Ch. 11.2 - In Problems 9-18, which rows and columns of the...Ch. 11.2 - In Problems 9-18, which rows and columns of the...Ch. 11.2 - In Problems 9-18, which rows and columns of the...Ch. 11.2 - Solve the matrix games in Problems 19-32,...Ch. 11.2 - Solve the matrix games in Problems 19-32,...Ch. 11.2 - Solve the matrix games in Problems 19-32,...Ch. 11.2 - Solve the matrix games in Problems 19-32,...Ch. 11.2 - Solve the matrix games in Problems 19-32,...Ch. 11.2 - Solve the matrix games in Problems 19-32,...Ch. 11.2 - Solve the matrix games in Problems 19-32,...Ch. 11.2 - Solve the matrix games in Problems 19-32,...Ch. 11.2 - Solve the matrix games in Problems 19-32,...Ch. 11.2 - Solve the matrix games in Problems 19-32,...Ch. 11.2 - Solve the matrix games in Problems 19-32,...Ch. 11.2 - Solve the matrix games in Problems 19-32,...Ch. 11.2 - Solve the matrix games in Problems 19-32,...Ch. 11.2 - Solve the matrix games in Problems 19-32,...Ch. 11.2 - In Problems 33-38, discuss the validity of each...Ch. 11.2 - In Problems 33-38, discuss the validity of each...Ch. 11.2 - In Problems 33-38, discuss the validity of each...Ch. 11.2 - In Problems 33-38, discuss the validity of each...Ch. 11.2 - In Problems 33-38, discuss the validity of each...Ch. 11.2 - In Problems 33-38, discuss the validity of each...Ch. 11.2 - You R and a friend C are playing the following...Ch. 11.2 - You R and a friend C are playing the following...Ch. 11.2 - For M=abcdP=p1p2Q=q1q2 Show that PMQ=EP,QCh. 11.2 - Using the fundamental theorem of game theory,...Ch. 11.2 - Show non strictly that the determined solution...Ch. 11.2 - Show that if a 22 matrix game has a saddle value,...Ch. 11.2 - Explain how to construct a 22 matrix game M for...Ch. 11.2 - Explain how to construct a 22 matrix game M for...Ch. 11.2 - In Problems 47 and 48, derive the formulas of...Ch. 11.2 - In Problems 47 and 48, derive the formulas of...Ch. 11.2 - Bank promotion A town has only two banks, bank R...Ch. 11.2 - Viewer ratings A city has two competitive...Ch. 11.2 - Investment You have inherited $10,000 just prior...Ch. 11.2 - Corporate farming For a one-time play...Ch. 11.3 - Show that M=1132 is a strictly determined matrix...Ch. 11.3 - Solve the following matrix game using geometric...Ch. 11.3 - In problem 1-6, find the smallest integer k0 such...Ch. 11.3 - In problem 1-6, find the smallest integer k0 such...Ch. 11.3 - In problem 1-6, find the smallest integer k0 such...Ch. 11.3 - In problem 1-6, find the smallest integer k0 such...Ch. 11.3 - In problem 1-6, find the smallest integer k0 such...Ch. 11.3 - In problem 1-6, find the smallest integer k0 such...Ch. 11.3 - In problem 7-12, solve the matrix game using a...Ch. 11.3 - In problem 7-12, solve the matrix game using a...Ch. 11.3 - In problem 7-12, solve the matrix game using a...Ch. 11.3 - In problem 7-12, solve the matrix game using a...Ch. 11.3 - In problem 7-12, solve the matrix game using a...Ch. 11.3 - In problem 7-12, solve the matrix game using a...Ch. 11.3 - Is there a better way to solve the matrix game in...Ch. 11.3 - Is there a better way to solve the matrix game in...Ch. 11.3 - Explain why the value of a matrix game is positive...Ch. 11.3 - Explain why the value of a matrix game is negative...Ch. 11.3 - In Problem 17-20, discuss the validity of each...Ch. 11.3 - In Problem 17-20, discuss the validity of each...Ch. 11.3 - In Problem 17-20, discuss the validity of each...Ch. 11.3 - In Problem 17-20, discuss the validity of each...Ch. 11.3 - In Problems 21-24 remove recessive rows and...Ch. 11.3 - In Problems 21-24 remove recessive rows and...Ch. 11.3 - In Problems 21-24 remove recessive rows and...Ch. 11.3 - In Problems 21-24 remove recessive rows and...Ch. 11.3 - (A) Let P and Q be strategies for the 22 matrix...Ch. 11.3 - Use properties of matrix addition and...Ch. 11.3 - Solve the matrix games in problems 27-30 by using...Ch. 11.3 - Solve the matrix games in problems 27-30 by using...Ch. 11.3 - Solve the matrix games in problems 27-30 by using...Ch. 11.3 - Solve the matrix games in problems 27-30 by using...Ch. 11.4 - Outline a procedure for solving the 45 matrix game...Ch. 11.4 - Suppose that the investor in Example 1 wishes to...Ch. 11.4 - In Problems 1-4, solve each matrix game 140012Ch. 11.4 - In Problems 1-4, solve each matrix game. 112201Ch. 11.4 - In Problems 1-4, solve each matrix game. 012103230Ch. 11.4 - In Problems 1-4, solve each matrix game. 120012201Ch. 11.4 - In Problems 5-8, outline a procedure for solving...Ch. 11.4 - In Problems 5-8, outline a procedure for solving...Ch. 11.4 - In Problems 5-8, outline a procedure for solving...Ch. 11.4 - In Problems 5-8, outline a procedure for solving...Ch. 11.4 - Scissors, paper ,stone game This game is well...Ch. 11.4 - Player R has a $2, a $5,and a $10 bill. Player C...Ch. 11.4 - Headphone sales. A department store chain is about...Ch. 11.4 - Tour agency A tour agency organizes standard and...Ch. 11 - In Problems 1 and 2, is the matrix game strictly...Ch. 11 - In Problems 1 and 2, is the matrix game strictly...Ch. 11 - In Problems 3-8, determine the value V of the...Ch. 11 - In Problems 3-8, determine the value V of the...Ch. 11 - In Problems 3-8, determine the value V of the...Ch. 11 - In Problems 3-8, determine the value V of the...Ch. 11 - In Problems 3-8, determine the value V of the...Ch. 11 - In Problems 3-8, determine the value V of the...Ch. 11 - Prob. 9RECh. 11 - Prob. 10RECh. 11 - Prob. 11RECh. 11 - Prob. 12RECh. 11 - Delete as many recessive rows and columns as...Ch. 11 - Problems 14-17 refer to the matrix game: M=2101...Ch. 11 - Problems 14-17 refer to the matrix game: M=2101...Ch. 11 - Problems 14-17 refer to the matrix game: M=2101...Ch. 11 - Problems 14-17 refer to the matrix game: M=2101...Ch. 11 - In Problems 18-21, discuss the validity of each...Ch. 11 - In Problems 18-21, discuss the validity of each...Ch. 11 - In Problems 18-21, discuss the validity of each...Ch. 11 - In Problems 18-21, discuss the validity of each...Ch. 11 - In Problems 22-26, solve each matrix game (first...Ch. 11 - In Problems 22-26, solve each matrix game (first...Ch. 11 - In Problems 22-26, solve each matrix game (first...Ch. 11 - In Problems 22-26, solve each matrix game (first...Ch. 11 - In Problems 22-26, solve each matrix game (first...Ch. 11 - Does every strictly determined 22 matrix game have...Ch. 11 - Does every strictly determined 33 matrix game have...Ch. 11 - Finger game Consider the following finger game...Ch. 11 - Refer to Problem 29. Use linear programming and a...Ch. 11 - Agriculture A farmer decides each spring whether...Ch. 11 - Agriculture Refer to Problem 31. Use formulas from...Ch. 11 - Advertising A small town has two competing grocery...Ch. 11 - Advertising Refer to Problem 33. Use linear...
Additional Math Textbook Solutions
Find more solutions based on key concepts
In Exercises 5–20, conduct the hypothesis test and provide the test statistic and the P-value and /or critical ...
Elementary Statistics (13th Edition)
Fill in each blank so that the resulting statement is true. An equation that expresses a relationship between t...
Algebra and Trigonometry (6th Edition)
Assessment 1-1A In a big red box, there are 7 smaller blue boxes. In each of the blue boxes, there are 7 black ...
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
Logarithmic differentiation Use logarithmic differentiation to evaluate f(x). 61. f(x)=(x+1)10(2x4)8
Calculus: Early Transcendentals (2nd Edition)
Fill in each blank so that the resulting statement is true. If n is a counting number, bn, read ______, indicat...
College Algebra (7th Edition)
The following set of data is from sample of n=5: a. Compute the mean, median, and mode. b. Compute the range, v...
Basic Business Statistics, Student Value Edition
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- 3 2 Find: ƒ(1) lim f(x) 14-x 2 ƒ(2) lim f(x) x-2- lim f(x) x+2+ lim f(x) x→4 3 y=f(x)arrow_forwardFor each graph below, state whether it represents a function. Graph 1 24y Graph 2 Graph 3 4 2 -8 -6 -4 -2 -2 2 4 6 Function? ○ Yes ○ No ○ Yes ○ No Graph 4 Graph 5 8 Function? Yes No Yes No -2. ○ Yes ○ No Graph 6 4 + 2 4 -8 -6 -4 -2 2 4 6 8 Yes -4++ Noarrow_forwardStudents were asked to simplify the expression (secØ - cosØ)/secØ Two students' work is given.Student A: step 1 secØ/secØ - cosØ/secØstep 2 cosØ/1 - (1/cosØ)step 3 1 - cos^2Østep 4 sin^2ØStudent B: step 1 (1/cosØ)-cosØ)/secØstep 2 (1 - cos^2Ø/cosØ)/secØstep 3 sin^2Ø/cos^2Østep 4 tan^2ØPart A: Which student simplified the expression incorrectly? Explain the errors that were made or the formulas that were misused.Part B: Complete the student's solution correctly, beginning with the location of the error.arrow_forward
- Although 330° is a special angle on the unit circle, Amar wanted to determine its coordinates using the sum and difference formulas.Part A: Determine cos 330° using the cosine sum identity. Be sure to include all necessary work.Part B: Determine sin 330° using the sine difference identity. Be sure to include all necessary work.arrow_forwardA public health researcher is studying the impacts of nudge marketing techniques on shoppers vegetablesarrow_forward4. Let A {w, e, s, t, f, i, e, l, d, s, t, a, t, e}. (a) How many different words (they do not have to make sense) can you spell with the letters in A? (b) Is your answer from above the same as the cardinality of the powerset of A, i.e. of P(A)? (c) What is |A|?arrow_forward
- what can the answer be pls helparrow_forward5. How many numbers can you make out of the digits 1, 2, 3, 4, 6 if the rule is that every digit has to be larger than the digit preceding it? For example 124 is ok, 122 is not ok. Every digit can be used only once, but you do not have to use every digit. A tree might help.arrow_forward5 plsarrow_forward
- 8 pls show workarrow_forward7 pls show workarrow_forwardThe director of admissions at Kinzua University in Nova Scotia estimated the distribution of student admissions for the fall semester on the basis of past experience. Admissions Probability 1,100 0.5 1,400 0.4 1,300 0.1 Click here for the Excel Data File Required: What is the expected number of admissions for the fall semester? Compute the variance and the standard deviation of the number of admissions. Note: Round your standard deviation to 2 decimal places.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
College Algebra (MindTap Course List)
Algebra
ISBN:9781305652231
Author:R. David Gustafson, Jeff Hughes
Publisher:Cengage Learning
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Big Ideas Math A Bridge To Success Algebra 1: Stu...
Algebra
ISBN:9781680331141
Author:HOUGHTON MIFFLIN HARCOURT
Publisher:Houghton Mifflin Harcourt
Finite Math: Markov Chain Example - The Gambler's Ruin; Author: Brandon Foltz;https://www.youtube.com/watch?v=afIhgiHVnj0;License: Standard YouTube License, CC-BY
Introduction: MARKOV PROCESS And MARKOV CHAINS // Short Lecture // Linear Algebra; Author: AfterMath;https://www.youtube.com/watch?v=qK-PUTuUSpw;License: Standard Youtube License
Stochastic process and Markov Chain Model | Transition Probability Matrix (TPM); Author: Dr. Harish Garg;https://www.youtube.com/watch?v=sb4jo4P4ZLI;License: Standard YouTube License, CC-BY