Chapter 11, Problem 15CQ

a.

To determine

Find the point estimates $b_0\text{ and }{b}_1$.

Answer to Problem 15CQ

The intercept $b_0$ is 13.

The slope $b_1$ is 1.06.

Explanation of Solution

Calculation:

The given information is that the sample data consists of 8 values for x and y.

Slope or $b_1$:

$b_1=r\frac{s_y}{s_x}$

where,

r represents the correlation coefficient between x and y.

$s_y$ represents the standard deviation of y.

$s_x$ represents the standard deviation of x.

Software procedure:

Step-by-step procedure to find the mean, standard deviation for x and y values using MINITAB is given below:

• • Choose Stat > Basic Statistics > Display Descriptive Statistics.
• • In Variables enter the columns of y and x.
• • Choose Options Statistics, select Mean and Standard deviation.
• • Click OK.

Output obtained from MINITAB is given below:

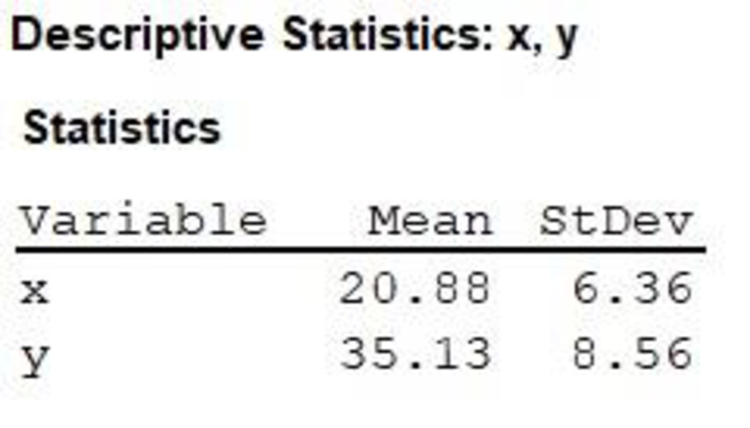
Correlation:

$r=\frac{1}{n-1}{\displaystyle \sum \left(\frac{x-\overline{x}}{s_x}\right)\left(\frac{y-\overline{y}}{s_y}\right)}$

Where,

$\overline{y}$ represents the mean of y values.

$\overline{x}$ represents the mean of x values.

$s_x$ represents the standard deviation of x.

$s_y$ represents the standard deviation of y.

n represents the sample size.

The table shows the calculation of correlation:

x	y	$x-\overline{x}$	$y-\overline{y}$	$\frac{x-\overline{x}}{s_x}$	$\frac{y-\overline{y}}{s_y}$	$\left(\frac{x-\overline{x}}{s_x}\right)\left(\frac{y-\overline{y}}{s_y}\right)$
25	40	4.12	4.87	0.6478	0.56893	0.36855
13	20	–7.88	–15.13	–1.239	–1.7675	2.18995
16	33	–4.88	–2.13	–0.7673	–0.2488	0.19093
19	30	–1.88	–5.13	–0.2956	–0.5993	0.17715
29	50	8.12	14.87	1.27673	1.73715	2.21787
19	37	–1.88	1.87	–0.2956	0.21846	–0.0646
16	34	–4.88	–1.13	–0.7673	–0.132	0.10129
30	37	9.12	1.87	1.43396	0.21846	0.31326
Total						5.4944

Thus, the correlation is

$\begin{array}{c}r=\frac{5.4944}{8-1}\\ =\frac{5.4944}{7}\\ =0.7850\end{array}$

$b_1=r\frac{s_y}{s_x}$

Substitute r as 0.7850, $s_y$ as 8.56 and $s_x$ as 6.36.

$\begin{array}{c}{b}_1=0.7850\left(\frac{8.56}{6.36}\right)\\ =0.7850\left(1.346\right)\\ =1.06\end{array}$

Thus, the slope $b_1$ is 1.06.

Intercept of $b_0$:

$b_0=\overline{y}-b_1\overline{x}$

$\overline{y}$ represents the mean of y values.

$\overline{x}$ represents the mean of x values.

$b_1$ represents the slope coefficient.

Substitute $\overline{y}$ as 35.13, $\overline{x}$ as 20.88 and $b_1$ as 1.06.

$\begin{array}{c}{b}_0=35.13-1.06\left(20.88\right)\\ =35.13-22.13\\ =13\end{array}$

Thus, the intercept $b_0$ is 13.

b.

To determine

Construct the 95% confidence interval for ${\beta }_1$.

Answer to Problem 15CQ

The 95% confidence interval for ${\beta }_1$ is $\left(0.23,1.89\right)$

Explanation of Solution

Calculation:

The confidence interval for ${\beta }_1$ is calculated by using the formula:

$\text{Confidence interval}=b_1\pm t_{\frac{\alpha }{2}}\cdot s_{b_1}$

Where,

$b_1$ represents the estimated slope coefficient.

$t_{\frac{\alpha }{2}}\cdot s_{b_1}$ represents the margin of error.

Critical value:

Software procedure:

Step-by-step procedure to find the critical value using MINITAB is given below:

• • Choose Graph > Probability Distribution Plot choose View Probability > OK.
• • From Distribution, choose ‘t’ distribution.
• • In Degrees of freedom, enter 6.
• • Click the Shaded Area tab.
• • Choose Probability and Two tail for the region of the curve to shade.
• • Enter the Probability value as 0.05.
• • Click OK.

Output obtained from MINITAB is given below:

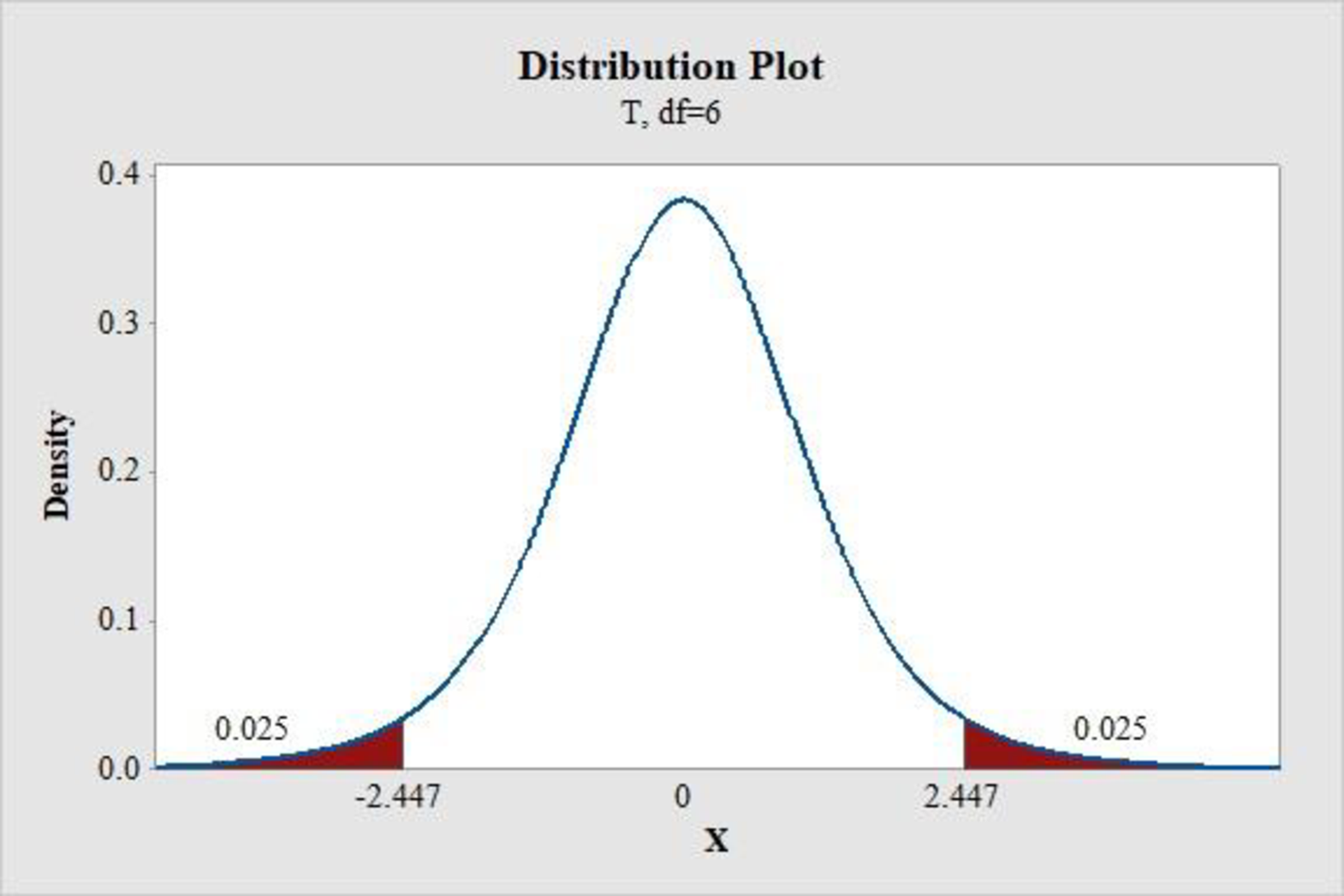
Thus, the critical value for a 95% confidence interval of ${\beta }_1$ is 2.447.

The standard error of $b_1$ is calculated by using the formula:

$s_b=\frac{s_e}{\sqrt{{\displaystyle \sum {\left(x-\overline{x}\right)}^2}}}$

Where,

$s_e$ represents the residual standard deviation.

${\displaystyle \sum {\left(x-\overline{x}\right)}^2}$ represents the sum of squares due to x.

Calculation of residual standard deviation:

Use the estimated regression equation to find the predicted value of y for each value of x and the estimated regression equation is $\widehat{y}=13+1.06x$.

The table below shows the calculation of residual standard deviation:

x	y	$\widehat{y}$	$y-\widehat{y}$	${\left(y-\widehat{y}\right)}^2$
25	40	39.5	0.5	0.25
13	20	26.78	–6.78	45.9684
16	33	29.96	3.04	9.2416
19	30	33.14	-3.14	9.8596
29	50	43.74	6.26	39.1876
19	37	33.14	3.86	14.8996
16	34	29.96	4.04	16.3216
30	37	44.8	–7.8	60.84
Total				196.57

Substitute ${{\displaystyle \sum \left(y-\widehat{y}\right)}}^2$ as 196.57 and n as 8.

$\begin{array}{c}{s}_e=\sqrt{\frac{196.57}{8-2}}\\ =\sqrt{\frac{196.57}{6}}\\ =\sqrt{32.76}\\ =5.724\end{array}$

Thus, the residual standard deviation $s_e$ is 5.724.

The table shows the calculation of sum of squares for x.

x	$x-\overline{x}$	${\left(x-\overline{x}\right)}^2$
25	4.12	16.9744
13	–7.88	62.0944
16	–4.88	23.8144
19	–1.88	3.5344
29	8.12	65.9344
19	–1.88	3.5344
16	–4.88	23.8144
30	9.12	83.1744
Total		282.88

Substitute ${\displaystyle \sum {\left(x-\overline{x}\right)}^2}$ as 282.88 and $s_e$ as 5.724.

$\begin{array}{c}{s}_b=\frac{5.724}{\sqrt{282.88}}\\ =\frac{5.724}{16.82}\\ =0.34\end{array}$

Thus, the standard error of $b_1$ is 0.34.

95% confidence interval for $b_1$ is given below:

$\begin{array}{c}\text{Confidence interval}=1.06\pm \left(2.447\right)\left(0.34\right)\\ =1.06\pm 0.83\\ =0.23,1.89\end{array}$

Thus, the 95% confidence interval for ${\beta }_1$ is $\left(0.23,1.89\right)$

c.

To determine

Test the significance of ${\beta }_1$ using 1% level of significance.

Answer to Problem 15CQ

There is no support of evidence to conclude that there is a linear relationship between x and y at 1% level of significance.

Explanation of Solution

Calculation:

The hypotheses used for testing the significance is given below:

Null hypothesis:

$H_0:{\beta }_1=0$

That is, there is no linear relationship between x and y.

Alternate hypothesis:

$H_1:{\beta }_1\ne 0$

That is, there is a linear relationship between x and y.

Test statistic:

$t=\frac{{\widehat{\beta }}_1-{\beta }_1}{s_{b_1}}$

Where,

${\widehat{\beta }}_1$ represents the estimated slope coefficient.

${\beta }_1$ represents the hypothesized value of slope coefficient.

$s_{b_1}$ represents the standard error of slope coefficient.

Substitute ${\widehat{\beta }}_1$ as 1.06, ${\beta }_1$ as 0 and $s_{b_1}$ as 0.34.

$\begin{array}{c}t=\frac{1.06-0}{0.34}\\ =\frac{1.06}{0.34}\\ =3.12\end{array}$

Critical value:

Software procedure:

Step-by-step procedure to find the critical value using MINITAB is given below:

• • Choose Graph > Probability Distribution Plot choose View Probability > OK.
• • From Distribution, choose ‘t’ distribution.
• • In Degrees of freedom, enter 6.
• • Click the Shaded Area tab.
• • Choose Probability and Two tail for the region of the curve to shade.
• • Enter the Probability value as 0.01.
• • Click OK.

Output obtained from MINITAB is given below:

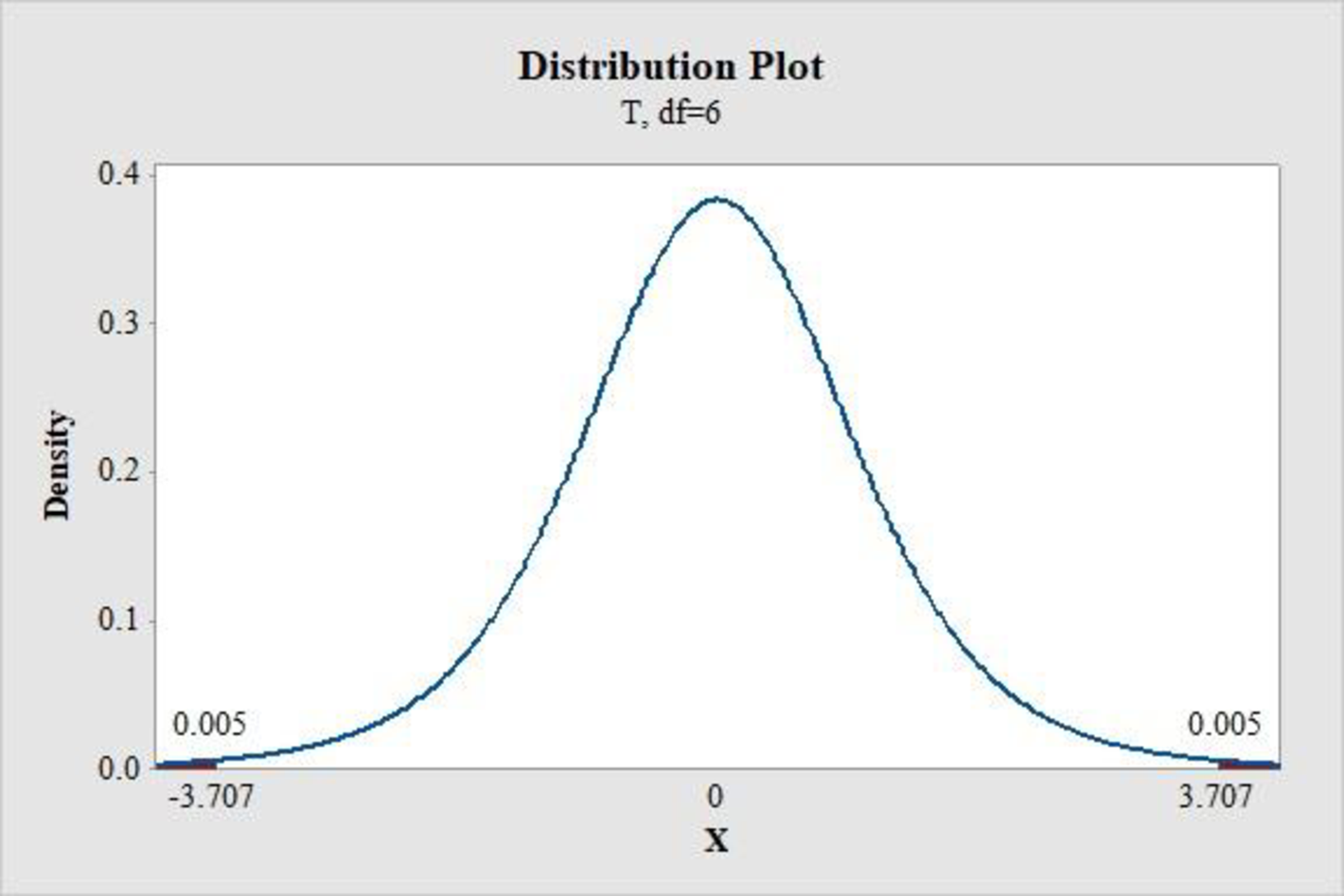
Thus, the critical value for a 99% confidence interval of ${\beta }_1$ is 3.707.

Decision Rule:

Reject the null hypothesis when the test statistic value is greater than the critical value for a given level of significance. Otherwise, do not reject the null hypothesis.

Conclusion:

The test statistic value is 3.12 and the critical value is 3.707.

The test statistic value is lesser than the critical value.

That is, $3.12\left(=\text{test statistic}\right)<3.707\left(=\text{critical value}\right)$

Thus, the null hypothesis is not rejected.

Hence, there is no support of evidence to conclude that there is a linear relationship between x and y at 1% level of significance.

d.

To determine

Construct the 95% confidence interval for the mean response for the given value of x.

Answer to Problem 15CQ

The 95% confidence interval for the mean response for the given value of x is $\left(29.1947,39.2047\right)$.

Explanation of Solution

Calculation:

The given value of x is 20.

Software procedure:

Step-by-step procedure to construct the 95% confidence interval for the mean response for the given value of x is given below:

• • Choose Stat > Regression > Regression.
• • In Response, enter the column containing the y.
• • In Predictors, enter the columns containing the x.
• • Click OK.
• • Choose Stat > Regression > Regression>Predict.
• • Choose Enter the individual values.
• • Enter the x as 20.
• • Click OK.

Output obtained from MINITAB is given below:

Interpretation:

Thus, the 95% confidence interval for the mean response for the given value of x is $\left(29.1947,39.2047\right)$.

e.

To determine

Construct the 95% prediction interval for the individual response for the given value of x.

Answer to Problem 15CQ

The 95% prediction interval for the individual response for the given value of x is $\left(19.3268,49.0726\right)$.

Explanation of Solution

The given value of x is 20.

From the MINITAB output obtained in the previous part (d) it can be observed that the 95% prediction interval for the individual response for the given value of x is $\left(19.3268,49.0726\right)$.