Chapter 11, Problem 11.34P

Interpretation Introduction

Interpretation:

An instantaneous $90\text{ wt}\%$ sulfuric acid rate as a function of time is to be determined for the given mixing process. Also, a plot of this rate vs time needs to be drawn.

Concept Introduction:

For mixing process, equation for enthalpy is:

  $\Delta H=H^E$ .......(1)

In terms of a third-degree polynomial equation in $x_1$, $H^E$ can be expressed as:

  $\frac{H^E}{x_1{x}_2}=a+b{x}_1+c{x}_1^2+d{x}_1^3$ .......(2)

Here, $a,b,c,\text{ and }d$ are the constants and can be found by polynomial regression using data for $H^E\text{ and }{x}_1$ .

For a binary system, equation $10.15$ to be used is:

  ${\overline{M}}_1=M+x_2\frac{dM}{d{x}_1}$ .......(3)

For a binary system, equation $10.16$ to be used is:

  ${\overline{M}}_2=M-x_1\frac{dM}{d{x}_1}$ .......(4)

Answer to Problem 11.34P

The rate of addition of sulfuric acid to the tank is defined as:

  $r=\frac{d\left(m\left(x_1\right)\right)}{dT\left(x_1\right)}=\frac{-84.72}{\Delta H-H_3}$

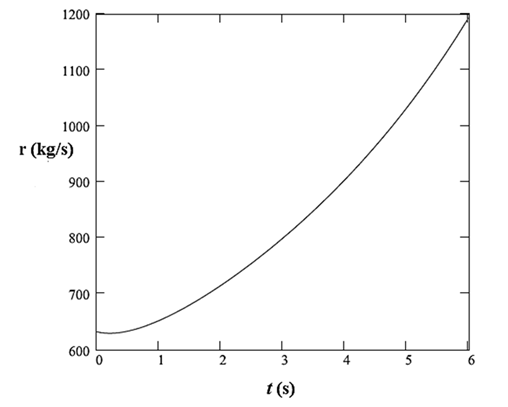
The plot of this rate with time is:

Explanation of Solution

Given information:

At ${25}^{\circ }\text{C}$, a $90{\text{ wt\% H}}_2{\text{SO}}_4$ solution is being added to a tank containing $4000\text{ kg}$ of pure water also at ${25}^{\circ }\text{C}$ over the period of $6\text{ hours}$ . In the tank, the final concentration of the acid is $50\text{ wt\%}$ and a constant temperature of ${25}^{\circ }\text{C}$ is maintained in the tank by continuously cooling the contents of the tank. The cooling system is designed for a constant rate of heat transfer which requires the addition of acid to pure water at variable rate.

The data which may fit to the cubic equation of $H^E/x_1{x}_2$ as a function of $x_1$ for ${\text{H}}_2{\text{SO}}_4{\text{/H}}_2\text{O}$ system as given in the previous problem is:

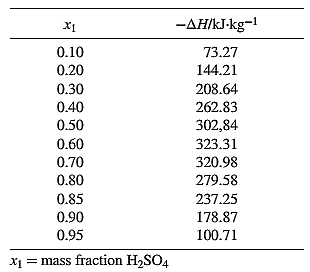
From the equation (1), the given heat of mixing for the values of $x_1$ is same as excess enthalpy. Thus,

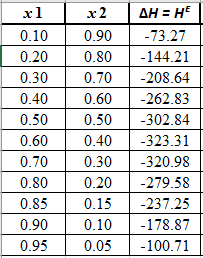
Now, calculate the value of $H^E/x_1{x}_2$ and plot the graph of $H^E/x_1{x}_2$ and use the regression tool of excel and fit the curve into the third-degree polynomial and compare the equation with the form of equation (2) as:

  $\frac{H^E}{x_1{x}_2}=-735.28-824.52(x_1)+195.2{(x_1)}^2-914.58{(x_1)}^3$ .......(5)

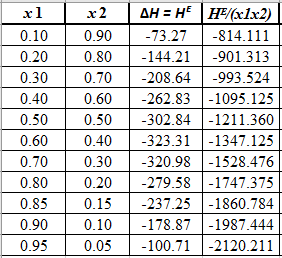
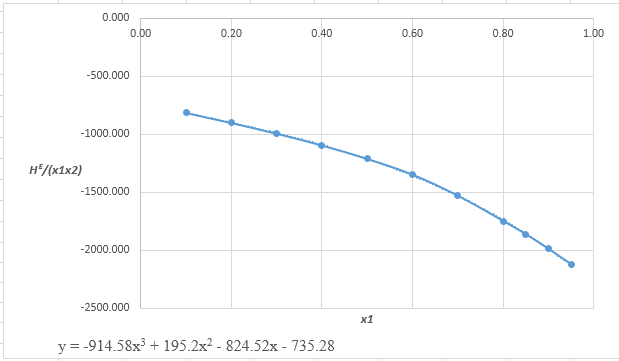
Rewrite the preceding equation in terms of $H^E$ as:

  $H^E=-735.28x_1-89.24{(x_1)}^2+1019.72{(x_1)}^3-1109.78{(x_1)}^4+914.58{(x_1)}^5$

Now, calculate the derivative this equation as:

  $\frac{d{H}^E}{d{x}_1}=-735.28-178.48(x_1)+3059.16{(x_1)}^2-4439.12{(x_1)}^3+4572.9{(x_1)}^4$ .......(6)

Now, calculate the value of ${\overline{H}}_1^E,\text{ and }{\overline{H}}_2^E$ using the equations (3) and (4) as:

  $\begin{array}{c}{\overline{H}}_1^E=H^E+x_2\frac{d{H}^E}{d{x}_1}\\ =\left(\begin{array}{l}-735.28x_1-89.24{(}^{x_1}+1019.72{(}^{x_1}-1109.78{(}^{x_1}+914.58{(}^{x_1}+\\ \left(1-x_1\right)\left(-735.28-178.48(x_1)+3059.16{(x_1)}^2-4439.12{(x_1)}^3+4572.9{(x_1)}^4\right)\end{array}\right)\\ =-735.28-178.48(x_1)+3148.4{(}^{x_1}-6478.56{(}^{x_1}+7902.24{(}^{x_1}-3658.32{(}^{x_1}\\ {\overline{H}}_2^E=H^E-x_1\frac{d{H}^E}{d{x}_1}\\ =\left(\begin{array}{l}-735.28x_1-89.24{(}^{x_1}+1019.72{(}^{x_1}-1109.78{(}^{x_1}+914.58{(}^{x_1}+\\ \left(x_1\right)\left(-735.28-178.48(x_1)+3059.16{(x_1)}^2-4439.12{(x_1)}^3+4572.9{(x_1)}^4\right)\end{array}\right)\\ =-1470.56x_1-267.72{(}^{x_1}+4078.88{(}^{x_1}-5548.9{(}^{x_1}+5487.48{(}^{x_1}\end{array}$

Now, at an instantaneous time $\text{'}t\text{'}$, let:

  $x_1$ be the mass fraction of ${\text{H}}_2{\text{SO}}_4$ in the tank.

  $\text{'}m\text{'}$ be the total of $90\%{\text{ H}}_2{\text{SO}}_4$ added up to time $\text{'}t\text{'}$ .

  $\text{'}H\text{'}$ be the enthalpy of ${\text{H}}_2{\text{SO}}_4$ solution in the tank at ${25}^{\circ }\text{C}$ .

  $\text{'}{H}_1\text{'}$ be the enthalpy of pure ${\text{H}}_2{\text{SO}}_4$ at ${25}^{\circ }\text{C}$ .

  $\text{'}{H}_2\text{'}$ be the enthalpy of pure ${\text{H}}_2\text{O}$ at ${25}^{\circ }\text{C}$ .

  $\text{'}{H}_3\text{'}$ be the enthalpy of ${\text{90 wt\% H}}_2{\text{SO}}_4$ at ${25}^{\circ }\text{C}$ .

Apply material balance on the given process as:

  $x_1\left(4000+m\right)=0.9m$

Solve this equation for $m$ as:

  $m=\frac{4000x_1}{0.9-x_1}$ .......(7)

Now, apply energy balance on the given process and take $Q$ as the heat transferred.

  $\begin{array}{c}Q=\Delta H^t\\ =\left(4000+m\right)H-4000H_2-m{H}_3\end{array}$

Since, the temperature for the given process is taken as constant at ${25}^{\circ }\text{C}$, we set $H_1=H_2=0$ and the equation for $\Delta H$ becomes:

  $\begin{array}{c}\Delta H=H-x_1{H}_1-x_2{H}_2\\ \Delta H=H\end{array}$

Using this relation, the energy balance equation now becomes:

  $Q=\left(4000+m\right)\Delta H-m{H}_3$ .......(8)

For the overall process, the given data are:

  $\begin{array}{c}t=6\text{ h}=21600\text{ s}\\ x_1=0.5\end{array}$

At this $x_1$, the values of $H_3\text{ and }\Delta H$ from the given data table are:

  $\begin{array}{c}{H}_3=-178.737\text{ kJ/kg}\\ \Delta H=-302.84\text{ kJ/kg}\end{array}$

Using equation (7) and (8) and the values required, the value of $m\text{ and }Q$ are:

  $\begin{array}{c}m\left(x_1=0.5\right)=5000\text{ kg}\\ Q\left(x_1=0.5\right)=-1.83\times {10}^6\text{ kJ}\end{array}$

Rewrite equations (7) and (8) as a function of $x_1$ as:

  $\begin{array}{c}m\left(x_1\right)=\frac{4000x_1}{0.9-x_1}\\ Q\left(x_1\right)=\left(4000+\left(m\left(x_1\right)\right)\right)\cdot \Delta H-\left(m\left(x_1\right)\right)\cdot H_3\end{array}$

It is given that the rate of heat transfer $\left(q\right)$ is constant throughout the process, so:

  $\begin{array}{c}q=\frac{Q\left(x_1\right)}{t}\\ =\frac{-1.83\times {10}^6\text{ kJ}}{21600\text{ s}}\\ =-84.72\text{ kJ/s}\end{array}$

Now, the equation for time as a function of $x_1$ as:

  $\begin{array}{l}T\left(x_1\right)=\frac{Q\left(x_1\right)}{q}\\ T\left(x_1\right)=\frac{\left(4000+\left(m\left(x_1\right)\right)\right)\cdot \Delta H-\left(m\left(x_1\right)\right)\cdot H_3}{-84.72}\end{array}$

Take derivative of $m\left(x_1\right)$ with respect to $T\left(x_1\right)$ as:

  $\begin{array}{c}dT\left(x_1\right)=\frac{1}{-84.72}\left(\Delta H\cdot d\left(m\left(x_1\right)\right)-H_3\cdot d\left(m\left(x_1\right)\right)\right)\\ dT\left(x_1\right)=\frac{1}{-84.72}\left(\Delta H-H_3\right)d\left(m\left(x_1\right)\right)\\ \frac{d\left(m\left(x_1\right)\right)}{dT\left(x_1\right)}=\frac{-84.72}{\Delta H-H_3}\end{array}$

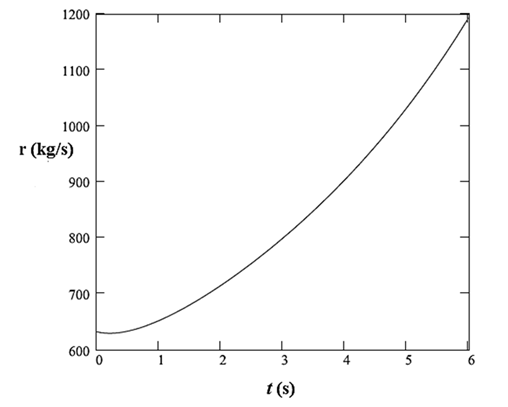
Therefore, the rate of addition of sulfuric acid to the tank is defined as:

  $r=\frac{d\left(m\left(x_1\right)\right)}{dT\left(x_1\right)}=\frac{-84.72}{\Delta H-H_3}$ .......(9)

At $x_1$ mass fraction of acid present in the tank at the instant $\text{'}t\text{'}$, the partial enthalpies of ${\text{H}}_2{\text{SO}}_4{\text{ and H}}_2\text{O}$ are taken as ${\overline{H}}_1^E\text{ and }{\overline{H}}_2^E$ . When $90{\text{ wt\% H}}_2{\text{SO}}_4$ is added to the tank, the change in enthalpy for the process is given by:

  $\Delta H=0.9{\overline{H}}_1^E+0.1{\overline{H}}_2^E$

This change in enthalpy is the required heat per kg of $90\%$ acid to keep the temperature at ${25}^{\circ }\text{C}$ . Use this equation in equation (9) and rewrite the rate equation as:

  $r=\frac{-84.72}{0.9{\overline{H}}_1^E+0.1{\overline{H}}_2^E-H_3}$ .......(10)

Now, using equations (9) and (10), plot the rate $\left(\text{kg/s}\right)$ as a function of time $\left(\text{s}\right)$ as:

Conclusion

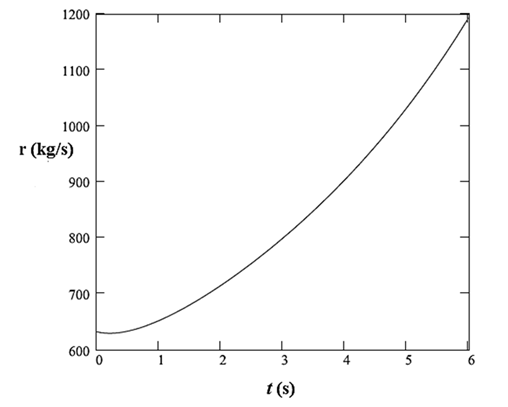
Thus, the rate of addition of sulfuric acid to the tank is defined as:

  $r=\frac{d\left(m\left(x_1\right)\right)}{dT\left(x_1\right)}=\frac{-84.72}{\Delta H-H_3}$

The plot of this rate with time is: