Chapter 10, Problem 64P

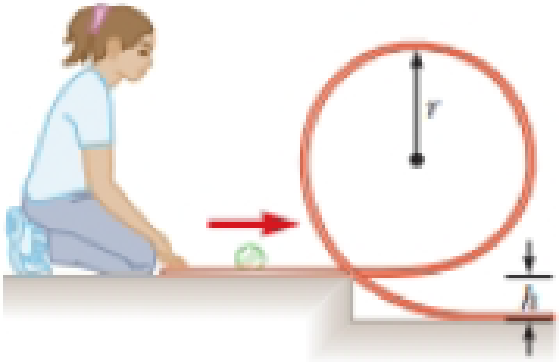
A tennis ball is a hollow sphere with a thin wall. It is set rolling without slipping at 4.03 m/s on a horizontal section of a track as shown in Figure P10.33. It rolls around the inside of a vertical circular loop of radius r = 45.0 cm. As the ball nears the bottom of the loop, the shape of the track deviates from a perfect circle so that the ball leaves the track at a point h = 20.0 cm below the horizontal section. (a) Find the ball’s speed at the top of the loop. (b) Demonstrate that the ball will not fall from the track at the top of the loop. (c) Find the ball’s speed as it leaves the track at the bottom. (d) What If? Suppose that static friction between ball and track were negligible so that the ball slid instead of rolling. Describe the speed of the ball at the top of the loop in this situation. (e) Explain your answer to part (d).

Figure P10.33

To determine

The speed of the ball at the top of the loop.

Answer to Problem 64P

The speed of the ball at the top of the loop is $2.38\text{m}/\text{s}$.

Explanation of Solution

Given information: The radius of the circular loop is $45.0\text{cm}$, the height of the track from the horizontal section is $20.0\text{cm}$ and the initial velocity of the ball is $4.03\text{m}/\text{s}$.

Write the expression for the law of energy conservation between horizontal track and the top of the loop is,

$K_{\text{t1}}+K_{\text{r1}}=K_{\text{t2}}+K_{\text{r2}}+U_{\text{2}}$ (1)

Here,

$K_{\text{t1}}$ is the translational kinetic energy of the ball at horizontal track.

$K_{\text{r1}}$ is the rotational kinetic energy of the ball at horizontal track.

$K_{\text{t2}}$ is the translational kinetic energy of the ball at top point of the loop.

$K_{\text{r2}}$ is the rotational kinetic energy of the ball at top point of the loop.

$U_2$ is the potential energy of the ball at top point of the loop.

Write the expression for the translational kinetic energy of the ball at horizontal track is,

$K_{\text{t1}}=\frac{1}{2}m{v}_{\text{1}}^2$

Here,

$m$ is the mass of the ball.

$v_{\text{1}}$ is the initial linear velocity of the ball.

Write the expression for the rotational kinetic energy of the ball at horizontal track is,

$K_{\text{r1}}=\frac{1}{2}I{\omega }_{\text{1}}^2$ (2)

Here,

$I$ is the moment of inertia of the ball about the center of mass.

${\omega }_{\text{1}}$ is the initial angular speed of the ball.

Write the expression for the moment of inertia of the ball is,

$I=\frac{2}{3}m{r}^2$

Write the expression for the initial angular speed of the ball is,

${\omega }_{\text{1}}=\frac{v_{\text{1}}}{r}$

Substitute $\frac{2}{3}m{r}^2$ for $I$ and $\frac{v_{\text{1}}}{r}$ for ${\omega }_{\text{1}}$ in equation (2).

$\begin{array}{c}{K}_{\text{r1}}=\frac{1}{2}\left(\frac{2}{3}m{r}^2\right){\left(\frac{v_{\text{1}}}{r}\right)}^2\\ =\frac{1}{3}m{v}_{\text{1}}^2\end{array}$

Write the expression for the translational kinetic energy of the ball at top point of the loop is,

$K_{\text{t2}}=\frac{1}{2}m{v}_{\text{2}}^2$

Here,

$v_{\text{2}}$ is the final linear velocity of the ball.

Write the expression for the rotational kinetic energy of the ball at top point of the loop is,

$K_{\text{r2}}=\frac{1}{2}I{\omega }_{\text{2}}^2$ (3)

Here,

${\omega }_{\text{2}}$ is the initial angular speed of the ball.

Write the expression for the moment of inertia of the ball is,

$I=\frac{2}{3}m{r}^2$

Write the expression for the initial angular speed of the ball is,

${\omega }_{\text{2}}=\frac{v_{\text{2}}}{r}$

Substitute $\frac{2}{3}m{r}^2$ for $I$ and $\frac{v_{\text{2}}}{r}$ for ${\omega }_{\text{2}}$ in equation (2).

$\begin{array}{c}{K}_{\text{r2}}=\frac{1}{2}\left(\frac{2}{3}m{r}^2\right){\left(\frac{v_{\text{2}}}{r}\right)}^2\\ =\frac{1}{3}m{v}_{\text{2}}^2\end{array}$

Write the expression for the potential energy of the ball at top point of the loop is,

$U_{\text{2}}=mg(2r)$

Here,

$g$ is the acceleration due to gravity.

Substitute $\frac{1}{2}m{v}_{\text{1}}^2$ for $K_{\text{t1}}$, $\frac{1}{3}m{v}_{\text{1}}^2$ for $K_{\text{r1}}$, $\frac{1}{2}m{v}_{\text{2}}^2$ for $K_{\text{t2}}$, $\frac{1}{3}m{v}_{\text{2}}^2$ for $K_{\text{r2}}$ and $mg(2r)$ for $U_{\text{2}}$ in equation (1).

$\frac{1}{2}m{v}_{\text{1}}^2+\frac{1}{3}m{v}_{\text{1}}^2=\frac{1}{2}m{v}_{\text{2}}^2+\frac{1}{3}m{v}_{\text{2}}^2+mg\left(2r\right)$

Simplify the above equation for $v_2$.

$\begin{array}{c}\frac{5}{6}m{v}_{\text{2}}^2=\frac{5}{6}m{v}_{\text{1}}^2-mg\left(2r\right)\\ v_{\text{2}}=\sqrt{v_{\text{1}}^2-\frac{6}{5}g\left(2r\right)}\end{array}$

Substitute $9.8\text{m}/{\text{s}}^{\text{2}}$ for $g$, $45.0\text{cm}$ for $r$ and $4.03\text{m}/\text{s}$ for $v_{\text{1}}$ in above equation to find $v_{\text{2}}$.

$\begin{array}{c}{v}_{\text{2}}=\sqrt{{\left(4.03\text{m}/\text{s}\right)}^2-\frac{6}{5}\times 9.8\text{m}/{\text{s}}^{\text{2}}\times \left(2\times 45.0\text{cm}\times \frac{{10}^{-2}\text{m}}{1\text{cm}}\right)}\\ =\sqrt{16.24{\text{m}}^{\text{2}}/{\text{s}}^{\text{2}}-10.58{\text{m}}^{\text{2}}/{\text{s}}^{\text{2}}}\\ =2.38\text{m}/\text{s}\end{array}$

Thus, the speed of the ball at the top of the loop is $2.38\text{m}/\text{s}$.

Conclusion:

Therefore, the speed of the ball at the top of the loop is $2.38\text{m}/\text{s}$.

To determine

The reason that the ball will not fall from the track at the top of the loop.

Answer to Problem 64P

The ball will not fall because the value of the centripetal acceleration is more than the acceleration due to gravity at the top point of the circular loop.

Explanation of Solution

Given information: The radius of the circular loop is $45.0\text{cm}$, the height of the track from the horizontal section is $20.0\text{cm}$ and the initial velocity of the ball is $4.03\text{m}/\text{s}$.

Formula to calculate the centripetal acceleration on the ball at the top of the loop is,

$a=\frac{{\left(v_{\text{2}}\right)}^2}{r}$

Here,

$a$ is the centripetal acceleration act on the ball at the top of the loop.

Substitute $2.38\text{m}/\text{s}$ for $v_{\text{2}}$ and $45.0\text{cm}$ for $r$ to find $a$.

$\begin{array}{c}a=\frac{{\left(2.38\text{m}/\text{s}\right)}^2}{45.0\text{cm}\times \frac{{10}^{-2}\text{m}}{1\text{cm}}}\\ =12.58{\text{m}/\text{s}}^2\end{array}$

Thus, the centripetal acceleration act on the ball at the top of the loop is $12.58{\text{m}/\text{s}}^2$.

Since the centripetal acceleration at the top of the loop is more than the acceleration due to gravity that is $\left(a>g\right)$ hence the ball remains on the track at top of the loop and will not fall.

Conclusion:

Therefore, the ball will not fall because the value of the centripetal acceleration is more than the acceleration due to gravity at the top point of the circular loop.

To determine

The speed of the ball as it leaves the track at the bottom.

Answer to Problem 64P

The speed of the ball as it leaves the track at the bottom is $4.31\text{m}/\text{s}$.

Explanation of Solution

Given information: The radius of the circular loop is $45.0\text{cm}$, the height of the track from the horizontal section is $20.0\text{cm}$ and the initial velocity of the ball is $4.03\text{m}/\text{s}$.

Write the expression for the law of energy conservation between horizontal track and the bottom of the loop is,

$K_{\text{t1}}+K_{\text{r1}}=K_{\text{t2}}+K_{\text{r2}}+U_{\text{2}}$ (4)

Here,

$K_{\text{t2}}$ is the translational kinetic energy of the ball at bottom point of the loop.

$K_{\text{r2}}$ is the rotational kinetic energy of the ball at bottom point of the loop.

$U_2$ is the potential energy of the ball at bottom point of the loop.

Write the expression for the translational kinetic energy of the ball at bottom point of the loop is,

$K_{\text{t3}}=\frac{1}{2}m{v}_{\text{3}}^2$

Here,

$v_{\text{3}}$ is the linear velocity of the ball at bottom.

Write the expression for the rotational kinetic energy of the ball at bottom point of the loop is,

$K_{\text{r3}}=\frac{1}{2}I{\omega }_{\text{3}}^2$ (5)

Here,

${\omega }_{\text{3}}$ is the initial angular speed of the ball.

Write the expression for the initial angular speed of the ball is,

${\omega }_{\text{3}}=\frac{v_{\text{3}}}{r}$

Substitute $\frac{2}{3}m{r}^2$ for $I$ and $\frac{v_{\text{3}}}{r}$ for ${\omega }_{\text{3}}$ in equation (5).

$\begin{array}{c}{K}_{\text{r3}}=\frac{1}{2}\left(\frac{2}{3}m{r}^2\right){\left(\frac{v_{\text{3}}}{r}\right)}^2\\ =\frac{1}{3}m{v}_{\text{3}}^2\end{array}$

Write the expression for the potential energy of the ball at top point of the loop is,

$U_{\text{3}}=-mgh$

Here,

$g$ is the acceleration due to gravity.

$h$ is the height of the bottom track from the horizontal section.

Substitute $\frac{1}{2}m{v}_{\text{1}}^2$ for $K_{\text{t1}}$, $\frac{1}{3}m{v}_{\text{1}}^2$ for $K_{\text{r1}}$, $\frac{1}{2}m{v}_{\text{3}}^2$ for $K_{\text{t3}}$, $\frac{1}{3}m{v}_{\text{3}}^2$ for $K_{\text{r3}}$ and $-mgh$ for $U_{\text{3}}$ in equation (4).

$\frac{1}{2}m{v}_{\text{1}}^2+\frac{1}{3}m{v}_{\text{1}}^2=\frac{1}{2}m{v}_{\text{3}}^2+\frac{1}{3}m{v}_{\text{3}}^2-mgh$

Simplify the above equation for $v_{\text{3}}$.

$\begin{array}{c}\frac{5}{6}m{v}_{\text{3}}^2=\frac{5}{6}m{v}_{\text{1}}^2+mgh\\ v_{\text{3}}=\sqrt{v_1^2+\frac{6}{5}gh}\end{array}$

Substitute $9.8\text{m}/{\text{s}}^{\text{2}}$ for $g$, $20.0\text{cm}$ for $h$ and $4.03\text{m}/\text{s}$ for $v_{\text{1}}$ in above equation to find $v_{\text{3}}$.

$\begin{array}{c}{v}_{\text{3}}=\sqrt{{\left(4.03\text{m}/\text{s}\right)}^2+\frac{6}{5}\times 9.8\text{m}/{\text{s}}^{\text{2}}\times 20.0\text{cm}\times \frac{{10}^{-2}\text{m}}{1\text{cm}}}\\ =\sqrt{16.24{\text{m}}^{\text{2}}/{\text{s}}^{\text{2}}+2.35{\text{m}}^{\text{2}}/{\text{s}}^{\text{2}}}\\ =4.31\text{m}/\text{s}\end{array}$

Thus, the speed of the ball as it leaves the track at the bottom is $4.31\text{m}/\text{s}$.

Conclusion:

Therefore, the speed of the ball as it leaves the track at the bottom is $4.31\text{m}/\text{s}$.

To determine

The speed of the ball at the top of the loop if ball slide instead of roll.

Answer to Problem 64P

The speed of the ball at the top of the loop is imaginary.

Explanation of Solution

Given information: The radius of the circular loop is $45.0\text{cm}$, the height of the track from the horizontal section is $20.0\text{cm}$ and the initial velocity of the ball is $4.03\text{m}/\text{s}$.

Write the expression for the law of energy conservation between horizontal track and the top of the loop is,

$K_{\text{t1}}=K_{\text{t2}}\text{'}+U_{\text{2}}\text{'}$ (6)

Here,

$K_{\text{t1}}$ is the translational kinetic energy of the ball at horizontal track.

$K_{\text{t2}}\text{'}$ is the new translational kinetic energy of the ball at top point of the loop.

$U_2\text{'}$ is the new potential energy of the ball at top point of the loop.

Write the expression for the rotational kinetic energy of the ball at horizontal track is,

Write the expression for the new translational kinetic energy of the ball at top point of the loop is,

$K_{\text{t2}}=\frac{1}{2}m{\left(v_2^{\text{'}}\right)}^2$

Here,

$v_2^{\text{'}}$ is the new linear velocity of the ball at the top point.

Write the expression for the new potential energy of the ball at top point of the loop is,

$U_{\text{2}}\text{'}=mg(2r)$

Substitute $\frac{1}{2}m{v}_{\text{1}}^2$ for $K_{\text{t1}}$, $\frac{1}{2}m{\left(v_2^{\text{'}}\right)}^2$ for $K_{\text{t2}}\text{'}$, and $mg(2r)$ for $U_{\text{2}}$ in equation (3).

$\frac{1}{2}m{v}_{\text{1}}^2=\frac{1}{2}m{\left(v_2^{\text{'}}\right)}^2+mg\left(2r\right)$

Simplify the above equation for $v_2$.

$\begin{array}{c}\frac{1}{2}m{\left(v_2^{\text{'}}\right)}^2=\frac{1}{1}m{v}_{\text{1}}^2-mg\left(2r\right)\\ v_2^{\text{'}}=\sqrt{v_{\text{1}}^2-2g\left(2r\right)}\end{array}$

Substitute $9.8\text{m}/{\text{s}}^{\text{2}}$ for $g$, $45.0\text{cm}$ for $r$ and $4.03\text{m}/\text{s}$ for $v_{\text{1}}$ in above equation to find $v_2^{\text{'}}$.

$\begin{array}{c}{v}_2^{\text{'}}=\sqrt{{\left(4.03\text{m}/\text{s}\right)}^2-2\times 9.8\text{m}/{\text{s}}^{\text{2}}\times \left(2\times 45.0\text{cm}\times \frac{{10}^{-2}\text{m}}{1\text{cm}}\right)}\\ =\sqrt{16.24{\text{m}}^{\text{2}}/{\text{s}}^{\text{2}}-17.64{\text{m}}^{\text{2}}/{\text{s}}^{\text{2}}}\\ =\sqrt{-1.4{\text{m}}^2/{\text{s}}^2}\end{array}$

Since the value inside the square root is negative that means the value is imaginary. This condition is impractical.

Thus, the speed of the ball at the top of the loop is imaginary.

Conclusion:

Therefore, the speed of the ball at the top of the loop is imaginary. It can’t be calculated.

To determine

The explanation of the solution of part (d).

Answer to Problem 64P

The ball has not sufficient energy to arrive at top of the circular loop.

Explanation of Solution

Given information: The radius of the circular loop is $45.0\text{cm}$, the height of the track from the horizontal section is $20.0\text{cm}$ and the initial velocity of the ball is $4.03\text{m}/\text{s}$.

The velocity comes out to be imaginary in part (d) that indicates the situation in impossible because as the boll slide instead of rolling, the ball have only translational kinetic energy which is insufficient for the ball to reach the top point on the circular loop.

Thus, the ball did not arrive at the top point of the loop.

Conclusion:

Therefore, the ball has not sufficient energy to arrive at top of the circular loop.