
Concept explainers
(a)
The angular speed of the rod.
(a)
Answer to Problem 73AP
The angular speed of the rod is
Explanation of Solution
For calculation of gravitational energy, rigid body can be modeled as particle at center of mass.
Only conservative forces are acting on the bar, we have conservation of energy of the bar-Earth system. This can be solving by Conservation of energy.
Write the expression for conservation of energy as.
Here,
Substitute
Rearrange the above equation as.
Write the expression for final kinetic energy of the rod as.
Here,
The moment of inertia of rod is
Substitute
Here,
Rearrange the above equation as.
Write the expression for initial potential energy as.
Here,
Conclusion:
Substitute
Simplify the above equation as.
Thus, the angular speed of the rod is
(b)
The magnitude of
(b)
Answer to Problem 73AP
The magnitude of the angular acceleration is
Explanation of Solution
Redraw the figure P10.73 as.
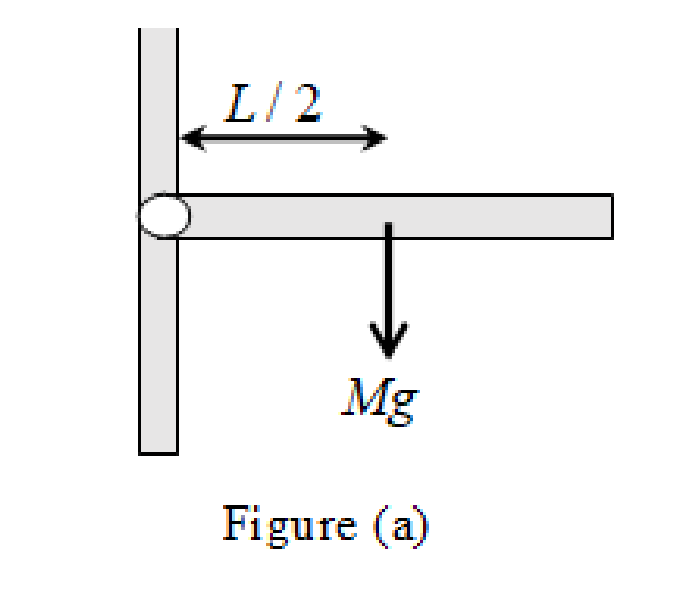
Angular acceleration can be found using torque analysis.
Write the expression for torque of the rod as.
Here,
Write the expression for torque in terms of force and distance as.
Here,
The length is between a center of rotation and the pivot point where a force is applied that means distance for the pivot is
Substitute
Substitute
Rearrange the above expression for angular acceleration as.
Thus, the magnitude of the angular acceleration is
Conclusion:
(c)
The
(c)
Answer to Problem 73AP
The net acceleration for the system is
Explanation of Solution
Redraw the figure P10.73 for calculate the components of the acceleration as
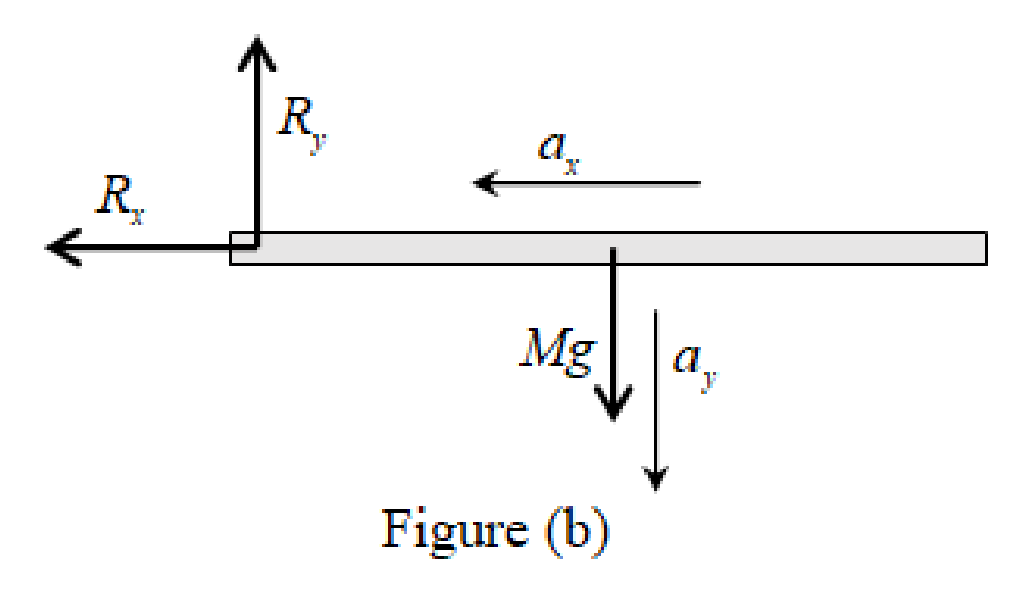
Refer to figure, the horizontal acceleration is directed towards the centre. If an object is free to move due to fixed point. The object will follow the circular path. Since, the horizontal acceleration will act as centripetal acceleration.
Write the expression for horizontal acceleration as.
Here,
Negative sign shows the direction of acceleration towards the negative
Write the expression for centripetal acceleration for the rod as.
Here,
The radius of circular path for this system is defined as the half length of the rod that is
Substitute
Simplify the above equation as.
As the vertical acceleration will be same as tangential acceleration the rod follows the circular path.
Write the expression for vertical acceleration as.
Here,
Write the expression for tangential acceleration as.
Substitute
Simplify the above equation as.
Write the expression for net acceleration for the
Here,
Conclusion:
Substitute
This is centripetal acceleration; it is directed along the negative horizontal.
Substitute
This is centripetal acceleration; it is directed along the negative vertical.
Substitute
Simplify the above equation as.
Thus, the net acceleration for the system is
(d)
The components of the reaction force at the pivot.
(d)
Answer to Problem 73AP
The net force exerts on the rod at the pivot is
Explanation of Solution
The pivot exerts a force
Write the expression for force on the rod as.
Here,
Write the expression for horizontal force as.
Substitute
Rearrange the above equation as.
Write the expression for vertical force as.
Substitute
Simplify the above equation as.
Conclusion:
Substitute
Thus, the net force exerts on the rod at the pivot is
Want to see more full solutions like this?
Chapter 10 Solutions
Physics For Scientists And Engineers With Modern Physics, 9th Edition, The Ohio State University
- Hi Expert in Physics, I have uploaded pictures with respect to some physics equations. Could please name all Greek alphabet and their English name?arrow_forward81 SSM Figure 29-84 shows a cross section of an infinite conducting sheet carrying a current per unit x-length of 2; the current emerges perpendicularly out of the page. (a) Use the Biot-Savart law and symmetry to show that for all points B P P. BD P' Figure 29-84 Problem 81. x P above the sheet and all points P' below it, the magnetic field B is parallel to the sheet and directed as shown. (b) Use Ampere's law to prove that B = ½µλ at all points P and P'.arrow_forwardWhat All equations of Ountum physics?arrow_forward
- Please rewrite the rules of Quantum mechanics?arrow_forwardSuppose there are two transformers between your house and the high-voltage transmission line that distributes the power. In addition, assume your house is the only one using electric power. At a substation the primary of a step-down transformer (turns ratio = 1:23) receives the voltage from the high-voltage transmission line. Because of your usage, a current of 51.1 mA exists in the primary of the transformer. The secondary is connected to the primary of another step-down transformer (turns ratio = 1:36) somewhere near your house, perhaps up on a telephone pole. The secondary of this transformer delivers a 240-V emf to your house. How much power is your house using? Remember that the current and voltage given in this problem are rms values.arrow_forwardThe human eye is most sensitive to light having a frequency of about 5.5 × 1014 Hz, which is in the yellow-green region of the electromagnetic spectrum. How many wavelengths of this light can fit across a distance of 2.2 cm?arrow_forward
- A one-dimensional harmonic oscillator of mass m and angular frequency w is in a heat bath of temperature T. What is the root mean square of the displacement of the oscillator? (In the expressions below k is the Boltzmann constant.) Select one: ○ (KT/mw²)1/2 ○ (KT/mw²)-1/2 ○ kT/w O (KT/mw²) 1/2In(2)arrow_forwardTwo polarizers are placed on top of each other so that their transmission axes coincide. If unpolarized light falls on the system, the transmitted intensity is lo. What is the transmitted intensity if one of the polarizers is rotated by 30 degrees? Select one: ○ 10/4 ○ 0.866 lo ○ 310/4 01/2 10/2arrow_forwardBefore attempting this problem, review Conceptual Example 7. The intensity of the light that reaches the photocell in the drawing is 160 W/m², when 0 = 18°. What would be the intensity reaching the photocell if the analyzer were removed from the setup, everything else remaining the same? Light Photocell Polarizer Insert Analyzerarrow_forward
- The lifetime of a muon in its rest frame is 2.2 microseconds. What is the lifetime of the muon measured in the laboratory frame, where the muon's kinetic energy is 53 MeV? It is known that the rest energy of the muon is 106 MeV. Select one: O 4.4 microseconds O 6.6 microseconds O 3.3 microseconds O 1.1 microsecondsarrow_forwardThe Lagrangian of a particle performing harmonic oscil- lations is written in the form L = ax² - Bx² - yx, where a, and are constants. What is the angular frequency of oscillations? A) √2/a B) √(+2a)/B C) √√Ba D) B/αarrow_forwardThe mean temperature of the Earth is T=287 K. What would the new mean temperature T' be if the mean distance between the Earth and the Sun was increased by 2%? Select one: ○ 293 K O 281 K ○ 273 K 284 Karrow_forward
 Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University





