University Physics Volume 1
18th Edition
ISBN: 9781938168277
Author: William Moebs, Samuel J. Ling, Jeff Sanny
Publisher: OpenStax - Rice University
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 1, Problem 15P
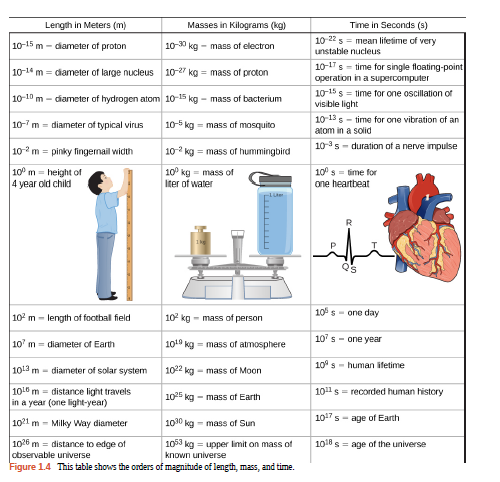
Use the orders of magnitude you found in the previous problem to answer the following questions to within an order of magnitude. (a) How many electrons would it take to equal the mass of a proton? (b) How many Earths would it take to equal the mass of the Sun? (c) How many Earth-Moon distances would it take to cover the distance from Earth to the Sun? (d) How many Moon atmospheres would it take to equal the mass of Earth’s atmosphere? (e) How many moons would it take to equal the mass of Earth? (f) How many protons would it take to equal the mass of the Sun?
For the remaining questions, you need to use Figure 1.4 to obtain the necessary orders of magnitude of lengths, masses, and times.
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
Example
Two charges, one with +10 μC of charge, and
another with - 7.0 μC of charge are placed in
line with each other and held at a fixed distance
of 0.45 m. Where can you put a 3rd charge of +5
μC, so that the net force on the 3rd charge is
zero?
*
Coulomb's Law Example
Three charges are positioned as seen below. Charge
1 is +2.0 μC and charge 2 is +8.0μC, and charge 3 is -
6.0MC.
What is the magnitude and the direction of the force
on charge 2 due to charges 1 and 3?
93
kq92
F
==
2
r13 = 0.090m
91
r12 = 0.12m
92
Coulomb's Constant: k = 8.99x10+9 Nm²/C²
✓
Make sure to draw a Free Body Diagram as well
Chapter 1 Solutions
University Physics Volume 1
Ch. 1 - Check Your Understanding Restate 4.79105kg using a...Ch. 1 - Check Your Understanding Light navels about 9 Pm...Ch. 1 - Check Your Understanding We know horn Figure 1.4...Ch. 1 - Check Your Understanding Given that 1 lb (pound)...Ch. 1 - Check Your Understanding Suppose we want the...Ch. 1 - Check Your Understanding Is the equation...Ch. 1 - Check Your Understanding Figure 1.4 says the mass...Ch. 1 - Check Your Understanding A high school track coach...Ch. 1 - The Scope and Scale of Physics What is physics?Ch. 1 - The Scope and Scale of Physics Some have described...
Ch. 1 - The Scope and Scale of Physics If two different...Ch. 1 - The Scope and Scale of Physics What determines the...Ch. 1 - The Scope and Scale of Physics Certain criteria...Ch. 1 - The Scope and Scale of Physics Can the validity of...Ch. 1 - Units and Standards Identify some advantages of...Ch. 1 - Units and Standards What are the SI base units of...Ch. 1 - Units and Standards What is the difference between...Ch. 1 - For each of the following scenarios, refer to...Ch. 1 - Significant Figures (a) What is the relationship...Ch. 1 - Solving Problems in Physics What information do...Ch. 1 - Solving Problems in Physics What should you do...Ch. 1 - The Scope and Scale of Physics Find the order of...Ch. 1 - Use the orders of magnitude you found in the...Ch. 1 - Roughly how many heartbeats are there in a...Ch. 1 - A generation is about one-third of a lifetime....Ch. 1 - Roughly how many times longer than the mean life...Ch. 1 - Calculate the approximate number of atoms in a...Ch. 1 - (a) Calculate the number of cells in a hummingbird...Ch. 1 - Assuming one nerve impulse must end before another...Ch. 1 - About how many floating-point operations can a...Ch. 1 - Roughly how many floating-point operations can a...Ch. 1 - The following times are given using metric...Ch. 1 - The following times are given in seconds. Use...Ch. 1 - The following lengths are given using metric...Ch. 1 - The following lengths are given in meters. Use...Ch. 1 - The following masses are written using metric...Ch. 1 - The following masses are given in kilograms. Use...Ch. 1 - The volume of Earth is on the order of 1021m3 ....Ch. 1 - The speed limit on some interstate highways is...Ch. 1 - A car is traveling at a speed of 33 m/s. (a) What...Ch. 1 - In SI units, speeds are measured in meters per...Ch. 1 - American football is played on a 100-yd-long...Ch. 1 - Soccer fields vary in size. A large soccer field...Ch. 1 - What is the height in meters of a person who is...Ch. 1 - Mount Everest, at 29,028ft , is the tallest...Ch. 1 - The speed of sound is measured to be 342 m/s on a...Ch. 1 - Tectonic plates are large segments of Earth’s mist...Ch. 1 - The average distance between Earth and the Sun is...Ch. 1 - The density of nuclear matter is about 1018kg/m3 ....Ch. 1 - The density of aluminum Is 2.7g/cm3 . What is the...Ch. 1 - A commonly used turn of mass in the English system...Ch. 1 - A furlong is 220 yd. A fortnight is 2 weeks....Ch. 1 - It takes 2 radians (rad) to get around a circle,...Ch. 1 - Light travels a distance of about 3108m/s . A...Ch. 1 - A light-nanosecond is the distance light travels...Ch. 1 - An electron has a mass of 9.1110-31kg . A proton...Ch. 1 - A fluid ounce is about 30mL. What is the voIume of...Ch. 1 - A student is trying to remember some formulas from...Ch. 1 - Consider the physical quantities s,v,a, and t with...Ch. 1 - Consider the physical quantities m,s,v,a, and t...Ch. 1 - Suppose quantity s is a length and quantity t is a...Ch. 1 - Suppose [V]=L3,[]=ML3, and [t]=T . (a) What is the...Ch. 1 - The arc length formula says the length sof arc...Ch. 1 - Estimates and Fermi Calculations Assuming the...Ch. 1 - Assuming the human body is primarily made of...Ch. 1 - Estimate the mass of air in a classroom.Ch. 1 - Estimate the number of molecules that make up...Ch. 1 - Estimate the surface area of a person.Ch. 1 - Roughly how many solar systems would it take to...Ch. 1 - (a) Estimate the density of the Moon. (b) Estimate...Ch. 1 - The average density of the Sun is on the order...Ch. 1 - Estimate the mass of a virus.Ch. 1 - A floating-point operation is a single arithmetic...Ch. 1 - Consider the equation 4000/400=10.0 . Assuming the...Ch. 1 - Suppose your bathroom scale reads your mass as 65...Ch. 1 - A good-quality measuring tape can be off by 0.50cm...Ch. 1 - An infant’s pulse rate is measured to be 1305...Ch. 1 - (a) Suppose that a person has an average heart...Ch. 1 - A can contains 375 mL of soda. How much is left...Ch. 1 - State how many significant figures are proper In...Ch. 1 - (a) How many significant figures are in the...Ch. 1 - (a) If your speedometer has an uncertainty of 2.0...Ch. 1 - (a) A person’s blood pressure is measured to be...Ch. 1 - A person measures his or her heart rate by...Ch. 1 - What is the area of a circle 3.102 cm in diameter?Ch. 1 - Determine the number of significant figures in the...Ch. 1 - Perform the following calculations and express...Ch. 1 - Consider the equation y=mt+b, where the dimension...Ch. 1 - Consider the equation...Ch. 1 - (a) A car speedometer has a 5% uncertainty. What...Ch. 1 - A marathon runner completes a 42.188-km course in...Ch. 1 - The sides of a small rectangular box are measured...Ch. 1 - When nonmetric units we used in the United...Ch. 1 - The length and width of a rectangular room are...Ch. 1 - A car engine moves a piston with a circular...Ch. 1 - The first atomic bomb was detonated on July 16,...Ch. 1 - The purpose of this problem is to show the entire...
Additional Science Textbook Solutions
Find more solutions based on key concepts
Fill in the blanks: The nose is to the mouth. The ankle is to the knee. The ring finger is to the inde...
Human Anatomy & Physiology (2nd Edition)
When Mendel did crosses of true-breeding purple- and white-flowered pea plants, the white-flowered trait disapp...
Campbell Biology (11th Edition)
WHAT IF? Consider two species that diverged while geographically separated but resumed contact before reproduct...
Campbell Biology in Focus (2nd Edition)
Choose the best answer to each of the following. Explain your reasoning. 1.Measurement of how orbital aur vital...
Cosmic Perspective Fundamentals
The number of named species is about ________, but the actual number of species on Earth is estimated to be abo...
Biology: Life on Earth with Physiology (11th Edition)
Whether the 0oC temperature is equal to 0oF temperature or not needs to be explained. Concept Introduction: The...
Living By Chemistry: First Edition Textbook
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- RT = 4.7E-30 18V IT = 2.3E-3A+ 12 38Ω ли 56Ω ли r5 27Ω ли r3 28Ω r4 > 75Ω r6 600 0.343V 75.8A Now figure out how much current in going through the r4 resistor. |4 = unit And then use that current to find the voltage drop across the r resistor. V4 = unitarrow_forward7 Find the volume inside the cone z² = x²+y², above the (x, y) plane, and between the spheres x²+y²+z² = 1 and x² + y²+z² = 4. Hint: use spherical polar coordinates.arrow_forwardганм Two long, straight wires are oriented perpendicular to the page, as shown in the figure(Figure 1). The current in one wire is I₁ = 3.0 A, pointing into the page, and the current in the other wire is 12 4.0 A, pointing out of the page. = Find the magnitude and direction of the net magnetic field at point P. Express your answer using two significant figures. VO ΜΕ ΑΣΦ ? Figure P 5.0 cm 5.0 cm ₁ = 3.0 A 12 = 4.0 A B: μΤ You have already submitted this answer. Enter a new answer. No credit lost. Try again. Submit Previous Answers Request Answer 1 of 1 Part B X Express your answer using two significant figures. ΜΕ ΑΣΦ 0 = 0 ? below the dashed line to the right P You have already submitted this answer. Enter a new answer. No credit lost. Try again.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
An Introduction to Physical SciencePhysicsISBN:9781305079137Author:James Shipman, Jerry D. Wilson, Charles A. Higgins, Omar TorresPublisher:Cengage Learning
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill
AstronomyPhysicsISBN:9781938168284Author:Andrew Fraknoi; David Morrison; Sidney C. WolffPublisher:OpenStax
An Introduction to Physical Science
Physics
ISBN:9781305079137
Author:James Shipman, Jerry D. Wilson, Charles A. Higgins, Omar Torres
Publisher:Cengage Learning
University Physics Volume 1
Physics
ISBN:9781938168277
Author:William Moebs, Samuel J. Ling, Jeff Sanny
Publisher:OpenStax - Rice University
Physics for Scientists and Engineers, Technology ...
Physics
ISBN:9781305116399
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Principles of Physics: A Calculus-Based Text
Physics
ISBN:9781133104261
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Glencoe Physics: Principles and Problems, Student...
Physics
ISBN:9780078807213
Author:Paul W. Zitzewitz
Publisher:Glencoe/McGraw-Hill
Astronomy
Physics
ISBN:9781938168284
Author:Andrew Fraknoi; David Morrison; Sidney C. Wolff
Publisher:OpenStax
Half life | Radioactivity | Physics | FuseSchool; Author: FuseSchool - Global Education;https://www.youtube.com/watch?v=IDkNlU7zKYU;License: Standard YouTube License, CC-BY