
Concept explainers
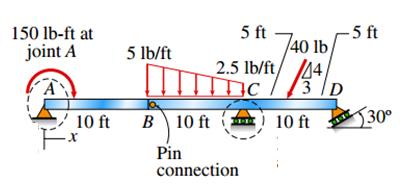
Segments AB and BCD of beam ABCD are pin connected at x = 10 ft. The beam is supported by a pin support at A and roller supports at C and D; the roller at D is rotated by 30* from the x axis (see figure). A trapezoidal distributed load on BC varies in intensity from 5 lb/ft at B to 2.5 lb/ft at C. A concentrated moment is applied at joint A, and a 40-lb inclined load is applied at the mid-span or CD.
(a) Find reactions at supports A, C, and D.
(b) Find the resultant force in the pin connection at B.
(c) Repeat parts (a) and (b) if a rotational spring(kr= 50 ft-lb/radian ) is added at A and the roller at C is removed.
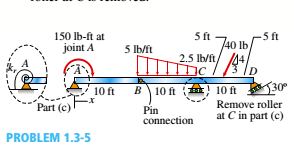
(a)
Determine the reactions at supports A, C, and D in the following figure.
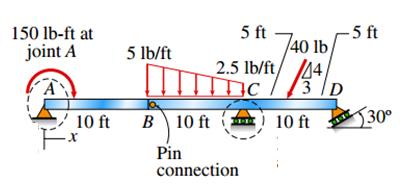
Answer to Problem 1.3.5P
The correct answers are:
Explanation of Solution
Given Information:
The following figure is given for reference.
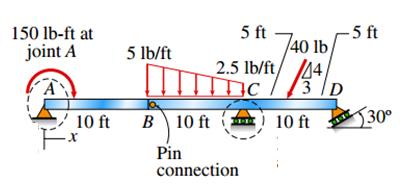
Calculation:
To find support reactions, draw free body diagram as shown below.
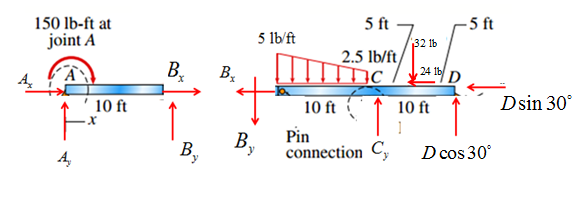
First analyze left hand side of the above figure as shown below.
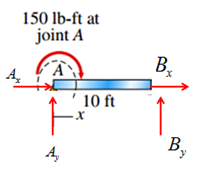
Take equilibrium of moments about point A,
Take equilibrium of forces in
Take equilibrium of forces in
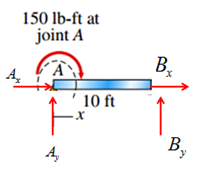
Now, analyze the right hand side of the above figure as shown below,
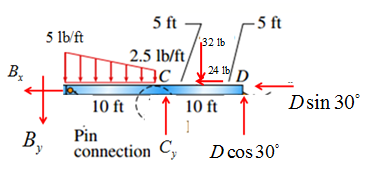
Take equilibrium of moments about point B,
Take equilibrium of forces in
Solve equations and for
Take equilibrium of forces in
Now solve equation
Conclusion:
Thus reactions forces are:
(b)
Determine the resultant force in pin B.
Answer to Problem 1.3.5P
The correct answer is:
Explanation of Solution
Given Information:
The following figure is given for reference.
Calculation:
To find support reactions, draw free body diagram as shown below.
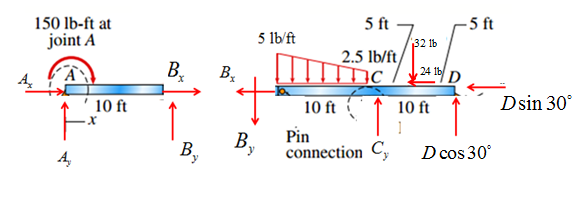
First analyze left hand side of the above figure as shown below.
Take equilibrium of moments about point A,
Take equilibrium of forces in
Now, analyze the right hand side of the above figure as shown below,
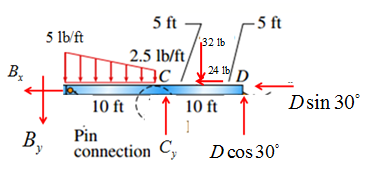
Take equilibrium of moments about point B,
Take equilibrium of forces in
Solve equations and for
Take equilibrium of forces in
Conclusion:
Thus resultant force is:
(c)
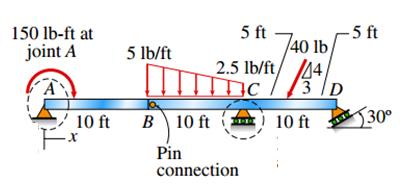
Determine the reactions at supports A, C, and D; and resultant force at B in the following figure.
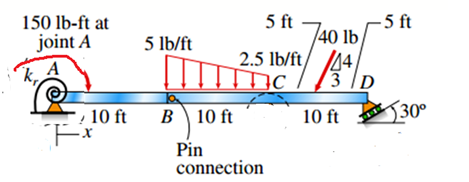
Answer to Problem 1.3.5P
The correct answers are:
Explanation of Solution
Given Information:
The following figure is given for reference.
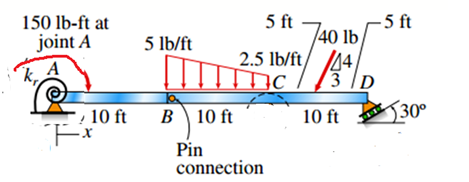
Calculation:
To find support reactions, draw free body diagram as shown below.
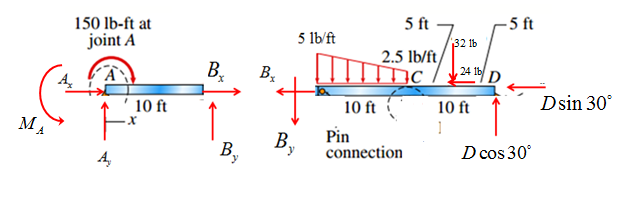
First, analyze the right hand side of the above figure as shown below,
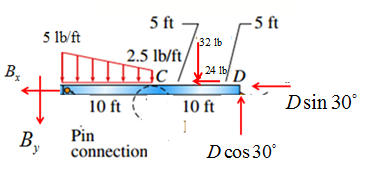
Take equilibrium of moments about point B,
So
Take equilibrium of forces in
Take equilibrium of forces in
Thus resultant force at B is:
Now, analyze left hand side of the above figure as shown below.
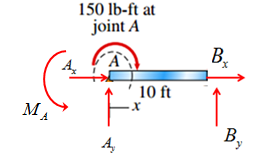
Take equilibrium of moments about point A,
Take equilibrium of forces in
Take equilibrium of forces in
Conclusion:
The correct answers are:
Want to see more full solutions like this?
Chapter 1 Solutions
Bundle: Mechanics Of Materials, Loose-leaf Version, 9th + Mindtap Engineering, 1 Term (6 Months) Printed Access Card
- In cold isostatic pressing, the mold is most typically made of which one of the following: thermosetting polymer tool steel sheet metal textile rubberarrow_forwardThe coefficient of friction between the part and the tool in cold working tends to be: lower higher no different relative to its value in hot workingarrow_forwardThe force F={25i−45j+15k}F={25i−45j+15k} lblb acts at the end A of the pipe assembly shown in (Figure 1). Determine the magnitude of the component F1 which acts along the member AB. Determine the magnitude of the component F2 which acts perpendicular to the AB.arrow_forward
 Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
