Answered: Estimate the mass of Earth Part 2)…

College Physics

11th Edition

ISBN:9781305952300

Author:Raymond A. Serway, Chris Vuille

Publisher:Raymond A. Serway, Chris Vuille

Chapter1: Units, Trigonometry. And Vectors

Section: Chapter Questions

Problem 1CQ: Estimate the order of magnitude of the length, in meters, of each of the following; (a) a mouse, (b)...

See similar textbooks

Related questions

Question

Please refer to the picture for the formulas and constants that have to be used and shown in this problem.

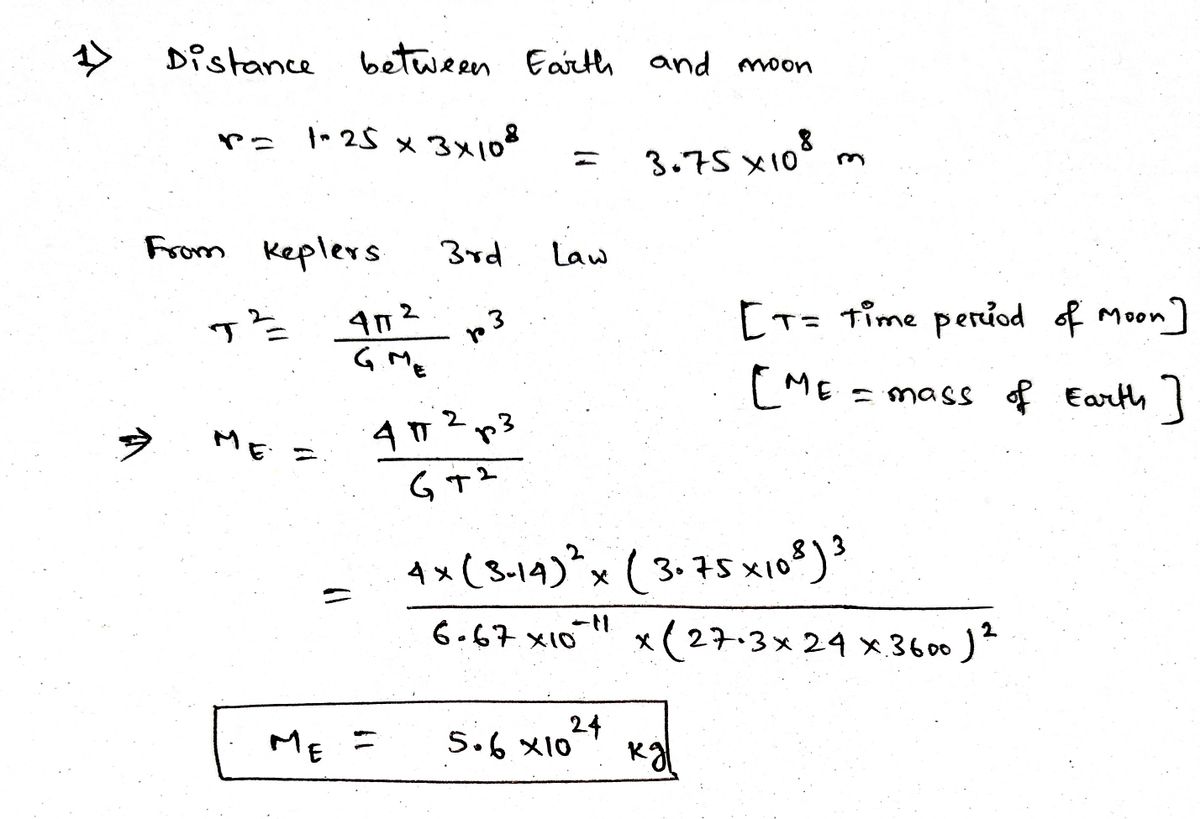
It takes light about 1.25 seconds to reach the moon from Earth. The moon orbits Earth about once every 27.3 days.

Part 1) Estimate the mass of Earth

Part 2) The force of Earth's gravitational pull on the moon is about 2.0 x 10^20 N. Estimate the mass of the moon.

Part 3) The moon has a radius of 1.7 x 10^6 m. On the surface of the moon, what is the acceleration due to the moon's gravity?

Part 4) How many g's is your answer to part c? Or what percentage of Earth's gravity would you experience on the moon?

Part 5) If you stood on the moon and jumped straight up with a velocity of 1.0 m/s, how long would it be before you landed back on the moon's surface?

Please answer all parts.

Please answer in a simply way

**Formulas**

- \(\vec{a}_{\text{avg}} = \frac{\Delta \vec{V}}{\Delta t} \rightarrow \vec{V}_f = \vec{V}_i + \vec{a}_{\text{avg}} \Delta t\)

- \(\vec{V}_{\text{avg}} = \frac{1}{2} (\vec{V}_i + \vec{V}_f)\)

- \(\vec{V}_{\text{avg}} = \frac{\Delta \vec{X}}{\Delta t} \rightarrow \vec{X}_f = \vec{X}_i + \vec{V}_i \Delta t + \frac{1}{2} \vec{a} \Delta t^2\)

- \(V_f^2 = V_i^2 + 2 a \Delta x\)

- \(F = m \vec{a}\)

- \(F_f = \mu_k F_N\)

- \(a_R = \frac{V^2}{r}\)

- \(\vec{F}_{AB} = -\vec{F}_{BA}\)

- \(F_f \leq \mu_s F_N\)

- \(F_G = G \frac{m_1 m_2}{r^2}\)

- \(f = \frac{1}{T}\)

**Constants**

- \(c \approx 3 \times 10^8 \, \text{m/s}\)

- \(g \approx 9.8 \, \text{m/s}^2\)

- \(G \approx 6.7 \times 10^{-11} \, \text{Nm}^2/\text{kg}^2\)

**Geometry**

- \(A = lw\)

- \(A = \frac{1}{2}bh\)

- \(A = \pi r^2\)

- \(c = 2\pi r\)

**Units**

- \(t = s\)

- \(\vec{X} = m\)

- \(\vec{V} = m/s\)

- \(\vec{a} = m/s^2\)

- \(m = \text{kg}\)

- \(\vec{F} = N\)

- \(T = s\)

- \(f = \text{Hz

Expert Solution

Step 1

Step by step

Solved in 2 steps with 2 images

SEE SOLUTION Check out a sample Q&A here

Knowledge Booster

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.

Similar questions