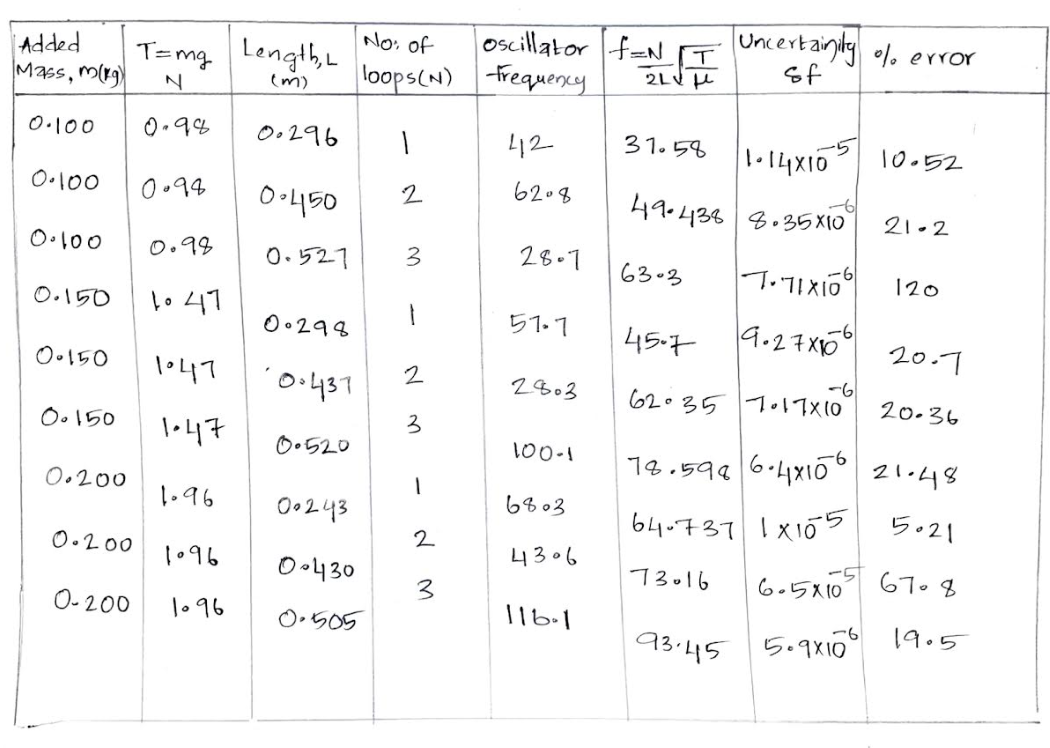
DATA TABLE Calculate the experimental value of the frequency f for each hanging mass and the corresponding uncertainty, 8f. Compare these values to the known oscillator frequency values. Added Tension Length Number Oscillator Experimental Uncertainty % error 8f in string mass fth-fexp L (m) T=mg (N) m (kg) of loops N frequency fth (Hz) Frequency fexp (Hz) N 24Vu f=; fth x 100
DATA TABLE Calculate the experimental value of the frequency f for each hanging mass and the corresponding uncertainty, 8f. Compare these values to the known oscillator frequency values. Added Tension Length Number Oscillator Experimental Uncertainty % error 8f in string mass fth-fexp L (m) T=mg (N) m (kg) of loops N frequency fth (Hz) Frequency fexp (Hz) N 24Vu f=; fth x 100
Related questions
Question
Hi I need help finding the uncertainty in u=6u=u[6L/L+6M/M]
And help with the data table(just show work and explanation for the 1st row)

Transcribed Image Text:SMC/Physics 23/Frequency of transverse standing waves lah ff
DATA TABLE
Calculate the experimental value of the frequency f for each hanging mass and the
corresponding uncertainty. 8f. Compare these values to the known oscillator frequency values.
% error
Uncertainty
fth - fexp
fth
x 100
Added Tension
in string
mass
m
T= mg
(kg)
(N)
0.100
0.100
0.100
0.150
0.150
0.150
0.200
0.200
0.200
0.98
0.48
७.१४
Length
L
(m)
0,195
0.456
Sample calculations:
(12)-> (= + √₂)
0,527
0.294
1.96 0.293
1.96 0.430
Number Oscillator Experimental
Frequency
fexp (Hz)
frequency
fth (Hz)
N
2LH
of loops
N
1
~
·1,47
1.41 0.437
1,47 0.510 3
1
3
1
2
2
42,0
62.8
78.1
57.7
78.3
100.1
68.3
43,6
4.46 0.505 3116.1
Name:
3-5
f=
8f
OC

Transcribed Image Text:SMC/Physics 23/Frequency of transverse standing waves lab II
MATERIAL
- electrically-driven oscillator
- Pasco function generator and leads
rod-mounted pulley
PROCEDURE
a) Record the mass per unit length of the string, u and its uncertainty, Su.
b) Set up the experiment (Fig 2) with a total of 100 grams hanging from the end of the string.
c)
Turn on the oscillator. Move the oscillator assembly toward or away from the pulley
until at least three loops are observed and the amplitude of the oscillation is at a max.
d) Use the meter stick to measure the distance L from the first node away from the
oscillator to the first node away from the pulley. (The loops at the two ends of the string
are not included because the end attached to the oscillator is not a node due to the
vibration, and the position of the node at the pulley end cannot be determined
accurately due to the curvature of the pulley.) Record the number of loops, N in the
distance L, and record L and the uncertainty 8L. This uncertainty should reflect the fact
that the positions of the nodes are difficult to observe.
e) Change the frequency using the dial until another standing wave (with a different N) is
achieved. Record the values. Change the frequency again and find a new N.
DATA and CLACULATIONS
f) Change the mass hanging from the end of the string to 150 grams and repeat parts d and
e. Change to 200 grams and repeat parts d and e.
Mass of string = M =
2,1
Length of string=l= 146
grams=
Mass density of string = μ =
Uncertainty in = dl=
cm =
・1194,60-3
O.S.
_cm
Name:
weight hanger
3 x 50-gram slotted weights
- string, 2.5 meters long
meter stick
-
0.007118
1.46
_kg/m
0,1
Uncertainty in M = 8 M =
SL SM
Uncertainty in μ = δμ = μ
+
L M
Uncertainty in L = 8L = 6.1 cm
Uncertainty in the mass attached = 8m = +0.2grams
Uncertainty in the oscillator frequency = df =
g
3-4
kilograms
meters
|H₂
Expert Solution
Step 1
Step by step
Solved in 6 steps with 6 images
