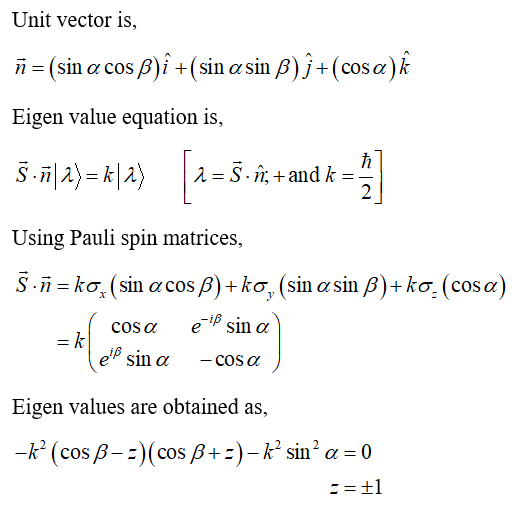
Problem 4. Construct the ket |S· în; +) such that S.âî|S · î; +) = (h/2)|S · în; +), (1) where în is a unit vector with polar angle B and azimuthal angle a, and S is the vector spin operator. Express your answer as a linear combination of |+) and |-). Hint: Treat this as an eigenvalue problem.
Problem 4. Construct the ket |S· în; +) such that S.âî|S · î; +) = (h/2)|S · în; +), (1) where în is a unit vector with polar angle B and azimuthal angle a, and S is the vector spin operator. Express your answer as a linear combination of |+) and |-). Hint: Treat this as an eigenvalue problem.
Related questions
Question
It's a

Transcribed Image Text:Problem 4. Construct the ket |S· în; +) such that
S.âî|S · î; +) = (h/2)|S · în; +),
(1)
where în is a unit vector with polar angle B and azimuthal angle a, and S is the vector spin operator.
Express your answer as a linear combination of |+) and |-). Hint: Treat this as an eigenvalue
problem.
Expert Solution
Step 1
Trending now
This is a popular solution!
Step by step
Solved in 2 steps with 2 images
