Answered: Calculate the uncertainties dr = V(r2)… | bartleby

Related questions

Question

Calculate the uncertainties dr = V(r2) and dp = Vp?) for
a particle confined in the region -a < x < a. The wave function of the
particle is:
1
= (x)
2a
-a < r < a,
COS
(x) = 0,
I > a, r < -a.

Expert Solution

Step 1

As we can see the given wave function is normalised and in outside region it's zero. Therefore

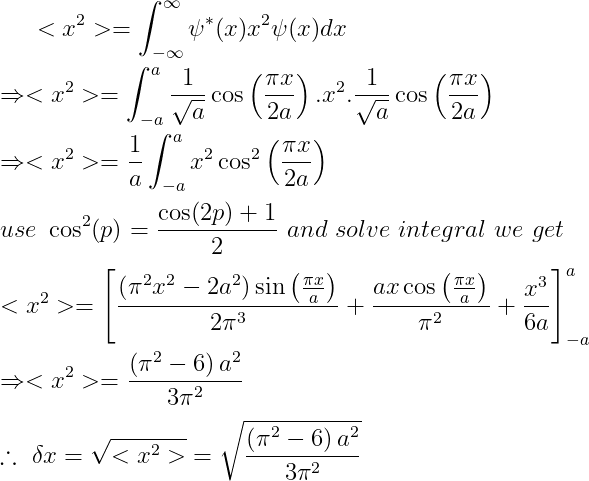
This is the position uncertainty.

Step by step

Solved in 2 steps with 3 images

Blurred answer

Similar questions

6QM Please answer question throughly and detailed.
For a quantum particle in a scattering state as it interacts a certain potential, the general expressions for the transmission and reflection coefficients are given by T = Jtrans Jinc R = | Jref Jinc (1) where Jinc, Jref, Jtrans are probability currents corresponding to the incident, reflected, and transmitted plane waves, respectively. (a). potential For the particle incident from the left to the symmetric finite square well -Vo; a < x < a, V(x) = 0 ; elsewhere, show that B Ꭲ ; R = A A
A particle with mass m is in the state mx +iat 2h V (x, t) = Ae where A and a are positive real constants. Calculate the expectation value of (p).
The wave function W(x,t)=Ax^4 where A is a constant. If the particle in the box W is normalized. W(x)=Ax^4 (A x squared), for 0<=x<=1, and W(x) = 0 anywhere. A is a constant. Calculate the probability of getting a particle for the range x1 = 0 to x2 = 1/3 a. 1 × 10^-5 b. 2 × 10^-5 c. 3 × 10^-5 d. 4 × 10^-5
An electron with an initial kinetic energy of 1.542 eV (in a region with 1.095 eV potential energy) is incident on a potential step (extending from x=0 to ∞) to V=2.381 eV. What is the transmission probability (in %)? FYI: If we had a travelling wave arriving at a similar potential DROP, then k1 (for x<0) would be real and the symmetry of R=(k1-k2)2/(k1+k2)2 implies reflection/transmission are the same as a potential RISE with the same energies but k1 and k2 swapped.
A particle with mass m is in the state .2 mx +iat 2h Y(x,t) = Ae where A and a are positive real constants. Calculate the expectation values of (x).
Consider the wavefunction Y(x) = exp(-2a|x|). a) Normalize the above wavefunction. b) Sketch the probability density of the above wavefunction. c) What is the probability of finding the particle in the range 0 < x s 1/a ?
A particle is in a three-dimensional cubical box that has side length L. For the state nX = 3, nY = 2, and nZ = 1, for what planes (in addition to the walls of the box) is the probability distribution function zero?
A particle with the velocity v and the probability current density J is incident from the left on a potential step of height Uo, that is, U (x) = Uo at r > 0 and U(x) = 0 at r 0.