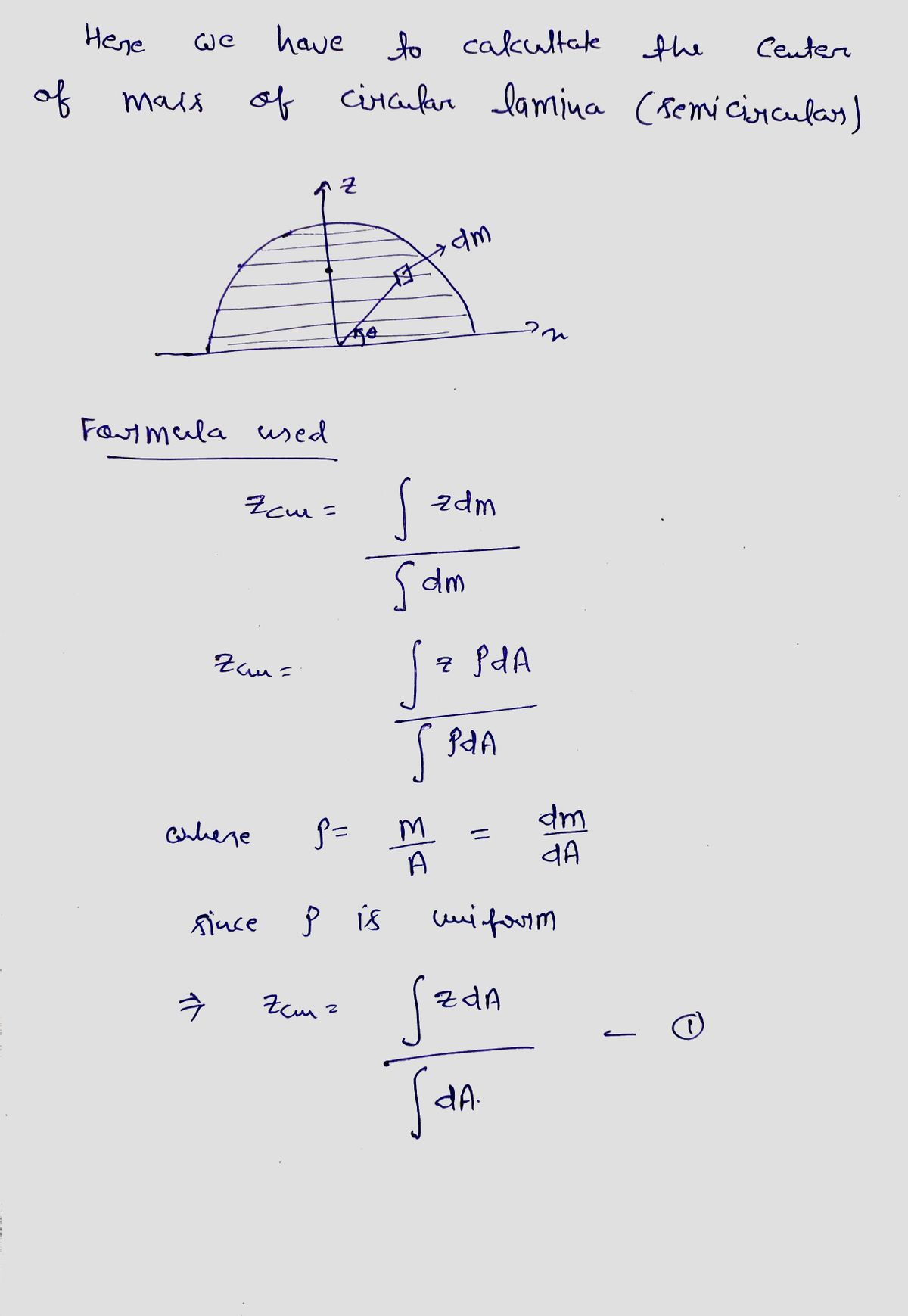
Bodies: Planar Motion Semicircle To find the center of mass of a thin wire bent into the form of a semicircle of radius a, we use axes as shown in Figure 8.1.2. We have dl = a do dl x Zcm za sin 0 Spla sina de pado 7cm 2a T 4a 3π (8.1.11) (8.1.12) Semicircular Lamina In the case of a uniform semicircular lamina, the center of mass is on the z-axis (Figure 8.1.2). As an exercise, the student should verify that (8.1.13) (8.1.14)
Bodies: Planar Motion Semicircle To find the center of mass of a thin wire bent into the form of a semicircle of radius a, we use axes as shown in Figure 8.1.2. We have dl = a do dl x Zcm za sin 0 Spla sina de pado 7cm 2a T 4a 3π (8.1.11) (8.1.12) Semicircular Lamina In the case of a uniform semicircular lamina, the center of mass is on the z-axis (Figure 8.1.2). As an exercise, the student should verify that (8.1.13) (8.1.14)
Related questions
Question
100%
calculate the center of mass of semicircular lamina using expression (prove 8.1.14)

Transcribed Image Text:Bodies: Planar Motion
0
Semicircle
To find the center of mass of a thin wire bent into the form of a semicircle of radius a, we
use axes as shown in Figure 8.1.2. We have
dl = a do
dl
X
7cm
z = a sin 0
p(a sin a do
Spade
7cm =
2a
T
4a
3π
(8.1.11)
(8.1.12)
Semicircular Lamina
In the case of a uniform semicircular lamina, the center of mass is on the z-axis
(Figure 8.1.2). As an exercise, the student should verify that
(8.1.13)
(8.1.14)
Expert Solution
Step 1
Step by step
Solved in 2 steps with 2 images
