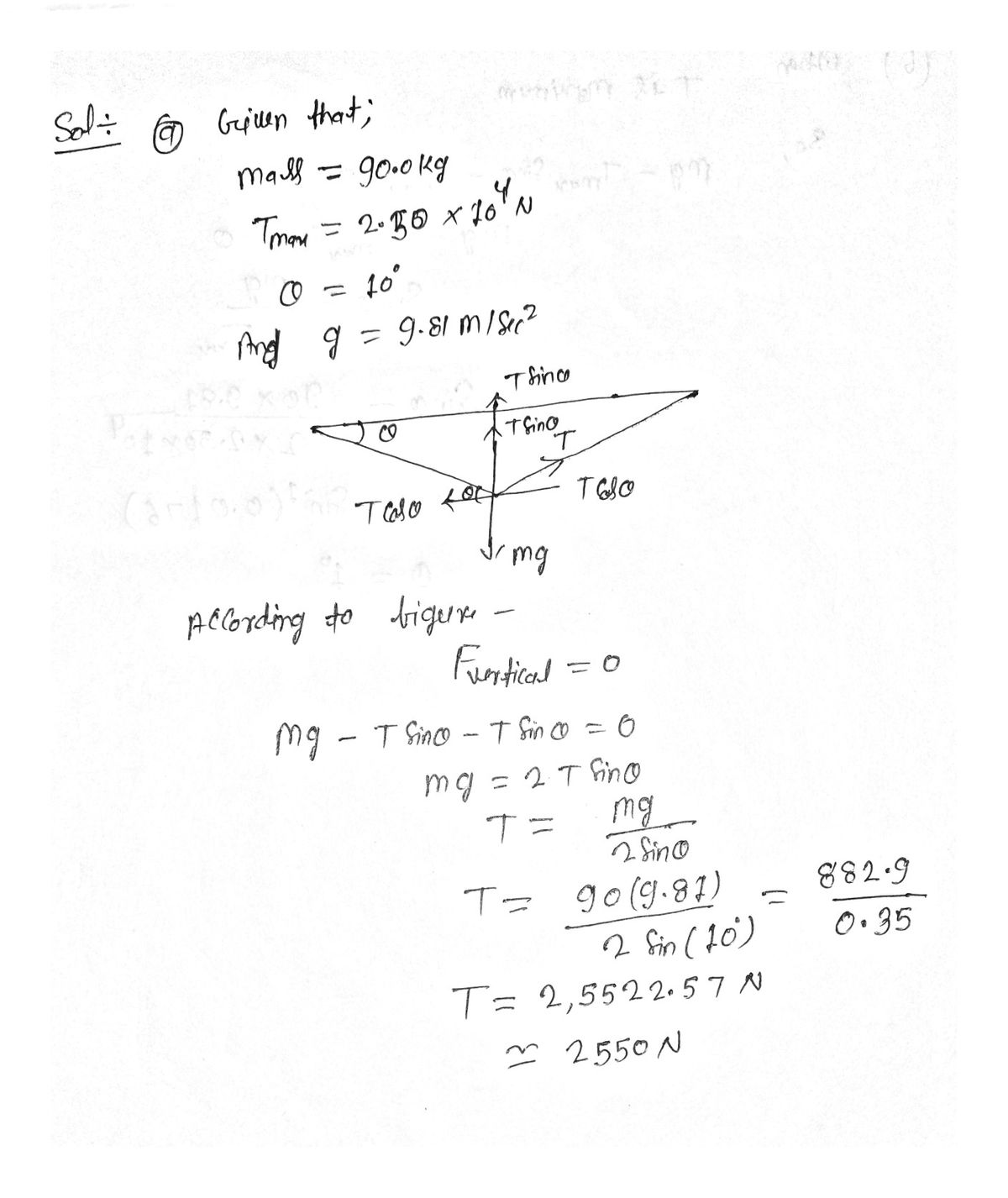
An adventurous archaeologist cros- ses between two rock cliffs by slowly going hand over hand along a rope stretched between the cliffs. He stops to rest at the middle of the rope (Figure 5.43). The rope will break if the tension in it exceeds 2.50 × 104 N. Our hero's mass is 90.0 kg. (a) If the angle 0 is 10.0º, find the tension in the rope. Start with a free-body diagram of the archaeologist. (b) What is the smallest value the angle 8 can have if 0 the rope is not to break?

An adventurous archaeologist cros- ses between two rock cliffs by slowly going hand over hand along a rope stretched between the cliffs. He stops to rest at the middle of the rope (Figure 5.43). The rope will break if the tension in it exceeds 2.50 × 104 N. Our hero's mass is 90.0 kg. (a) If the angle 0 is 10.0º, find the tension in the rope. Start with a free-body diagram of the archaeologist. (b) What is the smallest value the angle 8 can have if 0 the rope is not to break?

Chemistry

10th Edition

ISBN:9781305957404

Author:Steven S. Zumdahl, Susan A. Zumdahl, Donald J. DeCoste

Publisher:Steven S. Zumdahl, Susan A. Zumdahl, Donald J. DeCoste

Chapter1: Chemical Foundations

Section: Chapter Questions

Problem 1RQ: Define and explain the differences between the following terms. a. law and theory b. theory and...

See similar textbooks

Similar questions

How does the measure of the relative viscosity provide a measure of the transesterification process? Select one: The produced biodiesel has a much higher viscosity than the starting soybean oil. The produced glycerol binds with the produced FAMES, creating more viscous products. O As relative viscosity decreases, this indicates that the reaction is progressing as desired. The produced biodiesel has a much lower viscosity than the starting soybean oil.
(a) Would you expect the viscosity of n-pentane,CH3CH2CH2CH2CH3, to be larger or smaller than the viscosityof n-hexane, CH3CH2CH2CH2CH2CH3? (b) Wouldyou expect the viscosity of neopentane, (CH3)4C, to besmaller or larger than the viscosity of n-pentane? (See Figure11.6 to see the shapes of these molecules.)
Nitrogen is heated with oxygen to form dinitrogen pentaoxide gas. Express your answer as a chemical equation. Identify all of the phases in your answer. 0 ΑΣΦ P ? ti dong not occur for this question.
Which type of intermolecular interaction is shown below? ion-ion H-bond ion-dipole H dipole-dipole +N H induced dipole/dispersion H |||H
Write balanced net ionic equation for Na3PO4(aq)+NiCl2(aq)→Ni3(PO4)2(s)+NaCl(aq). Express your answer as a chemical equation. Identify all of the phases in your answer.
Balance each chemical equation. CO2(g)+CaSiO3(s)+H2O(l)→SiO2(s)+Ca(HCO3)2(aq) Express your answer as a chemical equation. Identify all of the phases in your answer.
Zn(s)+HNO3(aq)⟶Zn(NO3)2(aq)+H2(g) Express your answer as a chemical equation. Identify all of the phases in your answer. Keep the order of compounds as in the original equation. Al(s)+H2SO4(aq)⟶Al2(SO4)3(aq)+H2(g) Express your answer as a chemical equation. Identify all of the phases in your answer. Keep the order of compounds as in the original equation.
Human cells obtain energy from a reaction called cellular respiration. Balance the skeletal equation for cellular respiration. C6H12O6(aq)+ O2(g)= CO2(g) +H2O(I) express your answer as a chemical equation. Identify all of the phases in your
In your own words, describe why metals are such good conductors of electricity. Be sure to include the definition of metallic bond in your answer. Answer in 2 to 3 complete sentences. I !!! H Normal A Enter your answer here BIUS √x 20 Tx
7The substance with the lowest known boiling point (4 K) is helium, an atomic element that has two electrons. Hydrogen is a diatomic molecule and also has two electrons, but its boiling point is significantly higher, at 20.28 K. (a) What is the dominant intermolecular force between a pair of helium atoms and a pair of H2 molecules? (b) Why do you think H2 has a higher boiling point?
4. An alloy has an elastic modulus of 115 GPa. For the alloy, plastic deformation begins at a stress of 275 MPa. (a) If a sample of cross-sectional area 325 mm? is obtained from the alloy, determine the maximum load that may be applied on the sample without plastic deformation. (b) The original sample length is specified as 115 mm, what is the maximum length to which the sample can be stretched without plastic deformation.
Au2O(s)+C(s)→Au(s)+CO(g)Au2O(s)+C(s)→Au(s)+CO(g) Express your answer as a chemical equation. Identify all of the phases in your answer. Balance each chemical equation.